How to use L'Hopital's Rule to Find the Limit of ln(ln(x))/x as x approaches Infinity #shorts

preview_player
Показать описание
How to use L'Hopital's Rule to Find the Limit of ln(ln(x))/x as x approaches Infinity #shorts

If you enjoyed this video please consider liking, sharing, and subscribing.

There are several ways that you can help support my channel:)

************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course

Advanced Calculus Course

Calculus 1 Course

Calculus 2 Course

Calculus 3 Course

Calculus Integration Insanity

Differential Equations Course

College Algebra Course

How to Write Proofs with Sets Course

How to Write Proofs with Functions Course

Statistics with StatCrunch Course

Math Graduate Programs, Applying, Advice, Motivation

Daily Devotionals for Motivation with The Math Sorcerer

Thank you:)
  1. The Math Sorcerer
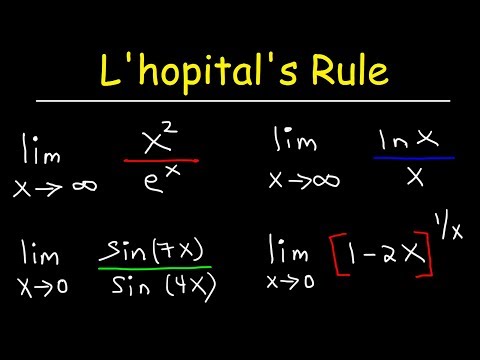
  2. How to use L'Hopital's Rule to Find the Limit of ln(ln(x))/x as x approaches Infinity #shorts
  3. limits lhopitals
  4. rule
Рекомендации по теме
L'hopital's rule 0:13:09

L'hopital's rule

Understanding Limits and 0:09:12

Understanding Limits and L'Hospital's Rule

L’Hopitals Rule 0:00:32

L’Hopitals Rule

US presidents explain 0:01:25

US presidents explain L'Hôpital's rule in under 90 seconds

Limits, L'Hôpital's rule, 0:18:27

Limits, L'Hôpital's rule, and epsilon delta definitions | Chapter 7, Essence of calculus

Simplified L’Hopitals Rule 0:07:23

Simplified L’Hopitals Rule | All You Need To Know About L’Hospitals Rule

Introduction to l'Hôpital's 0:08:52

Introduction to l'Hôpital's rule | Derivative applications | Differential Calculus | Khan ...

L'Hospital's Rule, Derivative, 0:00:37

L'Hospital's Rule, Derivative, Limits, Example - Calculus

Calculus-I : Finalde 0:09:33

Calculus-I : Finalde Çıkabilecek Sorular-15 (İngilizce)

How to apply 0:01:37

How to apply L'Hopital's Rule to evaluate the limit

L’Hospital’s Rule (2 0:02:13

L’Hospital’s Rule (2 Minutes)

But Why Does 0:15:55

But Why Does L'Hopital's Rule Work?

'Proof' of L'Hospital's 0:10:44

'Proof' of L'Hospital's Rule

easiest method to. 0:00:49

easiest method to. solve limit by L Hospital rule #maths #limit #subscribe

L'Hôpital's rule | 0:00:49

L'Hôpital's rule | JMDI DEFENCE ACADEMY | NDA 2023 | NDA foundation

l hospital rule 0:00:16

l hospital rule nda || l hospital || short trick @qutubsir

L'Hopital's Rule for 0:09:20

L'Hopital's Rule for the 0/0 Indeterminate Form (proof)

Calculus 6.08g - 0:13:34

Calculus 6.08g - An Intuitive Proof of l`Hopital`s Rule

Did L'Hospital Steal 0:00:59

Did L'Hospital Steal his Rule?

so you want 0:07:02

so you want to use L'Hospital's Rule?

How to Use 0:00:47

How to Use L'Hôpital's Rule #shorts

CALCULUS - I 0:10:40

CALCULUS - I LIMITS using L' Hopitals Rule

Limits requiring L'Hopital's 0:16:35

Limits requiring L'Hopital's Rule

Limit of a 0:06:32

Limit of a function | L'Hopital's rule

Комментарии
Автор

I immediately knew. Because lnx<x for all x>1, we can immediately recognize that ln(lnx) is a smaller infinity. Hence, the limit approaches zero.

jacobstarr
Автор

Well if you studied things about complexity and the big O notation, you probably know that O(ln n) is already way better than O(n), and O(ln ln n) is even better, so this limit is easily 0 by knowing that ln(ln x) is having a speed of increase way slower than x

not_vinkami
Автор

What happened if we have the same problem but x goes to 0 help please

danielcepedavillarraga
Автор

what the faaak, that went a bit to fast mate

hansjacobthordalsloth