filmov
tv
'Proof' of L'Hospital's Rule

Показать описание
In this video I showed a simplified 'proof' of L'Hoc pital's Rule using the definition of the derivative.
Calculus 6.08g - An Intuitive Proof of l`Hopital`s Rule
'Proof' of L'Hospital's Rule
L'Hopital's Rule for the 0/0 Indeterminate Form (proof)
Proof of special case of l'Hôpital's rule | Differential Calculus | Khan Academy
L'hopital's rule
General case proof of L'Hôpital's rule Part 2: infinity/infinity form (Requires Real analy...
Limits, L'Hôpital's rule, and epsilon delta definitions | Chapter 7, Essence of calculus
Proof of L'Hôpital's rule
Real Analysis 42 | L'Hospital's Rule
Understanding Limits and L'Hospital's Rule
Hopital rule proof
L'Hospital's Rule - Heuristic Proof
Did L'Hospital Steal his Rule?
Partial Proof of L'Hopital's Rule (Only Form 0/0)
Calculus AB/BC – 4.7 Using L'Hopital's Rule for Determining Limits of Indeterminate Forms...
L'Hospital's Rule - A general Proof
Real Analysis | The Generalized Mean Value Theorem and One part of L'Hospital's rule.
General case proof of L'Hôpital's rule ( l'hospital ) Part 1: 0/0 form (Requires Real...
Special case proof of L'Hôpital's (l'hospital) rule Part 2: infinity/infinity form
Tricky L'Hopital's Rule problem | Derivative applications | Differential Calculus | Khan A...
L Hospital Rule Proof and Example | L Hospital's Rule in Limits
L'Hopital's Rule ultimate study guide
Proof of l’Hopital Simple Case
L' Hopital's Rule | Proof | Phyomathix
Комментарии
 0:13:34
0:13:34
 0:10:44
0:10:44
 0:09:20
0:09:20
 0:05:21
0:05:21
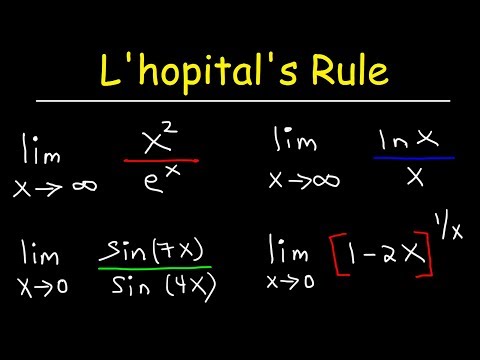 0:13:09
0:13:09
 0:05:54
0:05:54
 0:18:27
0:18:27
 0:23:54
0:23:54
 0:11:17
0:11:17
 0:09:12
0:09:12
 0:34:42
0:34:42
 0:07:41
0:07:41
 0:00:59
0:00:59
 0:04:07
0:04:07
 0:10:09
0:10:09
 0:18:04
0:18:04
 0:11:46
0:11:46
 0:10:05
0:10:05
 0:04:24
0:04:24
 0:13:10
0:13:10
 0:01:51
0:01:51
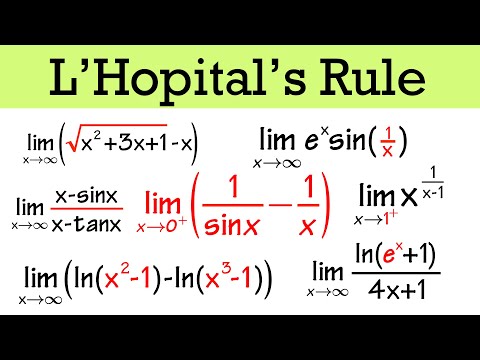 0:44:56
0:44:56
 0:09:00
0:09:00
 0:06:59
0:06:59