filmov
tv
L'Hospital's Rule, Derivative, Limits, Example - Calculus

Показать описание
This video covers a worked example on L'Hospital's rule, which is another contextual application of the derivative to evaluate the limits of functions with indeterminate limits.
L'hopital's rule
L'Hospital's Rule, Derivative, Limits, Example - Calculus
Limits, L'Hôpital's rule, and epsilon delta definitions | Chapter 7, Essence of calculus
Introduction to l'Hôpital's rule | Derivative applications | Differential Calculus | Khan ...
4.7g L'Hospital's Rule, Derivative, Limits, Example - AP Calculus BC
4.7e L'Hospital's Rule, Derivative, Limits, Example - AP Calculus BC
L'Hôpital's rule example 3 | Derivative applications | Differential Calculus | Khan Academ...
4.7c L'Hospital's Rule, Derivative, Limits, Example - AP Calculus BC
Limit Practice Example (L’Hospital’s Rule)
L'Hôpital's rule example 1 | Derivative applications | Differential Calculus | Khan Academ...
L'Hospital's Rule - Limits Examples
4.7d L'Hospital's Rule, Derivative, Limits, Example - AP Calculus BC
Understanding Limits and L'Hospital's Rule
L'Hôpital's rule example 2 | Derivative applications | Differential Calculus | Khan Academ...
6.7 L'Hôpital's Rule: Examples
How to apply L'Hopital's Rule to evaluate the limit
Did L'Hospital Steal his Rule?
Limits requiring L'Hopital's Rule
Limits | L'Hospital's Rule: Proof and 2 Examples
Tricky L'Hopital's Rule problem | Derivative applications | Differential Calculus | Khan A...
Proof of special case of l'Hôpital's rule | Differential Calculus | Khan Academy
Calculus 1: L'Hospital's Rule (2 of 25) Example 1
L'Hopital's Rule ultimate study guide
Calculus 2 Lecture 6.7: Evaluating Limits of Indeterminate Forms
Комментарии
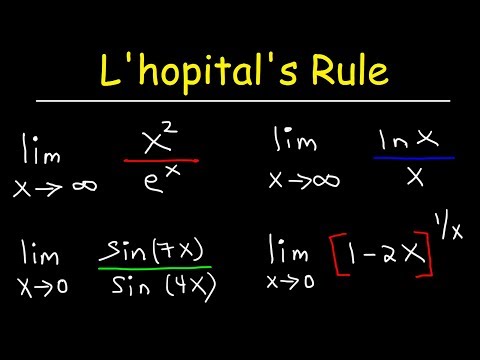 0:13:09
0:13:09
 0:00:37
0:00:37
 0:18:27
0:18:27
 0:08:52
0:08:52
 0:01:00
0:01:00
 0:00:41
0:00:41
 0:07:50
0:07:50
 0:00:22
0:00:22
 0:02:49
0:02:49
 0:07:43
0:07:43
 0:13:54
0:13:54
 0:00:29
0:00:29
 0:09:12
0:09:12
 0:05:15
0:05:15
 0:05:24
0:05:24
 0:01:37
0:01:37
 0:00:59
0:00:59
 0:16:35
0:16:35
 0:11:27
0:11:27
 0:13:10
0:13:10
 0:05:21
0:05:21
 0:03:07
0:03:07
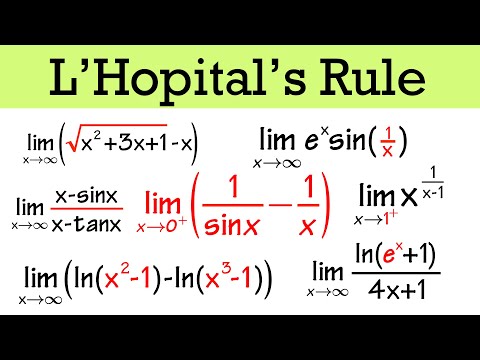 0:44:56
0:44:56
 1:40:42
1:40:42