filmov
tv
Partial fractions decomposition with a cubic denominator (root guessing method).

Показать описание
We compute the integral of (3x^2+5x-2)/(x^3-7x^2+7x+15) by using partial fractions decomposition. The denominator is a cubic polynomial, and it is not easily factorable, so we resort to guessing a root of the cubic polynomial and using the factor theorem.
The idea of the factor theorem is that every root of a polynomial corresponds to a linear factor, so if we can guess a root, then we automatically know a factor of the polynomial. We find a root at x=-1, which means the polynomial has a factor of x+1.
Now we use polynomial long division to divide out the factor of x+1 from the denominator. This leaves us with a quadratic polynomial that is quickly factored into two additional linear factors.
Now that the denominator is factored into three linear factors, we make our partial fractions proposal for the decomposition of the integrand into three terms: each a rational expression with a single linear factor in the denominator.
We clear all the denominators and use the partial fractions substitution method to determine the partial fractions coefficients.
Finally, we use the partial fractions decomposition to re-express the integral as a sum of three terms that all integrate to natural log terms, and we're done!
The idea of the factor theorem is that every root of a polynomial corresponds to a linear factor, so if we can guess a root, then we automatically know a factor of the polynomial. We find a root at x=-1, which means the polynomial has a factor of x+1.
Now we use polynomial long division to divide out the factor of x+1 from the denominator. This leaves us with a quadratic polynomial that is quickly factored into two additional linear factors.
Now that the denominator is factored into three linear factors, we make our partial fractions proposal for the decomposition of the integrand into three terms: each a rational expression with a single linear factor in the denominator.
We clear all the denominators and use the partial fractions substitution method to determine the partial fractions coefficients.
Finally, we use the partial fractions decomposition to re-express the integral as a sum of three terms that all integrate to natural log terms, and we're done!
 0:14:12
0:14:12
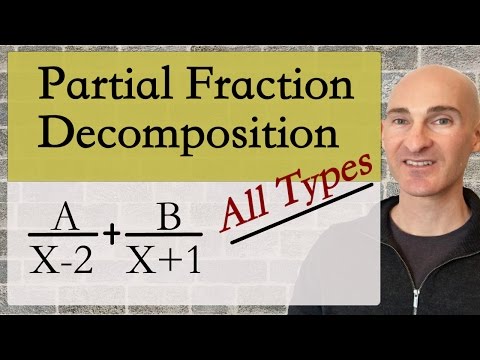 0:32:24
0:32:24
 0:09:08
0:09:08
 0:11:53
0:11:53
 0:08:08
0:08:08
 0:41:07
0:41:07
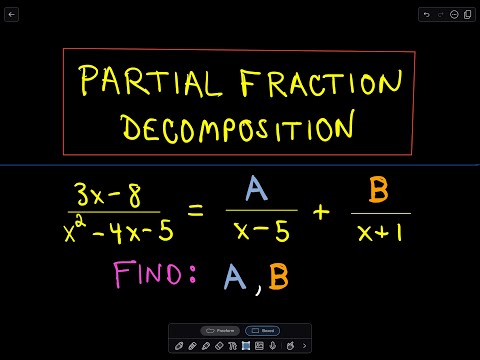 0:06:22
0:06:22
 0:08:37
0:08:37
 0:27:27
0:27:27
 0:06:08
0:06:08
 0:05:48
0:05:48
 0:11:02
0:11:02
 0:09:01
0:09:01
 0:13:28
0:13:28
 0:04:32
0:04:32
 0:07:19
0:07:19
 0:33:10
0:33:10
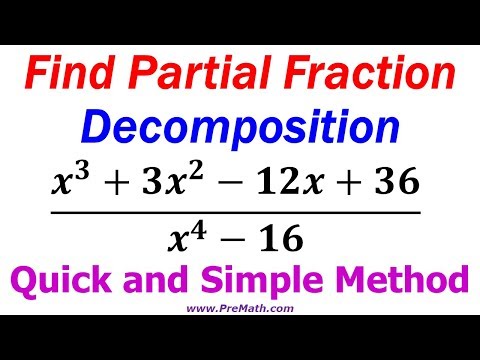 0:15:54
0:15:54
 0:08:54
0:08:54
 0:14:30
0:14:30
 0:06:27
0:06:27
 0:32:47
0:32:47
 0:12:36
0:12:36
 2:55:43
2:55:43