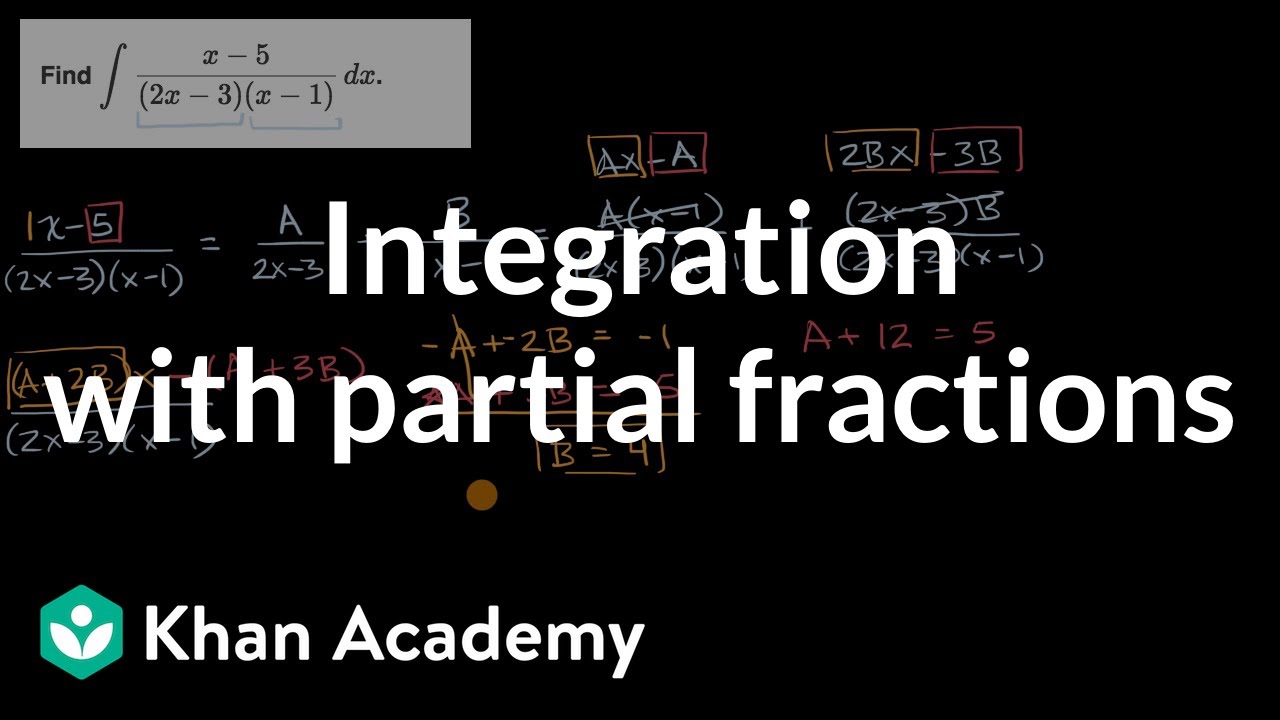
Integration with partial fractions | AP Calculus BC | Khan Academy

Finding the integral of a rational function using linear partial fraction decomposition.

Khan Academy is a nonprofit organization with the mission of providing a free, world-class education for anyone, anywhere. We offer quizzes, questions, instructional videos, and articles on a range of academic subjects, including math, biology, chemistry, physics, history, economics, finance, grammar, preschool learning, and more. We provide teachers with tools and data so they can help their students develop the skills, habits, and mindsets for success in school and beyond. Khan Academy has been translated into dozens of languages, and 15 million people around the globe learn on Khan Academy every month. As a 501(c)(3) nonprofit organization, we would love your help! Donate or volunteer today!

[omkarsawant]

Maths is beautiful when we have teachers like you👍👍

[Salamanca-joro]

I solved it the same way !! Great ! Now i can move forward to the next topic

[michalchik]

There's a much easier way to do this. Since the constants A&B have to be true for all values of x. And since the denominators are the same on both sides, just look at the numerators and set them as an equation.

X - 5= a(x -1) + b(2x-3)

First set x = 1, turning x -1 into 0, so the equation becomes

1-5=b(2*1-3)
Simplifying b equals 4. You can do the same thing, by picking x equals 3/2, cancelling out the b part of the equation, and solving for a

[edwardrk]

thankyou bro, I understand partial fraction now !!

[rafi_xoss]

I loved it, helped me a lot. Take love from your own Bangladesh.

[hotnika]

I solved by taking out the 1/2 from 1/2x-3 and solved -7/2*ln|x-3/2| out but seems that it is different with the result of -7/2*ln|2x-3| when substituting numbers into them, am I wrong?