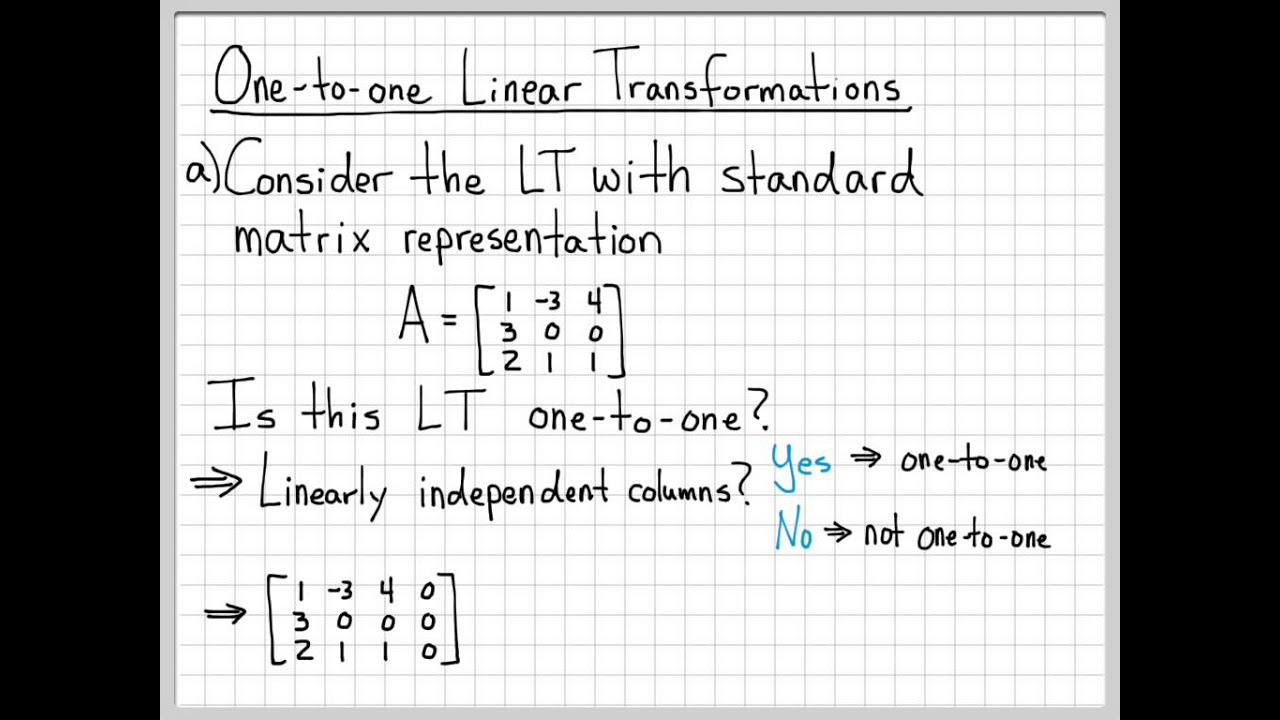
Linear Algebra Example Problems - One-to-One Linear Transformations

A one-to-one linear transformation maps each point in its domain to a unique point in its range.

When a linear transformation is described in term of a matrix it is easy to determine if the linear transformation is one-to-one or not by checking the linear dependence of the columns of the matrix. If the columns are linearly independent, the linear transformation is one-to-one. If the columns are dependent, then the linear transformation is not one-to-one.

This video works two different examples. One linear transformation is found to be one-to-one while the other linear transformation is NOT one-to-one.

[advnturetime]

Way better than Khan Academy's endless scribbling and repetition.

[FunOrange]

Incredibly clear and concise. I'm glad you didn't waste our time by writing everything out ahead of time and skipping row reduction.

[parassharma]

I can't thank you enough. YOU ARE A LEGEND. I watched & learned from all of your previous linear algebra videos in a single sitting. THANK YOU SO MUCH SIR!

[oogabooga]

just came back from your other video on the onto linear transformation and i didn't get what is the difference between them

[mazinabdelrahman]

You just explained what i spent days on in 4 minutes. Thank you soooo much

[MKSurvant]

Thank you so much! This never made sense for me before. Even Khan Academy confused me for once. THANK

[ryandavis]

Very concise, clear and competent explanation. Had to find a 3rd "c-word" there...not sure how this only has 5xx views...one of the best videos I've seen on the topic. Thank you...this will help me on my final...which is in 10 minutes!

[che-hsiensu]

Nice Video, Thanks a lot! Tmr is my linear algebra final, wish me luck!

[antoniomariasantosdacunha]

Excuse me if I'm saying something stupid, but isn't that more work than necessary? Following the hipothesis that in order for the rows of a matrix to be linearly independent, the augmented matrix has only trivial solutions, can't you verify that just by calculating the determinant? Isn't that way easier?

[MostafaAbdelrehim]

Thanks a lot Adam for the amazing video! I just curious to know what software you are using to imitate handwriting on whiteboard? Thanks

[muhammadseyab]

If A is sqaure matrix can we check this using determinant if its non zero then one to one....?

[toko]

2:18 How is that matrix independent. It only has 3 pivot columns so the last variable is a free parameter meaning you have infinite number of solutions. Thus its dependent and therefore not one-to one ??

[iced]

Quick question: If I have a 4x4 matrix and X1=0, X2=0, X3=, but X4 can equal a free variable, does that mean it's one-to-one. Like imagine a 4x4 matrix but the last row and column consist of all zeros, leaving X4 equal to a free variable or could I just set it equal to 0. This would mean the LT is one-to-one right?

[syedjunaidkhalid]

So in your both videos, you had the same matrix and in both you ended up saying linear independent. Which means both are one to one and onto at the same time?

[siderminerkgl]

why do you put the symbol of " if " => ? Wouldn't be right to put the " if and only if " <=> ?

[sportsgamingcubing]

Awesome video super helpful and concise. Thanks so much!

[PedroGomes-huhw]

Thank you for your explanations. Simple and clear :)

[nobodyandirine]

so LI is onto and LD is one to one or vice versa ? got a test tomorrow and my prof said one to one is infin solution and onto always has a pivot(LI)

[kknmk]

Very clear explanations and examples. Thanks!

[EmapMe]

Why is a transformation one to one if the columns are linearly independent? Isn't that the exact same thing you do to check whether a function is onto?