filmov
tv
The Real Reason Complex Numbers are Useful!

Показать описание
Have you ever wondered why complex numbers are so effective? It's because of geometry!
00:00 Intro
02:06 How can numbers have "phase"?
05:19 Deriving the complex numbers
13:02 What about Euler's Formula?
14:18 Application: Fourier series
16:09 Closing remarks
This is a submission for 3Blue1Brown's 2nd Summer of Math Exposition contest (#SoME2).
00:00 Intro
02:06 How can numbers have "phase"?
05:19 Deriving the complex numbers
13:02 What about Euler's Formula?
14:18 Application: Fourier series
16:09 Closing remarks
This is a submission for 3Blue1Brown's 2nd Summer of Math Exposition contest (#SoME2).
The Real Reason Complex Numbers are Useful!
How Imaginary Numbers Were Invented
The true history of complex numbers.
The Real World Uses of Imaginary Numbers
Imaginary Numbers Are Real [Part 1: Introduction]
Necessity of complex numbers
Imaginary Numbers Are Just Regular Numbers
Do Complex Numbers Exist?
Imaginary Numbers Are Not Imaginary | Jeff O'Connell | TEDxOhloneCollege
The beauty of complex numbers
Complex number fundamentals | Ep. 3 Lockdown live math
Complex Numbers Have More Uses Than You Think
How Imaginary Numbers Make Real Physics Easier to Understand
10 - What are Imaginary Numbers?
5 simple unsolvable equations
What are imaginary numbers?
How do complex numbers actually apply to control systems?
Imaginary Numbers Are Real [Part 5: Numbers are Two Dimensional]
Why Complex Multiplication Produces Rotation
Imaginary Numbers Are Real [Part 2: A Little History]
Complex Numbers in Quantum Mechanics
Why Complex Numbers as 2X2 Matrices???
Introduction to Complex Numbers (old version)
The geometric view of COMPLEX NUMBERS
Комментарии
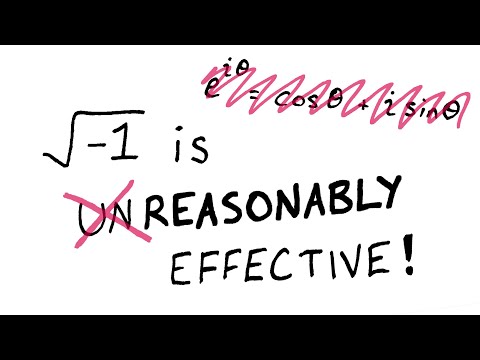 0:17:01
0:17:01
 0:23:29
0:23:29
 0:05:43
0:05:43
 0:16:49
0:16:49
 0:05:47
0:05:47
 0:07:39
0:07:39
 0:09:02
0:09:02
 0:11:26
0:11:26
 0:10:04
0:10:04
 0:13:42
0:13:42
 1:22:11
1:22:11
 0:21:46
0:21:46
 0:16:17
0:16:17
 0:38:00
0:38:00
 0:00:50
0:00:50
 0:03:55
0:03:55
 0:16:43
0:16:43
 0:04:38
0:04:38
 0:05:44
0:05:44
 0:05:16
0:05:16
 0:19:57
0:19:57
 0:11:51
0:11:51
 0:08:18
0:08:18
 0:10:19
0:10:19