filmov
tv
The geometric view of COMPLEX NUMBERS
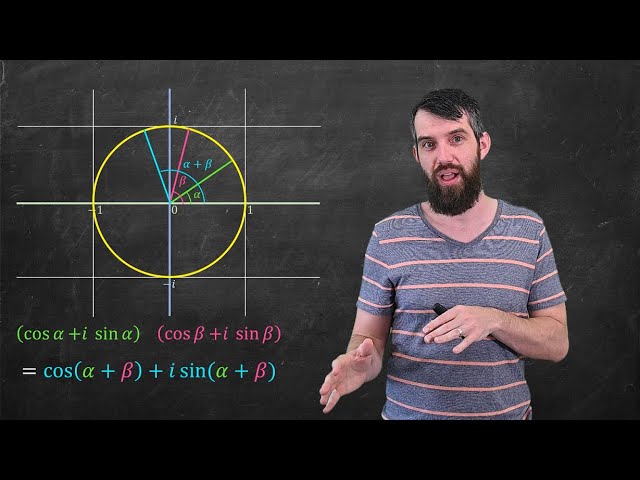
Показать описание
COURSE PLAYLISTS:
OTHER PLAYLISTS:
► Want to learn math effectively? Check out my "Learning Math" Series:
►Want some cool math? Check out my "Cool Math" Series:
BECOME A MEMBER:
SOCIALS:
The geometric view of COMPLEX NUMBERS
Complex numbers: The Geometric View (Exercises)
Imaginary Numbers Are Real [Part 1: Introduction]
The complex geometry of Islamic design - Eric Broug
The 5 ways to visualize complex functions | Essence of complex analysis #3
The geometric notation of complex numbers. #Shorts
Calculus 2: Complex Numbers & Functions (6 of 28) Geometric Representation
1.6.4 Geometric interpretation of complex numbers
Draw all possible Stereo isomers of a complex Ca[Co(NH3)Cl(Ox)2]
Complex Numbers as Points (3 of 4: Geometric Meaning of Multiplication)
Complex number fundamentals | Ep. 3 Lockdown live math
Geometric Transformations on Complex Numbers - Multiplying 2 Complex Numbers
Geometric Transformations of Complex Numbers - Addition
Complex exponents geometric approach
Complex Numbers as Points (2 of 4: Geometric Meaning of Subtraction)
Tensors: A Geometric View
Complex Magnitude Equation with Geometric Interpretation
[CA/Week 1] 2. Geometric interpretation of a complex number.
Geometric effect of multiplying complex numbers
Complex Numbers: Operations, Complex Conjugates, and the Linear Factorization Theorem
Geometric Algebra in 2D - Fundamentals and Another Look at Complex Numbers
Drawing geometric art #drawing😎😎 #shorts #drawing #art #drawingshorts #Zentangle Patterns
Dot products and duality | Chapter 9, Essence of linear algebra
Geometric Representations of sets of complex numbers
Комментарии
 0:10:19
0:10:19
 0:28:57
0:28:57
 0:05:47
0:05:47
 0:05:07
0:05:07
 0:14:32
0:14:32
 0:01:01
0:01:01
 0:02:22
0:02:22
 0:08:43
0:08:43
 0:13:37
0:13:37
 0:06:50
0:06:50
 1:22:11
1:22:11
 0:01:47
0:01:47
 0:06:11
0:06:11
 0:11:42
0:11:42
 0:07:42
0:07:42
 0:56:32
0:56:32
 0:08:20
0:08:20
![[CA/Week 1] 2.](https://i.ytimg.com/vi/AhXfUBU79ro/hqdefault.jpg) 0:04:44
0:04:44
 0:05:54
0:05:54
 0:08:35
0:08:35
 0:35:14
0:35:14
 0:00:37
0:00:37
 0:14:12
0:14:12
 0:14:49
0:14:49