filmov
tv
Number Theory | Inverses modulo n

Показать описание
We give a characterization of numbers which are invertible modulo n.
Number Theory | Inverses modulo n
How To Find The Inverse of a Number ( mod n ) - Inverses of Modular Arithmetic - Example
Extended Euclidean Algorithm and Inverse Modulo Tutorial
Multiplicative Inverse
Finding the Modular Inverse #numbertheory
What does a ≡ b (mod n) mean? Basic Modular Arithmetic, Congruence
Number Theory | Modular Inverses: Example
Multiplicative Inverse Mod n
The inverse of 3 modulo 7 is?
Finding Modular Inverses
Theory of Numbers, Lec.- 29(Inverse of an Integer Modulo m)
Number Theory | Linear Congruence Example 2
Basics of Modular Arithmetic
Solve a Linear Congruence using Euclid's Algorithm
Linear Congruence and Inverse of Modulo
Modulo Operator Examples #Shorts #math #maths #mathematics #computerscience
calculating the inverse of a number in some modulus for example inverse(11) mod 26=?
2.2.3 Inverses mod n: Video
Number Theory (Inverse of Number)
Modular Multiplicative Inverse : Modular Arithmetic for Division | CP Course | EP 61
Modular inverse made easy
Multiplicative inverses mod N
Multiplicative Modulo Inverse - Number Theory Advanced | Lecture 37.2
What is modular inverse ?
Комментарии
 0:08:02
0:08:02
 0:10:58
0:10:58
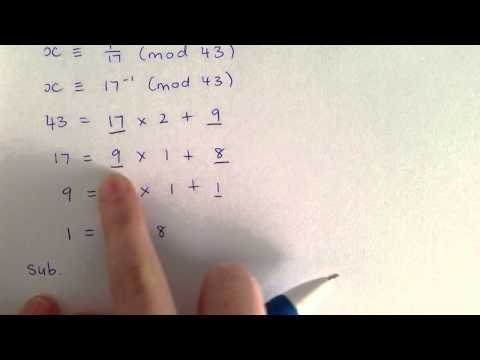 0:06:00
0:06:00
 0:10:03
0:10:03
 0:08:11
0:08:11
 0:05:45
0:05:45
 0:09:41
0:09:41
 0:17:49
0:17:49
 0:01:21
0:01:21
 0:05:01
0:05:01
 0:29:19
0:29:19
 0:04:44
0:04:44
 0:18:39
0:18:39
 0:14:23
0:14:23
 0:04:59
0:04:59
 0:00:30
0:00:30
 0:01:33
0:01:33
 0:04:17
0:04:17
 0:17:05
0:17:05
 0:16:50
0:16:50
 0:04:12
0:04:12
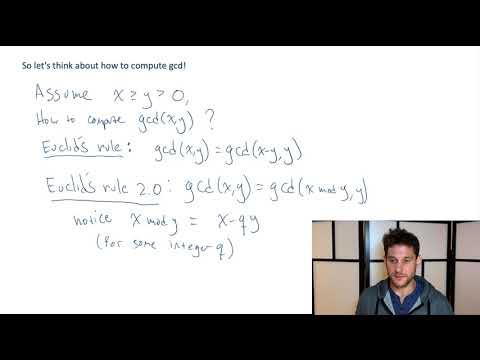 0:14:22
0:14:22
 0:10:06
0:10:06
 0:06:25
0:06:25