filmov
tv
Basics of Modular Arithmetic

Показать описание
If you need to post a picture of your solution or idea:
#NumberTheoryTopics #ModularArithmetic
EXPLORE 😎:
PLAYLISTS 🎵 :
ADDITIONAL TOPICS:
Chinese Remainder Theorem
Fermat’s Little Theorem
Euler’s Theorem
Primitive Roots and Power Residues
Hensel’s Lemma
Quadratic Residues and Quadratic Reciprocity
RESOURCES:
#NumberTheoryTopics #ModularArithmetic
EXPLORE 😎:
PLAYLISTS 🎵 :
ADDITIONAL TOPICS:
Chinese Remainder Theorem
Fermat’s Little Theorem
Euler’s Theorem
Primitive Roots and Power Residues
Hensel’s Lemma
Quadratic Residues and Quadratic Reciprocity
RESOURCES:
What is Modular Arithmetic - Introduction to Modular Arithmetic - Cryptography - Lesson 2
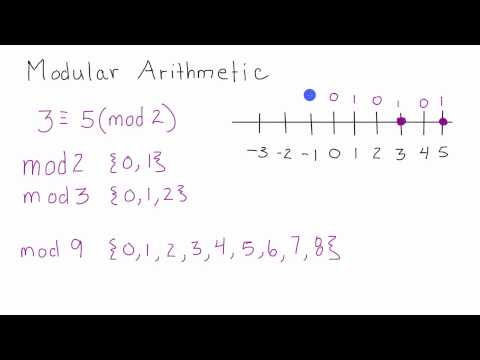
Basics of Modular Arithmetic
What does a ≡ b (mod n) mean? Basic Modular Arithmetic, Congruence
Modular Arithmetic (Part 1)
This completely changed the way I see numbers | Modular Arithmetic Visually Explained
How does Modular Arithmetic work?
Modular Arithmetic Equations
Modular Arithmetic Basics
Understanding Mathematical Symbols The Meaning of Equality, Proportionality, and Inequality
MODULAR ARITHMETIC | INTRODUCTION
Modular Arithmetic Basics: Congruence mod n
Modular Arithmetic | A Full Course | Maths Center
[Discrete Mathematics] Modular Arithmetic
Discrete Math - 4.1.2 Modular Arithmetic
Mod By A Group: Generalized Modular Arithmetic, from Basic Modular Arithmetic Congruence to 'No...
Understanding Modular Arithmetic - Introduction to Modular Arithmetic - Part 1
Modulus Operator - CS101 - Udacity
■ What is Modular Arithmetic? | An introduction to the strange world of mathematical time-telling
Modulo Operator Examples #Shorts #math #maths #mathematics #computerscience
Modulo
Modular arithmetic!
Modular Arithmetic Basics
Solving congruences, 3 introductory examples
Modular Arithmetic Part-1 Explained with Examples in Hindi
Комментарии
 0:04:48
0:04:48
 0:18:39
0:18:39
 0:06:02
0:06:02
 0:10:57
0:10:57
 0:20:33
0:20:33
 0:11:19
0:11:19
 0:08:26
0:08:26
 0:04:48
0:04:48
 0:00:55
0:00:55
 0:07:00
0:07:00
 0:15:21
0:15:21
 2:21:16
2:21:16
![[Discrete Mathematics] Modular](https://i.ytimg.com/vi/d-n92Ml1iu0/hqdefault.jpg) 0:08:27
0:08:27
 0:22:27
0:22:27
 0:15:25
0:15:25
 0:08:30
0:08:30
 0:01:08
0:01:08
 0:12:42
0:12:42
 0:00:30
0:00:30
 0:04:07
0:04:07
 0:00:27
0:00:27
 0:00:26
0:00:26
 0:03:51
0:03:51
 0:08:07
0:08:07