filmov
tv
Derivatives... What? (NancyPi)
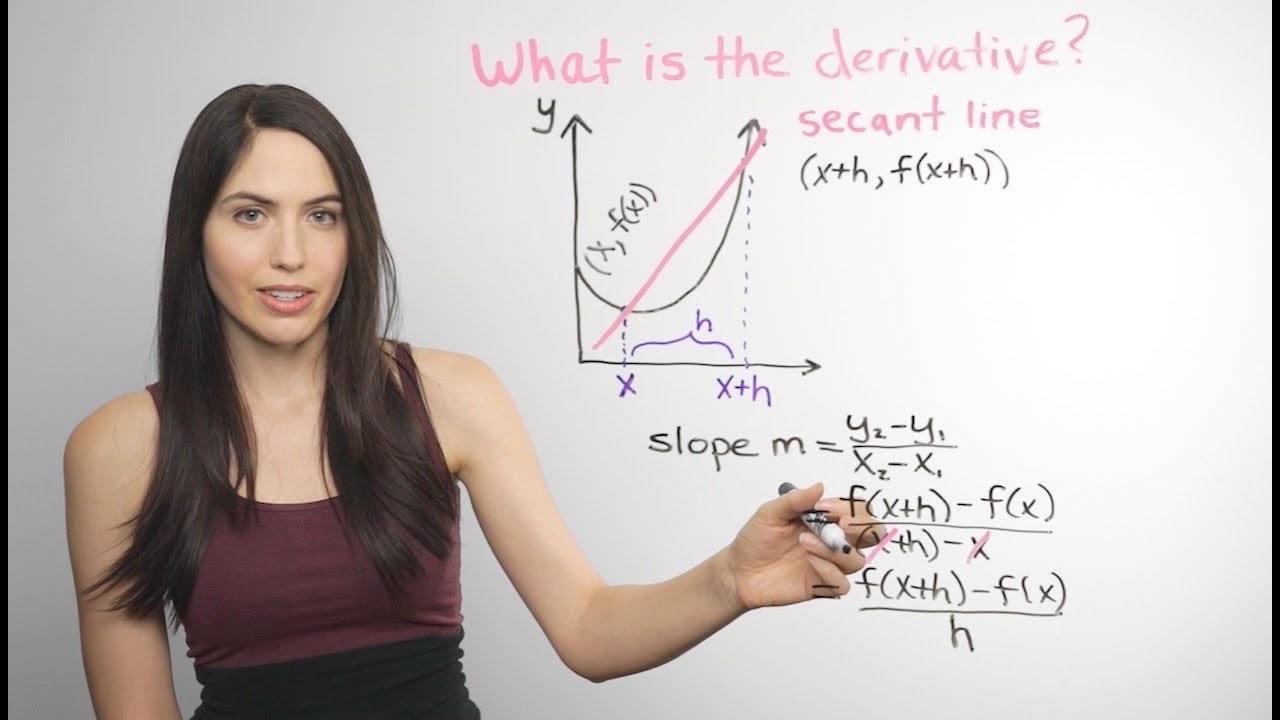
Показать описание
MIT grad shows the DEFINITION of the derivative and how to FIND the derivative using that limit definition. To skip ahead: 1) For what the derivative MEANS, skip to 0:23. 2) For the SLOPE OF THE SECANT line, skip to 2:53. 3) For the SECANT BECOMING THE TANGENT and DEFINITION OF THE DERIVATIVE, skip to 5:54. 4) For how to FIND THE DERIVATIVE USING THE (LIMIT) DEFINITION of the derivative, skip to 10:28. Nancy formerly of MathBFF explains the steps.
INTRODUCTION to derivatives:
1) WHAT IS THE DERIVATIVE? It's a function that tells you the slope (of the line tangent to the curve) at every point. Another way to think of it is that the derivative gives you the rate of change at any instant (the "instantaneous rate of change" at each point). You can find the derivative either with the proper DEFINITION OF THE DERIVATIVE ("by the limit process") or the faster, simpler way with the shortcut derivative rules such as the Power Rule, Product Rule, Quotient Rule, and Chain Rule. This video shows the first way, with the DEFINITION of the derivative, and how to use it to calculate the derivative.
2) SLOPE OF SECANT: To make the definition of the derivative, we can start with the slope of the straight secant line through a point x and some other point nearby, h distance away horizontally. If we label the two points and use the slope formula to write an expression for the slope of this secant line, the expression you get is [f(x+h) - f(x)] / h, which is also known as the DIFFERENCE QUOTIENT.
3) DEFINITION OF DERIVATIVE: That straight line slope we just found is actually a decent ESTIMATE for the slope at x, but it's not really the slope at x. We can get the EXACT slope of the tangent line at x by closing in on x and narrowing h to zero, by taking the LIMIT of the secant slope, as h approaches 0. The slope of the secant line becomes the slope of the tangent line. Not only is this limit equal to the slope of the tangent line, it is the definition of the derivative (when the limit exists) or f'(x).
INTRODUCTION to derivatives:
1) WHAT IS THE DERIVATIVE? It's a function that tells you the slope (of the line tangent to the curve) at every point. Another way to think of it is that the derivative gives you the rate of change at any instant (the "instantaneous rate of change" at each point). You can find the derivative either with the proper DEFINITION OF THE DERIVATIVE ("by the limit process") or the faster, simpler way with the shortcut derivative rules such as the Power Rule, Product Rule, Quotient Rule, and Chain Rule. This video shows the first way, with the DEFINITION of the derivative, and how to use it to calculate the derivative.
2) SLOPE OF SECANT: To make the definition of the derivative, we can start with the slope of the straight secant line through a point x and some other point nearby, h distance away horizontally. If we label the two points and use the slope formula to write an expression for the slope of this secant line, the expression you get is [f(x+h) - f(x)] / h, which is also known as the DIFFERENCE QUOTIENT.
3) DEFINITION OF DERIVATIVE: That straight line slope we just found is actually a decent ESTIMATE for the slope at x, but it's not really the slope at x. We can get the EXACT slope of the tangent line at x by closing in on x and narrowing h to zero, by taking the LIMIT of the secant slope, as h approaches 0. The slope of the secant line becomes the slope of the tangent line. Not only is this limit equal to the slope of the tangent line, it is the definition of the derivative (when the limit exists) or f'(x).
Комментарии
 0:14:30
0:14:30
 0:14:30
0:14:30
 0:14:17
0:14:17
 0:18:44
0:18:44
 0:11:56
0:11:56
 0:16:36
0:16:36
 0:15:37
0:15:37
 0:06:05
0:06:05
 0:04:05
0:04:05
 0:06:53
0:06:53
 0:12:48
0:12:48
 0:03:14
0:03:14
 0:05:21
0:05:21
 0:16:42
0:16:42
 0:25:48
0:25:48
 0:18:00
0:18:00
 0:19:15
0:19:15
 0:12:40
0:12:40
 0:00:58
0:00:58
 0:00:35
0:00:35
 0:23:58
0:23:58
 0:07:35
0:07:35
 0:10:57
0:10:57
 0:02:26
0:02:26