filmov
tv
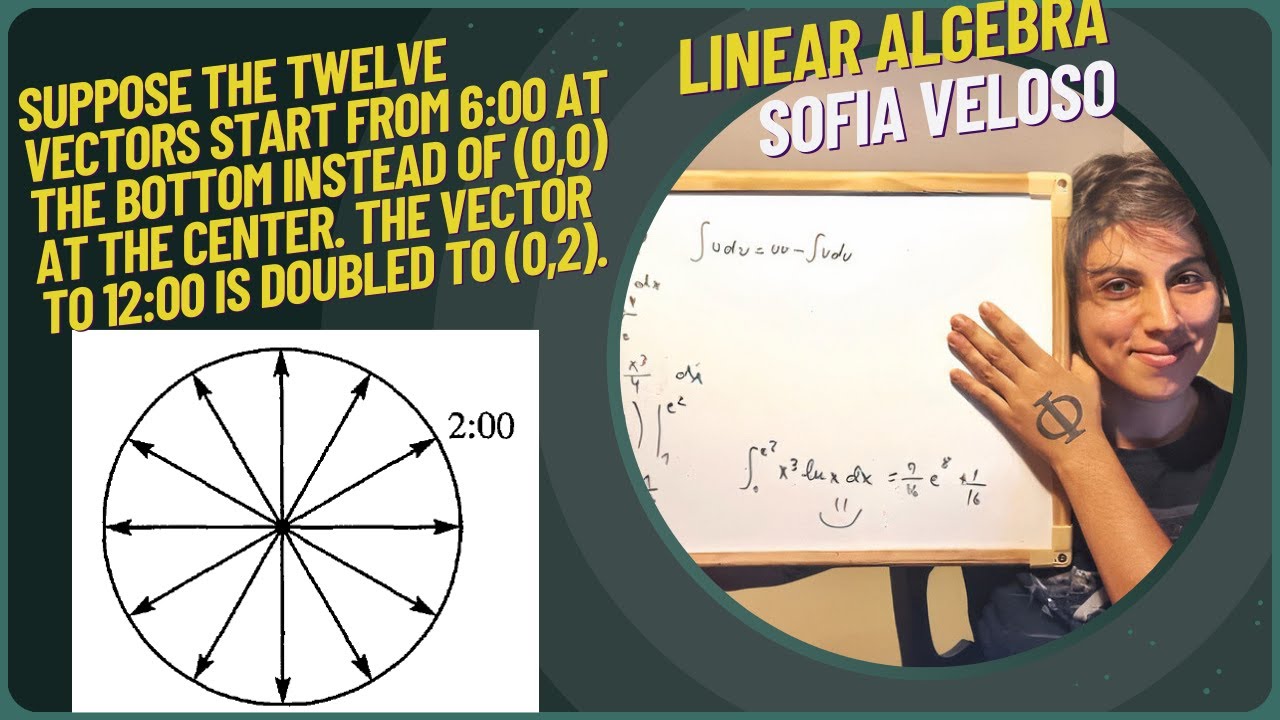
1.1.14 Suppose the twelve vectors start from 6:00 at the bottom instead of (0,0) at the center
Показать описание
Problem 1.1.14 From Gilbert Strang's Introduction to Linear Algebra fourth edition. Chapter 1 - introduction to vectors - vectors and linear combinations.
Enjoy, and I am available for tutoring and private classes! :)
Suppose the twelve vectors start from 6:00 at the bottom instead of (0,0) at the
center. The vector to 12:00 is doubled to (0,2). Add the new twelve vectors.
Enjoy, and I am available for tutoring and private classes! :)
Suppose the twelve vectors start from 6:00 at the bottom instead of (0,0) at the
center. The vector to 12:00 is doubled to (0,2). Add the new twelve vectors.
1.1.14 Suppose the twelve vectors start from 6:00 at the bottom instead of (0,0) at the center
Linear Algebra Example Problems - Coordinate System Representation Example #1
Eigenvectors and eigenvalues | Chapter 14, Essence of linear algebra
How To Find The Equation of a Plane Given Three Points
How to determine if one vector is in the span of other vectors?
How to find out if a set of vectors are linearly independent? An example.
How to Find the Matrix of a Linear Transformation
Linear Algebra: Norm
Introduction to orthonormal bases | Linear Algebra | Khan Academy
Linear transformations | Matrix transformations | Linear Algebra | Khan Academy
Intro to Matrices
Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra
Linear transformations and matrices | Chapter 3, Essence of linear algebra
How To Find The Directional Derivative and The Gradient Vector
[Linear Algebra] Linear Transformations
Volume of the parallelepiped determined by vectors (KristaKingMath)
Solving congruences, 3 introductory examples
Orthogonal, parallel or neither (vectors) (KristaKingMath)
Suppose the vectors \( x_{1}, x_{2} \) and \( x_{3} \) are the solu...
Triangle Inequality for Real Numbers Proof
Representation of signed number | sign magnitude form | 1's complement and 2's complement...
Solving Matrix Equations
Moment generating functions -- Example 1
Introduction to Relative motion!
Комментарии
 0:03:57
0:03:57
 0:05:54
0:05:54
 0:17:16
0:17:16
 0:06:56
0:06:56
 0:05:00
0:05:00
 0:04:41
0:04:41
 0:05:19
0:05:19
 0:31:21
0:31:21
 0:11:16
0:11:16
 0:13:52
0:13:52
 0:11:23
0:11:23
 0:09:59
0:09:59
 0:10:59
0:10:59
 0:28:30
0:28:30
![[Linear Algebra] Linear](https://i.ytimg.com/vi/cFIRXQBfgg0/hqdefault.jpg) 0:12:30
0:12:30
 0:07:02
0:07:02
 0:03:51
0:03:51
 0:06:57
0:06:57
 0:04:39
0:04:39
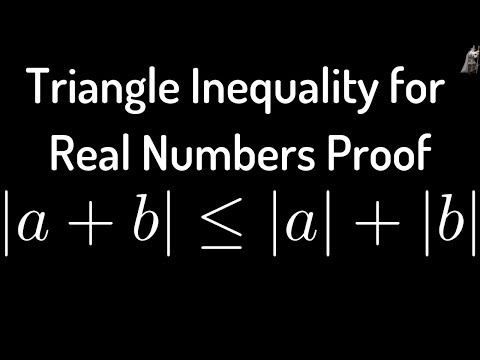 0:03:08
0:03:08
 0:05:56
0:05:56
 0:06:31
0:06:31
 0:05:19
0:05:19
 0:02:04
0:02:04