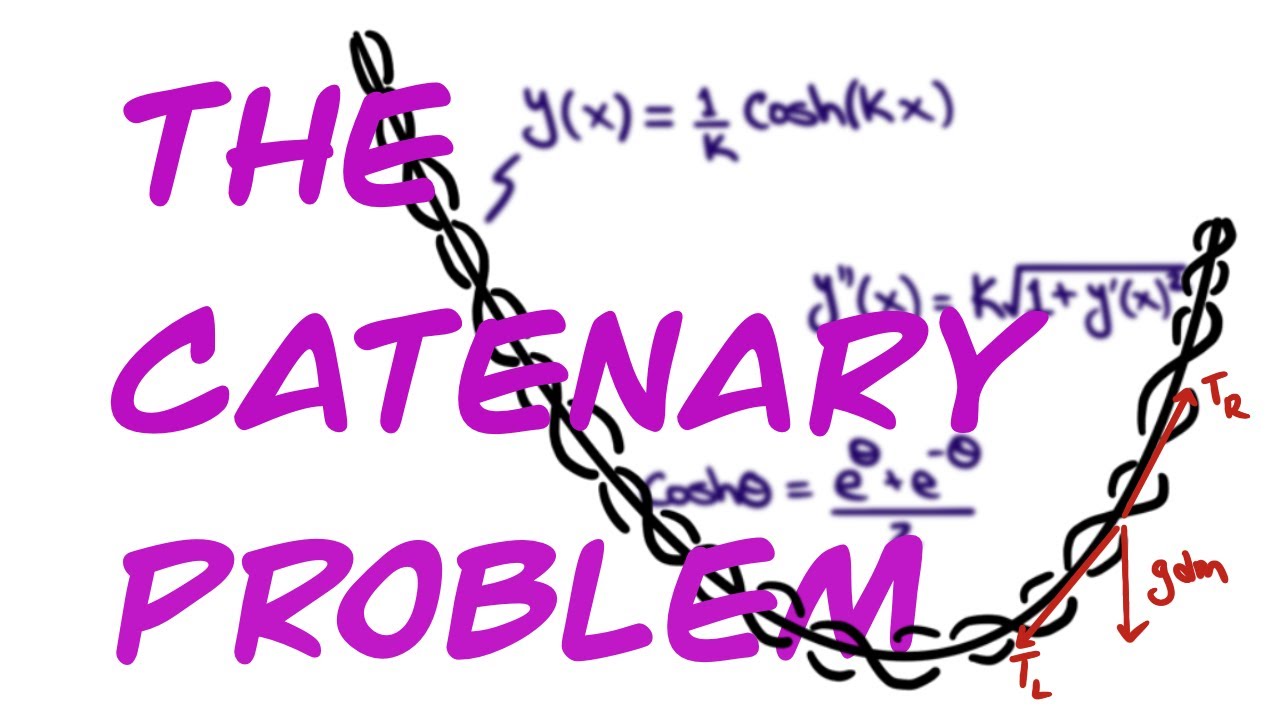
How Do You Find The Shape of Hanging Rope? Classic Physics Problem

When you hang up a rope between two points under the weight of gravity, it makes a distinctive arc-like shape that anyone can instinctively recognize. In this video, I'll show you how to actually derive the shape---and find the mathematical function that describes it---using F = ma and a little calculus.

About the classic physics problem series:
In these intro-to-intermediate-level physics videos, I'll discuss classic physics "challenge" problems that you might meet in your introductory mechanics and electromagnetism classes. They might be based on simple concepts, but these problems can still get pretty tough!

About me:

[dukenukem]

What a fun little problem! I can wait to do it with my son. Somehow, I made it all the way through a Ph.D. in physics without seeing this problem. Thanks for posting!

[alphalunamare]

Many years ago in my first job before going to college I was asked to work out how much concrete a bridge needed. The shape was a catenary so I figured I'd work out the length. It took me 2 days and when I gave the answer, the structural engineer put a piece of string along the drawing and said Yes you're right. I felt so dumb. I had used integration with substitution a few times and there were square roots everywhere but the answer was correct. Then a graduate asked me why I hadn't used hyperbolic functions? What are they I asked? So anyway I looked them up and just couldn't believe how easy they made everything. I quit my job and went to college :-)

[kingbeauregard]

I really like the moment when you say (I paraphrase), "well, that's all the physics, now it's just math". That's a good way to look at these problems.

I seem to recall there's a way to derive the catenary by using the Lagrangian, though if you do it wrong, the result you get is that the rope just falls to the ground.

[Salien]

Excellent. I'm especially happy that you didn't just get to the integral part and say "if we look at an integration table, we find that this is the integral for cosh." That always seemed like a non-explanation for me.

[utuberaj]

Mr. Elliot- you made this derivation look so simple. It was really interesting and from 1st principles.
I appreciate your videos on ALL Physics topics you have made, some are quite advanced.
Why don't you make a video of the derivation of the famous curves of the 17th century - the Brachistochrone and the Tautochrone- that took the brilliance of the Bernoullis and Newton and Leinlbniz. The Tautochrone was discovered by another genius Huygens.

[hrperformance]

This is the first time I've seen this. Great explanation. I had to pause and think a few times but I was able to follow pretty well.
Super enjoyable! Many thanks for posting 😁

[ccdavis]

3 in a row, all very well done.
Top ranked reference/learning resource.
subbed

[adrianf.]

To make the change of variables u = iv rigorous, I think one has to consider contour integrals. As an alternative, I recently solved a similar integral (int sqrt(x^2 + 1)) using the identity sinh^2 + 1 = cosh^2.

Please note that T(x) should point "in the same direction as" T(x + dx).

In total, you managed with only one mistake, which makes this frankly the best physics video I ever watched, including many by distinguished professors.

[mohammedal-haddad]

I read this proof in a book and it was very hard to follow, you make it so easy and fun. Thank you very much.

[arnoldkotlyarevsky]

This problem has bothered me since undergrad. I went to gradschool for physics and while I got better at math and answering test questions this never really connected for me. Im now in my thirties, and I have periodically tried to revisit this to see if I could make it feel more intuitive. Thank you for putting this out.

[moonwatcher]

Really nice!!! Calm talking pace, clear and interesting explanation, rigorous but entertaining stuff. Gorgeous video, mate. Thanks

[johnchristian]

This is a great video. Amazing how something so simple as a hanging rope has such deep physics and math.

[darkolking]

Great video as usual. I learn, again 🤗, something new and that makes me very happy

[chandlerketelsen]

Fantastic! I am taking modern physics, thermodynamics, and a few other courses right now for my physics major at Washington State University. Seeing these videos makes me enthusiastic to put effort into my classes which will help me with my degree and beyond. I appreciate this!

[TIOS]

Excellent. It’s been a very long time since did this problem. I’d have had a very difficult time working through it myself.

[juliencasel]

I got asked this on an oral interrogation back when I was an undergrad student. I knew at the time about the "trick" of applying Newton's law to a small piece of the rope, but I was still completely helpless... 25 years later I finally grabbed a piece of paper and tried to figure it out... and it was way harder than I thought.

I actually spent an shameful amount of time solving the differential equation, actually I don't think I resolved it directly but I used some tricks to simplify it. In the end I got the cosh but it was reaaally painful.

[jimgarrett]

One of my favorite centenary are power lines across a canyon. They are marked on aeronautical charts. Why? You can see them from the air (so you can know where you are) and more importantly so you don't run into them.

[michaelmcgee]

A little above my head, gleaned a little, good video.

[informalchipmunk]

7:57 alternatively, we could substitute:
u = tanθ
=> du = sec²θ•dθ
and also,
1 + u² = 1 + tan²θ = sec²θ
hence we have:
∫(sec²θ/√(sec²θ)•dθ
= ∫secθ•dθ
Now some of you may already know the result to that integral, if not then we can multiply and divide by cosθ to get:
∫(cosθ/(1 - sin²θ))•dθ
Now let sinθ = v
=> cosθ•dθ = dv
Now we have ∫(1/(1 - v²))•dv
Which is a pretty simple partial fraction integral -which I'm too lazy to type- which is left as a challenge to the reader.

We finally arrive at ln(|secθ + tanθ|) + c
Now just sub θ = tan⁻¹u
And as I finish writing this I've realised that your method is far superior 😂.

[Nekuzir]

Hey I was right, its the hyperbolic trig stuff. I haven't done the actual maths with it but its nice being able to recognize it when it shows up.