filmov
tv
Golden Ratio Visual Computation
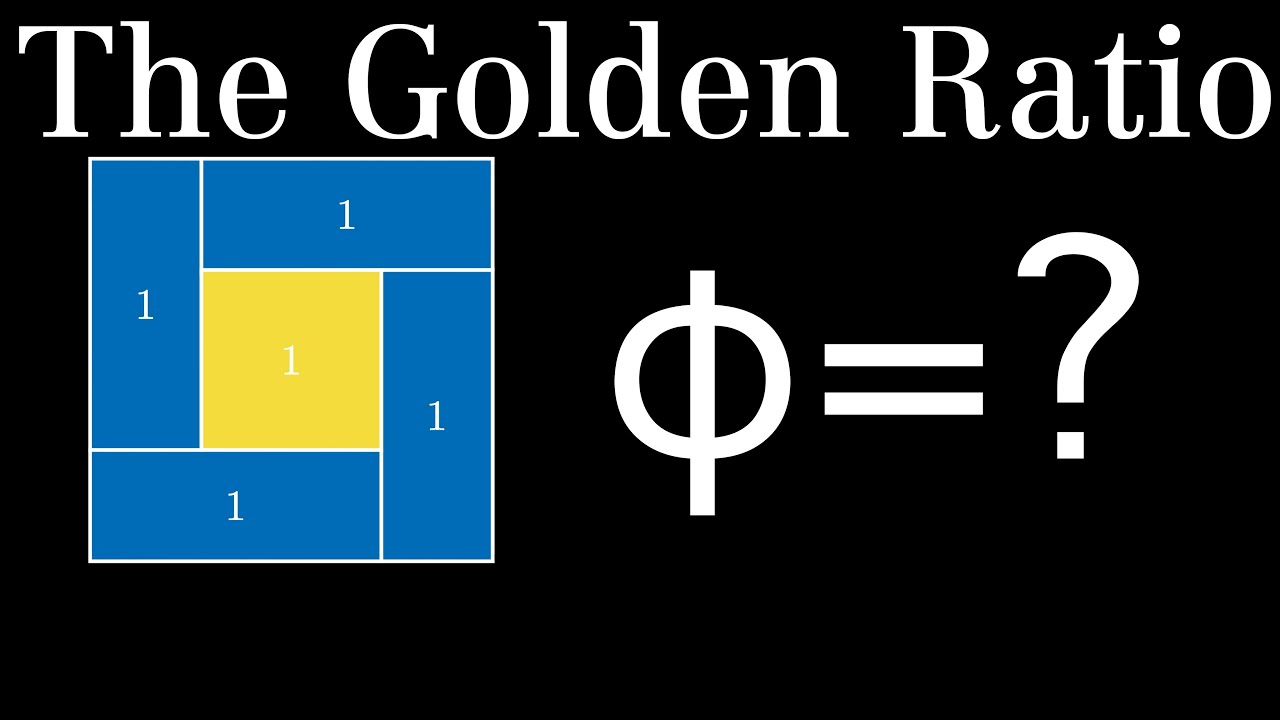
Показать описание
This is a short, animated visual proof of showing how to compute the value of the golden ratio (which is the positive number satisfying x=1+1/x) without using the quadratic formula explicitly. Instead, we use a Tatami diagram and compute the areas of the included rectangles and squares to find the total area of the outer square in two ways.. #manim #math #mathvideo #goldenratio #goldensection #mathshorts #geometry #animation #theorem #pww #proofwithoutwords #visualproof #proof #area #algebra #pww #proof #areas #mathematics #mtbos
Check out these related videos:
If you like this video, please click "like" and consider subscribing and checking out my other videos.
To learn more about animating with manim, check out:
_____________________________
Music in this video:
Creative Commons Attribution-ShareAlike 3.0 Unported
Check out these related videos:
If you like this video, please click "like" and consider subscribing and checking out my other videos.
To learn more about animating with manim, check out:
_____________________________
Music in this video:
Creative Commons Attribution-ShareAlike 3.0 Unported
Golden Ratio Visual Computation
The Golden Ratio: Myth or Math?
Golden ratio!
Drawing the Golden Ratio
Animation: The easiest way to find Golden Ratio | mathocube |
A Golden Ratio Infinite Series Dissection (visual proof)
Golden Ratio is Irrational from a Regular Pentagon (visual proof)
Golden Ratio & How It Is Calculated : Math & Algebra
What is the Fibonacci Sequence & the Golden Ratio? Simple Explanation and Examples in Everyday L...
Golden Ratio = Mind Blown!
The magic of Fibonacci numbers | Arthur Benjamin | TED
Golden Ratio Explained | Golden Ratio Spiral #architecture #design
Golden Section Search Method
Short Animations: Fibonacci Spiral | Animated video by Mathing
Golden Ratio Composition Secret!
The Golden Ratio in Engineering : Math Tips
Calculating the Golden Ratio Geometrically
Golden Ratio for Art Beginners #ArtHistory
Why is 1.618034 So Important?
Calculating the golden proportion in Photoshop (in one minute)
Fibonacci Sequence
How To Draw a Golden Ratio Rectangle & Spiral | Sacred Geometry Tutorial
Way beyond the golden ratio: The power of AB=A+B (Mathologer masterclass)
The Golden Ratio rolling in Euler's constant hypocloids (visualization)
Комментарии
 0:02:29
0:02:29
 0:04:40
0:04:40
 0:00:59
0:00:59
 0:00:59
0:00:59
 0:01:23
0:01:23
 0:03:13
0:03:13
 0:03:56
0:03:56
 0:01:34
0:01:34
 0:05:01
0:05:01
 0:09:46
0:09:46
 0:06:25
0:06:25
 0:00:31
0:00:31
 0:07:43
0:07:43
 0:01:01
0:01:01
 0:18:43
0:18:43
 0:01:41
0:01:41
 0:12:29
0:12:29
 0:08:39
0:08:39
 0:09:37
0:09:37
 0:01:12
0:01:12
 0:00:15
0:00:15
 0:03:28
0:03:28
 0:49:49
0:49:49
 0:01:33
0:01:33