filmov
tv
Linear Algebra - Lecture 35 - Diagonalizable Matrices
Показать описание
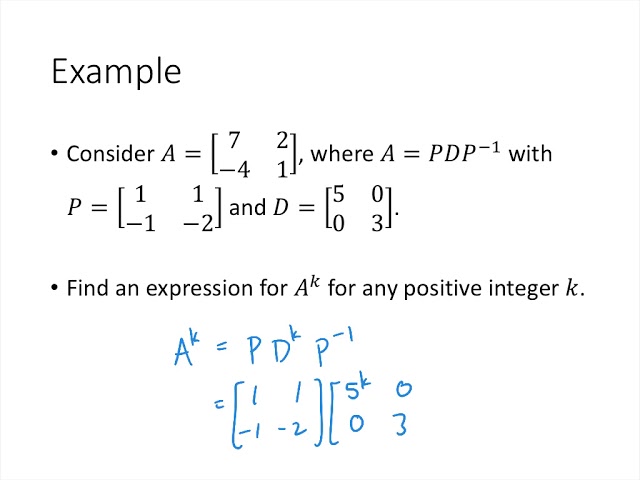
In this lecture, we discuss what it means for a square matrix to be diagonalizable. We prove the Diagonalization Theorem, which tells us exactly when a matrix is diagonalizable.
0:08:54
Linear Algebra - Lecture 35 - Diagonalizable Matrices
0:36:39
Linear Algebra Lecture 35 - Linear Transformations | Proving a function is a linear transformation
0:09:43
Diagonalization of matrices | Lecture 35 | Matrix Algebra for Engineers
0:26:46
Linear Algebra - Lecture 35: Computing the Determinant (via Gaussian Elimination)
0:06:42
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (30 of 35) Power Method A^n, A^5
0:13:53
Linear Algebra - Lecture 34 - The Characteristic Equation
0:14:34
Linear Algebra 35 | Rank-Nullity Theorem
0:20:47
Lecture 35: Linear Algebra (dimension of a vector space)
1:01:44
Ch 3 Linear Regression | Exercise 3.3 (Q6 - Q.14) | Class 12 HSC Commerce | Maths 2 @GalaxyofMaths
0:06:20
Algebra - Ch. 35: Systems of of Linear Eq. in 3 Variables (11 of 25) Method 5: Augmented Matrix
0:05:20
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (1 of 35) What Are Eigenvalues? (Part 1)
0:08:46
Advanced Linear Algebra - Lecture 35: Geometric Interpretation of the Singular Value Decomposition
0:14:34
Linear Algebra 35 | Rank-Nullity Theorem [dark version]
0:07:57
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (9 of 35) Bases and Eigenvalues: 1
0:09:05
Linear Algebra: Ch 2 - Determinants (35 of 48) Find Determinant=? by Reducing to Echleon Form: 3
0:07:02
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (25 of 35) Diagonalization (Part 1/3)
0:03:53
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (7 of 35) Given the Eigenvector, Eigenvalues=?
0:04:33
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (29 of 35) Power Method A^3
0:03:36
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (27 of 35) Diagonalization (Part 3/3)
0:30:10
Lecture 35 | Applied Linear Algebra | Vector Properties | Prof AK Jagannatham
0:08:44
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (8 of 35) Eigenvector=? of a 3x3 Matrix
0:05:23
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (10 of 35) Bases and Eigenvalues: 2
0:05:16
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (12 of 35) Basis=? for a 3x3 Matrix: Ex. 1
0:09:42
Line Integral of a Scalar Field | Lecture 35 | Vector Calculus for Engineers
Комментарии