filmov
tv
Find the homogeneous solution to the Cauchy-Euler second order differential equation

Показать описание
Find the homogeneous solution to the Cauchy-Euler second order differential equation
Aboard the M-theory laboratory spaceship, Augustin-Louis Cauchy and Leonhard Euler, equipped with nothing but pen, paper, and their brilliant minds, tackle the second order differential equation through a methodical approach. They start by recognizing the equation as a Cauchy-Euler form, indicative of its characteristic roots solving method. Assuming a solution of the form y=x^m, they substitute this back into the equation to derive a characteristic polynomial. The key step involves calculating the roots of this polynomial, leading them to a general solution in terms of these roots. Through their collaboration, they artfully navigate the complexities of the equation, employing a mix of intuition and mathematical precision, ultimately deriving a solution that showcases the beauty of mathematical problem-solving and the power of intellectual collaboration in the serene, high-tech confines of their futuristic workspace.
Aboard the M-theory laboratory spaceship, Augustin-Louis Cauchy and Leonhard Euler, equipped with nothing but pen, paper, and their brilliant minds, tackle the second order differential equation through a methodical approach. They start by recognizing the equation as a Cauchy-Euler form, indicative of its characteristic roots solving method. Assuming a solution of the form y=x^m, they substitute this back into the equation to derive a characteristic polynomial. The key step involves calculating the roots of this polynomial, leading them to a general solution in terms of these roots. Through their collaboration, they artfully navigate the complexities of the equation, employing a mix of intuition and mathematical precision, ultimately deriving a solution that showcases the beauty of mathematical problem-solving and the power of intellectual collaboration in the serene, high-tech confines of their futuristic workspace.
 0:06:41
0:06:41
 0:26:55
0:26:55
 0:12:44
0:12:44
 0:08:33
0:08:33
 0:07:59
0:07:59
 0:02:03
0:02:03
 0:12:52
0:12:52
 0:05:37
0:05:37
 0:50:54
0:50:54
 0:41:28
0:41:28
 0:01:47
0:01:47
 0:17:05
0:17:05
 0:06:30
0:06:30
 0:05:22
0:05:22
 0:09:09
0:09:09
 0:06:57
0:06:57
 0:01:47
0:01:47
 0:25:17
0:25:17
 0:08:21
0:08:21
 0:07:47
0:07:47
 0:22:28
0:22:28
 0:17:09
0:17:09
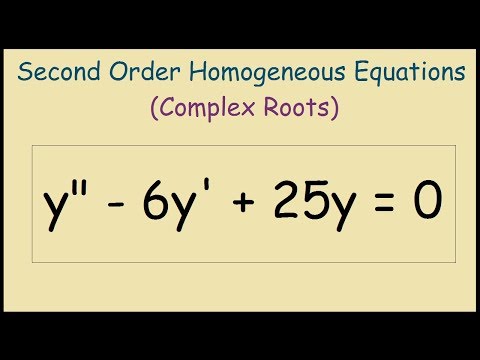 0:02:54
0:02:54
 0:26:57
0:26:57