filmov
tv
Complex Analysis: Nasty Integral with Elegant Solution

Показать описание
Today, we evaluate a very difficult-looking integral (at least for real methods) using complex analysis.
Complex Analysis: Nasty Integral with Elegant Solution
Complex Analysis: Another Integral Snack
Cauchy Integral Formula with Examples - Complex Analysis by a Physicist
A stellar integral solved using some wonderful complex analysis
Complex Analysis: Integral of log(x)/(x+1)^2
Complex Analysis L08: Integrals in the Complex Plane
Evaluating Real Integrals With Cauchy's Residue Theorem - Complex Analysis By A Physicist
Complex Analysis: Integral of 1/(x^n+1) feat. pizza contour
Complex Analysis: Integral of 1/(x^4-x^2+1) using Contour Integration
Complex Analysis 26 | Keyhole contour
EX: A contour integral with higher-order poles
Complex Analysis: An Integral from @MichaelPennMath
Complex Analysis L10: Cauchy Integral Formula
Complex Analysis: Cauchy's Integral Theorem
Complex Analysis L09: Complex Residues
Complex Analysis: Integral of sech(ax) using Contour Integration
Complex Analysis: Integral of log(x^2+1)/x^2
Complex Analysis - Line Integrals
Complex Analysis: Double Keyhole Contour
Complex Analysis: One EXOTIC Integral
Complex Analysis-ing a CRAZY Integral
Complex Analysis: Is this solution way too overkill?
Outtakes - Integral of ln(x)/(x^n+1) with Complex Analysis
Insane Contour Integral from @maths_505 !
Комментарии
 0:24:48
0:24:48
 0:07:40
0:07:40
 0:09:27
0:09:27
 0:20:29
0:20:29
 0:24:46
0:24:46
 0:41:29
0:41:29
 0:16:06
0:16:06
 0:36:33
0:36:33
 0:18:31
0:18:31
 0:08:37
0:08:37
 0:07:31
0:07:31
 0:34:00
0:34:00
 0:16:43
0:16:43
 0:08:04
0:08:04
 0:24:51
0:24:51
 0:25:44
0:25:44
 0:34:36
0:34:36
 0:20:37
0:20:37
 0:41:33
0:41:33
 0:37:03
0:37:03
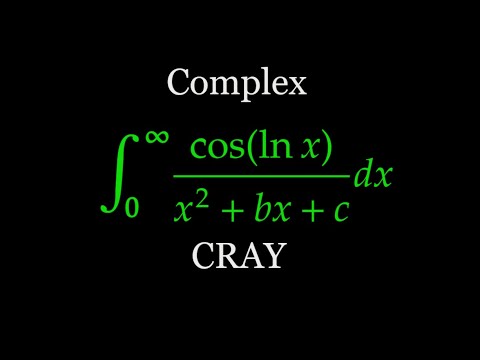 0:24:10
0:24:10
 0:25:14
0:25:14
 0:23:52
0:23:52
 0:17:48
0:17:48