filmov
tv
Complex Analysis - Line Integrals

Показать описание
Complex Analysis - Computing Line Integrals
Complex Analysis L08: Integrals in the Complex Plane
Complex integration, Cauchy and residue theorems | Essence of Complex Analysis #6
Complex Analysis: what is a contour integral?
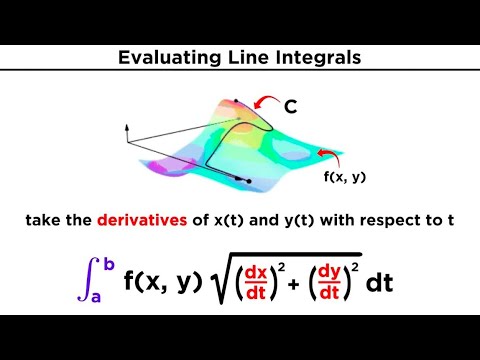
Evaluating Line Integrals
Complex Analysis - Line Integrals
What is a LINE INTEGRAL? // Big Idea, Derivation & Formula
Complex Analysis - Complex Integration Line Integral Example & Solution
22. Volume of Solid of Revolution in Polar Form | Problem 3 | Integral Calculus
Line Integrals. #calculus
Complex integration||Line integrals of complex functions||Complex analysis||Lecture 12
21. Line integrals (Cultivating Complex Analysis 3.1 part 1)
Complex Line Integrals First Evaluation Method
Complex Analysis #4 | Complex line integrals
Complex Analysis | Unit 2 | Lecture 1 | Trace of a Curve and Complex Line Integral
The *Complex* Integral of (-1)^x
Stokes Theorem vs Greens Theorem (circulation)
Complex Analysis : Complex line Integral Part 1
Intro Complex Analysis, Lec 23, Real Line Integrals and Applications, Complex Integration
Complex Analysis | Unit 2 | Lecture 13 | Example of Cauchy's Integral Formula
Complex Analysis : Complex Line Integral
COMPLEX INTEGRATION (LINE INTEGRAL PART 1)
Complex Integrals | Contour Integration | Complex Analysis #11
Evaluating Line Integral # Line Integrals # Complex Analysis
Комментарии
 0:07:03
0:07:03
 0:41:29
0:41:29
 0:40:45
0:40:45
 0:10:15
0:10:15
 0:12:54
0:12:54
 0:20:37
0:20:37
 0:14:02
0:14:02
 0:16:36
0:16:36
 0:17:12
0:17:12
 0:00:51
0:00:51
 0:35:31
0:35:31
 0:24:24
0:24:24
 0:09:27
0:09:27
 0:16:46
0:16:46
 0:07:41
0:07:41
 0:00:51
0:00:51
 0:00:08
0:00:08
 0:30:22
0:30:22
 0:54:39
0:54:39
 0:09:05
0:09:05
 0:06:16
0:06:16
 0:18:29
0:18:29
 0:14:05
0:14:05
 0:01:00
0:01:00