Limits 'at' infinity

Description:
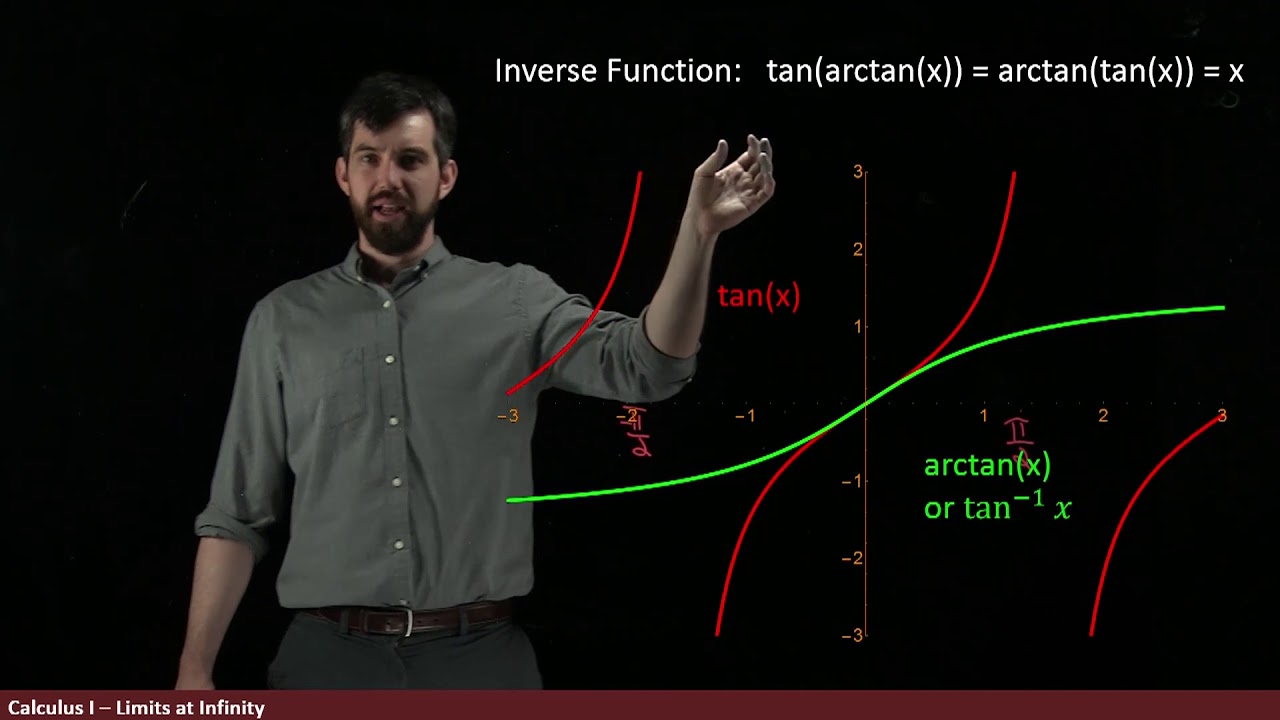
As x tends to either positive or negative infinity, the the functions can tend towards fixed values creating horizontal asymptotes.

Learning Objectives:
1) Compute limits at infinity

Now it's your turn:
1) Summarize the big idea of this video in your own words
2) Write down anything you are unsure about to think about later
3) What questions for the future do you have? Where are we going with this content?
4) Can you come up with your own sample test problem on this material? Solve it!

Learning mathematics is best done by actually DOING mathematics. A video like this can only ever be a starting point. I might show you the basic ideas, definitions, formulas, and examples, but to truly master calculus means that you have to spend time - a lot of time! - sitting down and trying problems yourself, asking questions, and thinking about mathematics. So before you go on to the next video, pause and go THINK.

This video is part of a Calculus course taught by Dr. Trefor Bazett at the University of Cincinnati.

BECOME A MEMBER:

MATH BOOKS & MERCH I LOVE:

[nrkv]

Great lecture professor, thank you.
I wish you could provide us an exercises pdfs

[ancadeva]

I spent a lot of time calculating from values this limits. I like the graphic method you propose. Maybe a lot of points with their values were more suggestive to the arctan. I am an left handed too, but you do really write and read from right to left?

[qqtrol]

Aren't x and 1/x inverse functions of themselves? You stated it like they were inverse functions of the other.

[wqltr]

Wait, aren't x and 1/x not inverse functions. They are sometimes called inverses because their product is 1. But the inverse FUNCTION of 1/x is 1/x, since 1/(1/x) = x