filmov
tv
How To Solve Amazon's Pair Of Socks Interview Puzzle

Показать описание
This problem was part of a written interview test for Amazon in India. A drawer contains 12 identical black socks and 12 identical white socks. If you pick 2 socks at random, what is the probability of getting a matching pair?
My blog post for this video
Source of puzzle
Connect on social media. I update each site when I have a new video or blog post, so you can follow me on whichever method is most convenient for you.
My Books
My blog post for this video
Source of puzzle
Connect on social media. I update each site when I have a new video or blog post, so you can follow me on whichever method is most convenient for you.
My Books
How To Solve Amazon's Pair Of Socks Interview Puzzle
Amazon fire tv remote pairing(enter button & home button)same time hold in 10sec#Mr.service
how to sync or Pair different left and right samsung galaxy bud plus - Fix
Fire stick remote pairing....
What Happened to my Firestick Remote | Let's Pair it!!! | FLIXBOLT
How Connect PAIR New Not Working REMOTE Amazon Fire TV FireStick Device Stick Install LY73PR w87cun
Is This Pair of Nike TN Fake? #sneakerheads #sneakers #viral
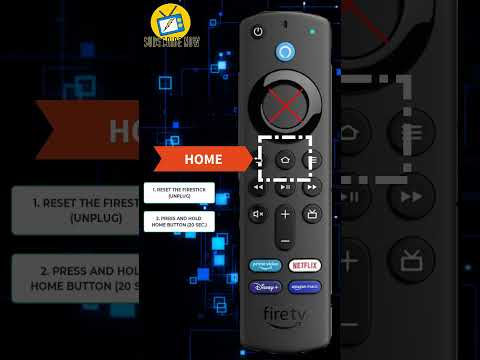
How to Fix Amazon Fire TV Remote Not Pairing or Syncing (Easy Method)
I've LOST CONTROL!! Faulty SEGA Master System 2 - Fix it Friday
Amazon Fire TV: How to Pair Remote (Only Power button working?) Fixed!
Amazon Echo Buds: How to Factory Reset (issues connecting, pairing, one works, etc)
How to SYNC & Pair Firestick Remote that Won't Connect (Easy Tutorial)
How to Connect or Pair your DualShock 4 to your PlayStation 4 without a USB cable #gaming #ps4
How to PAIR New Firestick Remote & Without Old Remote (Fast Tutorial)
Beats Powerbeats Pro: How to Hard Reset (problems pairing or connecting?) FIXED!
How to Turn On Pairing Mode - Google Pixel Buds Pro
✨baby ballerina first pair of pointe shoes 🩰 #ballerina #pointe #shorts #pointeshoes #ballet
Shopping for a new pair of Crocs. #shorts #crocs #shoes #shoelife #kids #dickssportinggoods #why
Richmat adjustable bed remote reset/pairing
The Bluetooth device is ready to pair
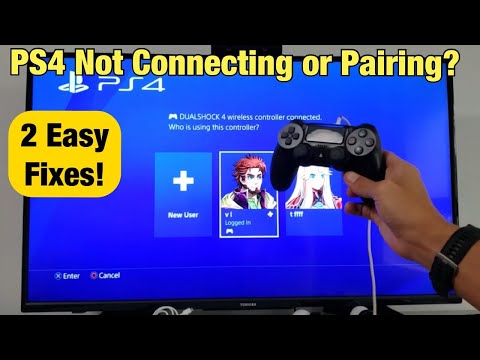
PS4 Controller Not Connecting or Pairing (Not Working)? 2 Easy Fixes
Get adaptable style on a budget with Pair Eyewear's affordable frames!
When You Need A Really, Really Big Pair Of Pliers! #Knipex #MrSubaru1387 #Shorts
How to set up your Echo Show | Amazon Alexa
Комментарии
 0:03:22
0:03:22
 0:00:15
0:00:15
 0:00:26
0:00:26
 0:00:23
0:00:23
 0:00:43
0:00:43
 0:02:47
0:02:47
 0:00:13
0:00:13
 0:04:11
0:04:11
 0:25:31
0:25:31
 0:01:53
0:01:53
 0:02:07
0:02:07
 0:02:26
0:02:26
 0:00:29
0:00:29
 0:02:03
0:02:03
 0:01:09
0:01:09
 0:00:30
0:00:30
 0:00:17
0:00:17
 0:00:14
0:00:14
 0:01:01
0:01:01
 0:00:13
0:00:13
 0:02:05
0:02:05
 0:00:29
0:00:29
 0:00:56
0:00:56
 0:01:25
0:01:25