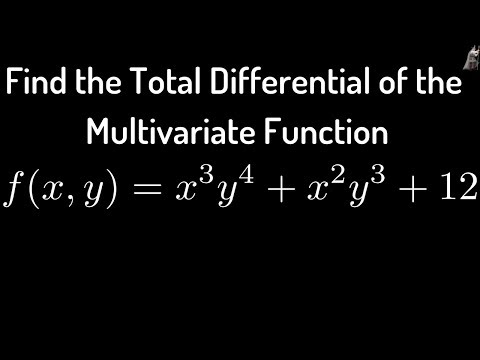
Multivariate calculus | Find Total Derivative (dy/dx) By Using Partial Derivatives | Ex 2.6 Q 42-45

PARTIAL DERIVATIVE. TOTAL DIFFERENTIAL. TOTAL DERIVATIVE
multivariable function:
A multivariable function is just a function whose input and/or output is made up of multiple numbers. In contrast, a function with single-number inputs and a single-number outputs is called a single-variable function.
For example, a function like f ( x , y ) = x 2 y f(x, y) = x^2 y f(x,y)=x2yf, left parenthesis, x, comma, y, right parenthesis, equals, x, squared, y, which has a two-variable input and a single-variable output, associates points in the x y xy xy -plane with points on the number line.

limit of multivariable function:
Along any line y=mx in the domain of the f(x,y), the limit is 0. However, along the path y=−sinx, which lies in the domain of f(x,y) for all x≠0, the limit does not exist. Since the limit is not the same along every path to (0,0), we say lim(x,y)→(0,0)sin(xy)x+y does not exist.

continuity of multivariable function:
We define continuity for functions of two variables in a similar way as we did for functions of one variable. Let a function f(x,y) be defined on an open disk B containing the point (x0,y0). f is continuous at (x0,y0) if lim(x,y)→(x0,y0)f(x,y)=f(x0,y0). f is continuous on B if f is continuous at all points in B.

multivariable functions and applications:
Multivariable calculus can be applied to analyze deterministic systems that have multiple degrees of freedom. Functions with independent variables corresponding to each of the degrees of freedom are often used to model these systems, and multivariable calculus provides tools for characterizing the system dynamics.