Limits of Multivariable Functions - Calculus 3

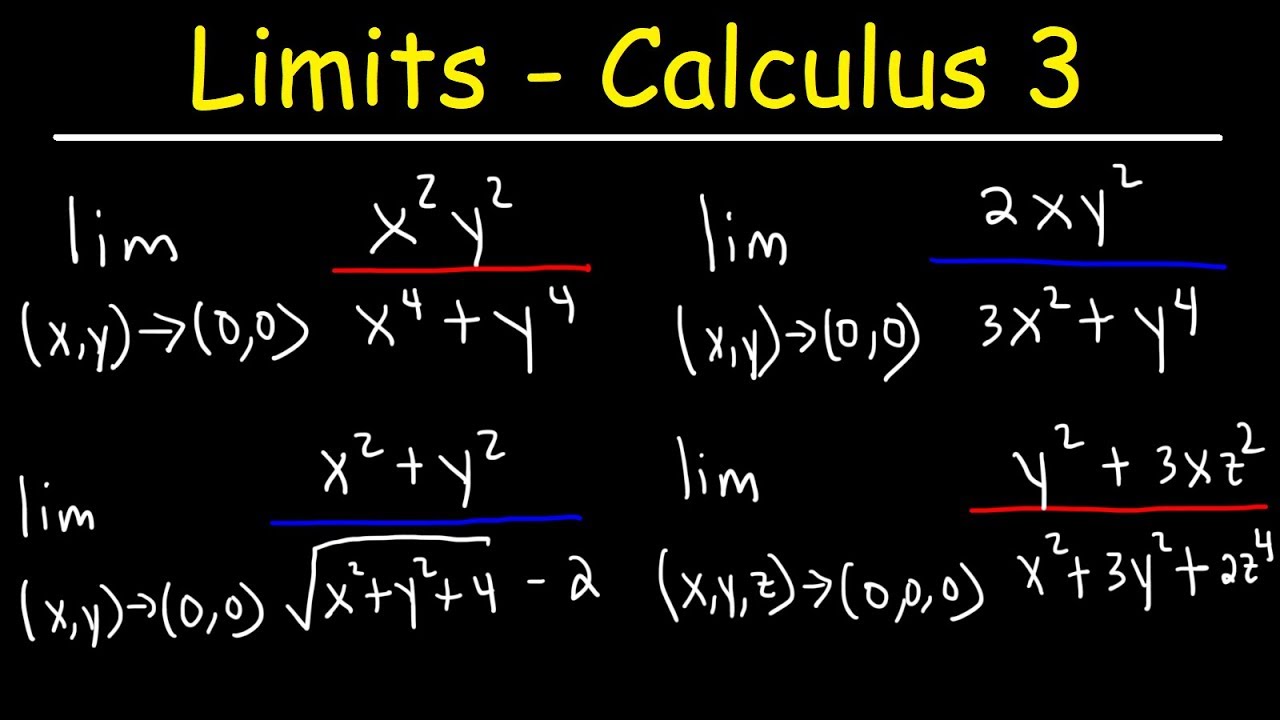
This Calculus 3 video tutorial explains how to evaluate limits of multivariable functions. It also explains how to determine if the limit does not exist.

Angle Between Two Planes:

Distance Between Point and Plane:

Chain Rule - Partial Derivatives:

Implicit Partial Differentiation:

________________________________
Directional Derivatives:

Limits of Multivariable Functions:

Double Integrals:

Local Extrema & Critical Points:

Absolute Extrema - Max & Min:

________________________________
Lagrange Multipliers:

Triple Integrals:

2nd Order - Differential Equations:

Undetermined Coefficients:

Variation of Parameters:

________________________________
Final Exams and Video Playlists:

Full-Length Videos and Worksheets:

[johnch]

Khan Academy gets constantly so much praise and they deserve it, but for someone looking to pass with 5 days of studying in the whole semester videos like this are what truly can help.

[janeh]

0:00 direct substitution
0:43 manipulating equation to allow direct substitution
1:53 proving the limit D.N.E.
4:15 proving the limit D.N.E.
6:59 direct substitution
7:35 proving the limit D.N.E.
12:40 manipulating equation to allow direct substitution
15:20 using parametric curves

[kimjongtrill]

Just came here to say I've watched all your Calc videos, don't know what I'd do without you. I'm on Calc 3 now so keep em coming!

[lidyasolomon]

Lmao pray for me...I havent paid attention all semester, but my final is tomorrow. I'm cramming with his videos. hahaha. This could be the first class I fail in my life. Jesus take the wheelll. I got an A in both Calc 1 and 2 so if I fail it would be a major disgrace for me and my GPA. I'm on the honors list, so hahaha pray for me.

[uke]

You are single handedly giving me my math credits towards my degree 😂

[samiabdullah]

16:43 last example the y -axis when z=0 and x=0 its equal 1over2 which not zero

[radityafajri]

I think in the last example, when we approach the function from the y-axis (which gives us x = 0 and y = 0), the result doesn't equal to zero. So, from that, we can conclude that the limit DNE.

[jeenagurungfuenjnsvuc]

If only i had a maths teacher like you before..
I won't t have been here today watching your videos.
But I am glad that i watched it.
You explained my whole degree in just these minutes.
Thank you so much. 💛 Your videos are worth watching...

[scottwitoff]

Is there a limit as to how many topics you can cover? Keep up the good work!

[SonGoku-wdmi]

Calc 3? im so far gone from calc 1 trying to find an answer from a test... good luck Uni students doing this.

[abumahraz]

Kudus to d create tutor on YouTube. I'm at d top of my class with d help of ur tutorials. Now in lvl3 & I'm missing ur tutorials on d courses I'm taking. But thanks so much for ur support sir.

[vinniesanchez]

I’m in Algebra 2 as a sophomore and I understand this. That’s how good this guy is at explaining math.

[quamos]

You are the reason why am still surviving calculus

[debasishkundu]

This is absolutely Why don't we have teachers like you in 😭😭😭😭 life would've gotten much 🥺

[georgesadler]

Professor Organic Chemistry Tutor, thank you for solving Limits of Multivariable Functions in Calculus Three/ Multivariable Calculus. In some cases, Polar Coordinates can also be used to evaluate Limits of Multivariable Functions in Multivariable Calculus. This is an error free video/lecture on YouTube TV with the Organic Chemistry Tutor.

[nikhilavishek]

I always wonder, why the channel name is The Organic Chemistry Tutor when a person who is teaching is actually a gem in mathematics.

[mentor__adriansyahariak]

16:36, I'm sorry but if I check with lim y->0 which is (x=z=0), i got 1/2, isn't it?

[ycvllim]

question about the last problem, is it okay to let x, z=0 and the value becomes y^2/2y^2 which keeps value 1/2 and can say it's limit dne?

[Max-cubw]

Just a question, for the last problem, approaching from the y-axis, leaving x=0 and z=0 wouldn't you get 1/2 which also proves the limit DNE?

[bjornvanderlande]

really good video thanks, just had a question. In the last example you stated that this problem couldnt be solved by travelling over a certain axis, but if we travel over the y axis this limit becomes y^2/2y^2 which is 1/2 which is different than going over the x or z axis so the limit doesnt exist. Or am I overlooking something?