Line Integral | Surface Integral | Mechanics | lecture 9 | BS physics | BSc | Calculus | ADS

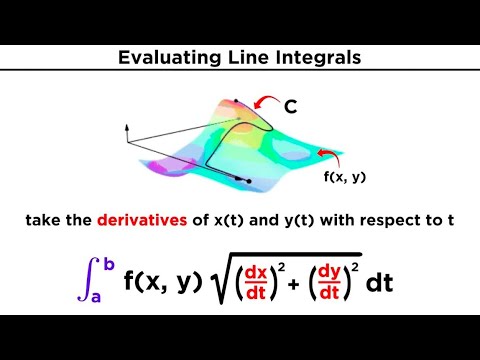
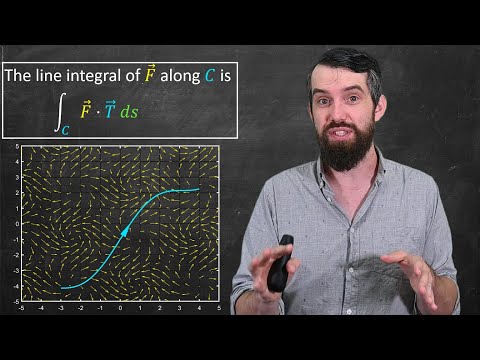
A line integral, also known as a path integral, is a concept in calculus and vector calculus that involves integrating a function along a specific path in a vector field. It's a way to calculate quantities such as work, circulation, or flux as you move along a curve. Imagine you're tracing a path through a vector field, and at each point, you're multiplying the field's value by a function and then adding up these products along the path. Line integrals have applications in physics, engineering, and various other fields to analyze how quantities change as you move along a particular path.

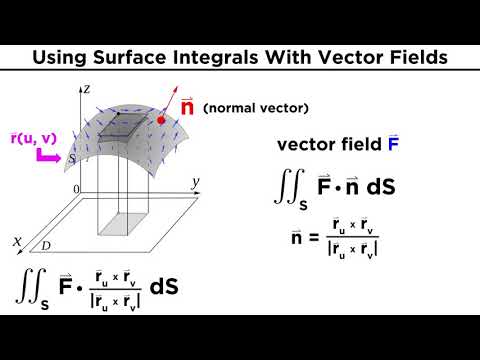
A surface integral is a fundamental concept in calculus and multivariable calculus that allows us to calculate various quantities over a two-dimensional surface in a three-dimensional space. Imagine you have a function defined on a surface, and you want to find the total effect of that function over the entire surface. It's like adding up the contributions of the function at every point on the surface. Surface integrals have applications in physics, engineering, and many other fields. They're used to compute quantities like flux, which measures how much a vector field "flows through" a surface, or to calculate the total mass or charge distribution on a given surface.

Title: "Line Integrals and Surface Integrals: Navigating the Mathematics of Curves and Surfaces"

Description:

Welcome to [physics ka safar]! In this educational video, we're diving deep into the world of calculus and vector calculus to explore two important mathematical tools: line integrals and surface integrals. Join us as we demystify these concepts, understand their significance, and showcase their applications in physics, engineering, and more.

In this video, we'll cover the following key aspects of line integrals and surface integrals:

Introduction to Integration: We'll start with an overview of integration and why it's crucial for understanding quantities that vary over curves and surfaces.

Line Integrals: Dive into line integrals, which allow us to calculate quantities along curves in three-dimensional space. Understand their mathematical formulation and physical interpretation.

Applications of Line Integrals: Explore real-world applications of line integrals, including work done by a force along a path, circulation in fluid dynamics, and more.

Surface Integrals: Transition to surface integrals, which enable us to calculate quantities over surfaces. Learn how to set up and evaluate surface integrals.

Physical Significance: Discover the physical significance of surface integrals and their applications in fields like flux in electromagnetism, mass flow rates in fluid dynamics, and heat transfer.

Calculation and Examples: Work through practical examples to illustrate how to calculate line integrals and surface integrals, solving real-world problems.

By the end of this video, you'll have a solid understanding of line integrals and surface integrals, their significance, and how they help us analyze quantities over curves and surfaces in three-dimensional space.

Don't forget to hit the like button, subscribe to [physics ka safar], and turn on notifications to stay updated with our educational content. If you have any questions or topics you'd like us to cover in future videos, please leave a comment below.

Join us on this enlightening journey through the world of line integrals and surface integrals, and let's navigate the mathematics of curves and surfaces together!

[AliHamzaAliHamza-jxie]

Sir aap hanstay bahut ha wasy ya aak acha way ha bacho KO understand kervane ka.Thanks sir

[aymanshah-ehts]

The way you explains complex concepts with such clarity and enthusiasm is truly remarkable.Definitely the best physics teacher out there! 🌟🔬 #PhysicsGenius❤😊

[mehwishshahzadi]

Thank you so much for such informative video ❤❤

[AhtishamAli-eifj]

Sir jo topic krvaya kren unka ka aik aik sath mn numerical b chakha dya kren