filmov
tv
Surface Integrals // Formulas & Applications // Vector Calculus
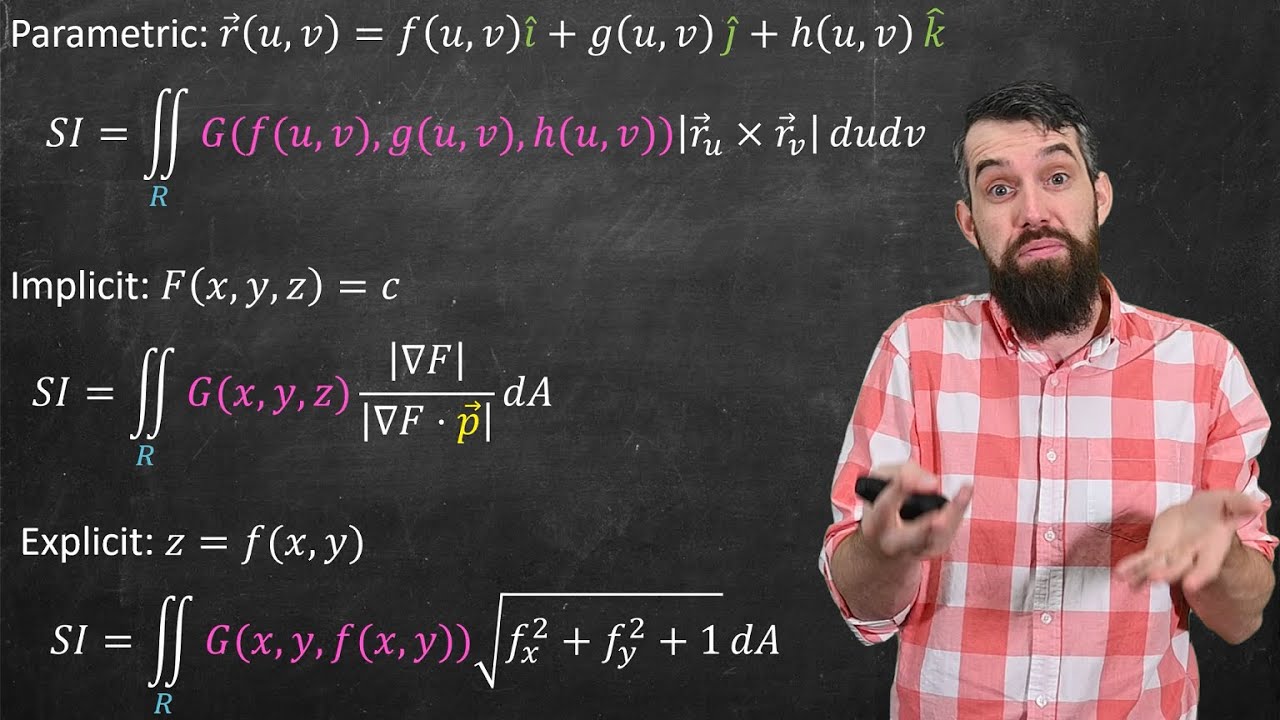
Показать описание
In this video we come up formulas for surface integrals, which are when we accumulate the values of a scalar function over a surface. This is analogous to a line integral over a curve. As surfaces can be described implicitly, explicitly or parametrically - we come up with a surface area formula for each type. One application is finding the total mass of a surface with variable mass density, and a second is computing the average temperature on the surface of the earth.
0:00 Surface Area Formulas
1:50 Surface Integral Formulas
3:55 Application #1: Mass
4:30 Application #2 Averages
MY VECTOR CALCULUS PLAYLIST:
OTHER COURSE PLAYLISTS:
OTHER PLAYLISTS:
► Learning Math Series
►Cool Math Series:
BECOME A MEMBER:
MATH BOOKS & MERCH I LOVE:
SOCIALS:
0:00 Surface Area Formulas
1:50 Surface Integral Formulas
3:55 Application #1: Mass
4:30 Application #2 Averages
MY VECTOR CALCULUS PLAYLIST:
OTHER COURSE PLAYLISTS:
OTHER PLAYLISTS:
► Learning Math Series
►Cool Math Series:
BECOME A MEMBER:
MATH BOOKS & MERCH I LOVE:
SOCIALS:
Surface Integrals // Formulas & Applications // Vector Calculus
Evaluating Surface Integrals
Describing Surfaces Explicitly, Implicitly & Parametrically // Vector Calculus
Understanding Surface integrals
What is Double integral? Triple integrals? Line & Surface integral? Volume integral? #SoME2
Derivation of surface integaral formula.
23: Scalar and Vector Field Surface Integrals - Valuable Vector Calculus
Introduction to the surface integral | Multivariable Calculus | Khan Academy
GATE ECE - 51 Most Repeated Topics | Don't Miss to Study These !! #gate2025 #gatepreparation
Surface Area of Revolution By Integration Explained, Calculus Problems, Integral Formula, Examples
Surface Integrals
How to Evaluate a Surface Integral
Surface Integrals
INTEGRATION BY PARTS (EASIEST METHOD)
Surface Integrals
The Surface Area formula for Parametric Surfaces // Vector Calculus
Example of calculating a surface integral part 1 | Multivariable Calculus | Khan Academy
What is a Surface Integral?
The Secret Behind Integration: Unveiling Riemann Sums! (Animated)#maths
surface integral, example 2 (KristaKingMath)
Surface integral definition and formula/ surface integration formulas
Computing the Flux Across a Surface // Vector Calculus
Multivariable Calculus | Two surface integrals over vector fields.
Simple Integral vs Double Integral #calculus #maths
Комментарии
 0:08:18
0:08:18
 0:12:24
0:12:24
 0:11:05
0:11:05
 0:01:00
0:01:00
 0:05:59
0:05:59
 0:05:01
0:05:01
 0:27:51
0:27:51
 0:22:14
0:22:14
 0:27:15
0:27:15
 0:30:36
0:30:36
 0:34:49
0:34:49
 0:10:26
0:10:26
 0:35:35
0:35:35
 0:00:22
0:00:22
 0:19:45
0:19:45
 0:09:26
0:09:26
 0:10:45
0:10:45
 0:15:06
0:15:06
 0:00:29
0:00:29
 0:14:09
0:14:09
 0:04:05
0:04:05
 0:08:09
0:08:09
 0:20:12
0:20:12
 0:00:50
0:00:50