One-to-One and Onto Linear Transformations | Linear Algebra

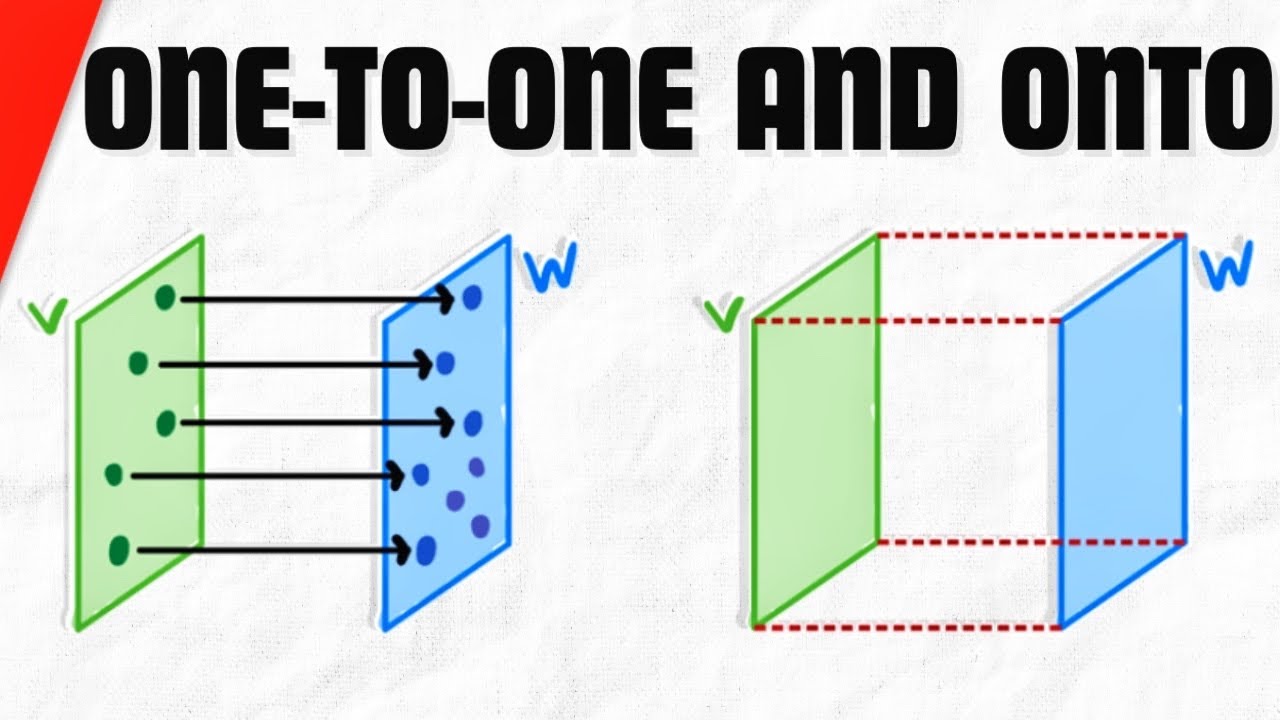
preview_player
Показать описание
We introduce one to one and onto linear transformations. These are properties you may be familiar with, one to one is also called "injective" and onto is called "surjective". These properties are important, and as we'll see in later lectures, many nice things can be said about linear transformations with either or both of these properties. We'll show several examples of linear transformations that are onto or one-to-one or neither. We'll prove a theorem establishing an equivalence between a linear transformation being one-to-one, being onto, and having the zero space as its kernel (provided the domain and codomain have the same finite dimension). #linearalgebra

Join Wrath of Math to get exclusive videos, lecture notes, and more:

0:00 Intro
0:32 Definition of One-to-One and Onto
1:19 Visualize the Definitions
3:14 Zero Kernel is same as One-to-One
6:03 Rotation is One to One and Onto
6:46 Projection onto xy Plane is Neither One to One nor Onto
7:55 Transpose is One to One and Onto
9:13 Zero Transformation is Neither One to One nor Onto
10:01 One to One but not Onto
11:31 An Onto Equivalence
15:14 Conclusion

★DONATE★

Outro music by Ben Watts and is available for channel members.

Follow Wrath of Math on...
  1. Wrath of Math
  2. wrath of math
  3. one to one and onto
  4. one to one linear transformations
  5. onto linear transformations
  6. examples of one to one linear transformations
Рекомендации по теме
Linear Algebra: Checking 0:06:29

Linear Algebra: Checking if a transformation is one-to-one and onto

Determine if a 0:04:13

Determine if a Linear Transformation is One-to-One and/or Onto (R3 to R2)

Is a linear 0:06:09

Is a linear transformation one to one? Onto? An isomorphism?

Surjective (onto) and 0:09:32

Surjective (onto) and injective (one-to-one) functions | Linear Algebra | Khan Academy

66. One-to-One and 0:13:36

66. One-to-One and Onto Linear Transformations

Determining whether a 0:25:51

Determining whether a transformation is onto | Linear Algebra | Khan Academy

One to one, 0:07:23

One to one, onto, matrix

One-to-one and Onto 0:20:47

One-to-one and Onto Linear Transformations

One-to-One and Onto 0:16:03

One-to-One and Onto Linear Transformations | Linear Algebra

One-to-one, Onto, and 0:09:30

One-to-one, Onto, and the Big Theorem Part II

One-to-One and Onto 0:18:43

One-to-One and Onto Linear Transformations

One to One 0:18:19

One to One and Onto Transformations | Linear Transformation | Engineering Mathematics

Section 3.2: One-to-one 0:13:24

Section 3.2: One-to-one and Onto Transformations

Linear Transformation - 0:17:46

Linear Transformation - One One Transformation - Onto Transformation - One One & Onto Transforma...

One-to-One and Onto 0:50:02

One-to-One and Onto Transformations

Linear Transformations: Onto 0:08:24

Linear Transformations: Onto

Relating invertibility to 0:06:31

Relating invertibility to being onto and one-to-one | Linear Algebra | Khan Academy

Linear Algebra Example 0:05:20

Linear Algebra Example Problems - Onto Linear Transformations

Linear Algebra 2 0:01:41

Linear Algebra 2 - 2.2.2 - One-to-one and Onto Linear Transformations (Video 3)

Linear Algebra 2 0:06:11

Linear Algebra 2 - 2.2.2 - One-to-one and Onto Linear Transformations (Video 1)

Linear Algebra 2 0:05:59

Linear Algebra 2 - 2.2.2 - One-to-one and Onto Linear Transformations (Video 5)

Is the linear 0:05:44

Is the linear transformation one-to-one/onto?

Linear transformations and 0:10:59

Linear transformations and matrices | Chapter 3, Essence of linear algebra

Comparing One-to-One and 0:06:03

Comparing One-to-One and Onto Matrix Transformations

Комментарии
Автор

Hello wrath of math, 😊

I hope you’re doing well. 🌟 I know you have a strong background in mathematics and a deep, logical way of thinking, which is something I really admire about you. 📚✏

I’ve been thinking about a topic that has always intrigued me, and I wanted to share it with you. 🌍 It’s the religion of Islam. 🕌

What fascinates me about Islam is the close connection between science and faith. 🔄 The Quran contains many verses that refer to science and nature, and some scholars find references to mathematical and scientific principles within it. 📖✨

I’d like to invite you, out of our mutual respect for reason and logic, to explore some texts or books that discuss Islam from a scientific or philosophical perspective. 💡📘

I believe that you might find this aspect interesting. And of course, I’m here to discuss any questions or thoughts you might have during this journey. 🤝

Wishing you a great day, and I look forward to hearing your thoughts. 🌞

Youcef-lx
Автор

Wrath of Math, You're fantastic! Let's be friends and have fun!

IOSALive