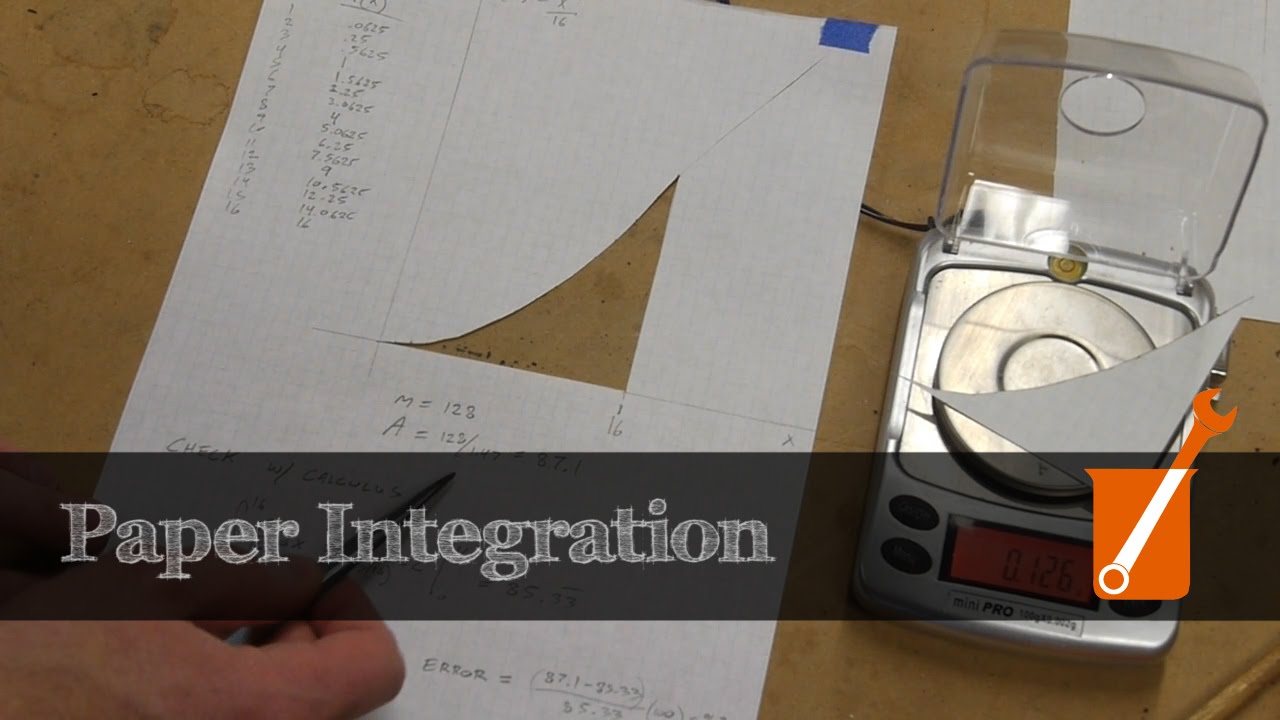
Mathematical integration without calculus

I show how to convert an area measurement problem into a mass measurement problem that is easier to solve. In general, this idea of converting measurement problems into different spaces is very powerful, and may prove useful in the future.

[alahalla]

Gotta ask my professor if i can bring a balance to the next exam.

[markbell]

Good examples.  In about 1981 in my geochemistry class the Professor gave use a problem to work-out the partial pressure of oxygen (i.e. oxygen fugacity) of some high temp/press mineral reaction.  The result was an insoluble polynomial (at least by me) that graphed as a decaying curve asymptotically approaching y=0.  Anyway, most folks plotted the function on 8.5x11 notebook paper and weighed the area as you showed.  Well for me, propagated by my ADHD, 8.5x11 just would not do.  So, I bought a 100 foot long piece of butcher paper and using calipers and tape measure made my 100 foot area under curve.  As I recall, my result was not the most accurate, but needless to say it was the most impressive.  Cheers, Mark

[karlw]

Perhaps the most important application of methods like this is in education. Kids' brains are different and a certain portion of the population will comprehend concepts more readily using physical representations than by using equations only. Presenting material in a variety of ways including physical examples helps to keep more students interested in math and show people who otherwise might quit that there is a science of applied math called engineering...

[freggo]

We used this method back in the 70s on result plots from our Gas Chromatographs. Perkin Elmer had just released the first electronic integrator but at over $10, 000 per ( we had about a dozend GCs in the lab) this was out of the budget. So either manual triangulation or Scissors and a sensitive lab scale where the tools of the trade :-) Tks for waking up some good memories!

[matthewjames]

Very clever. Although I suspect you increased your error in your M/A constant by writing pencil on the sheets of paper. Since there is roughly the same amount of graphite on all sheets of paper, this will increase the M/A ratio for smaller sheets - which is exactly what you notice for the 5x5 sheet. Still very clever. I enjoyed it :)

[MrPoffersher]

You sir are a true nerd, in the best possible way.

[electrodacus]

The best example of this type that comes to mind is volume calculation by submerging complex shaped objects in water.

[pocket]

I think you made the best case for it at the end. The reason that calculus is often useless to us in real life is because we have only collected real datum points, which can't possibly reveal to us their overarching pattern (function). Two points define a line, and three a plane, but even 250 samples that look like they sit on a certain curve don't prove with certainty that we have the function we're looking for. Rare is the case where we already have the actual function.


 I spend my time arguing for the usefulness of mathematics, but only to a certain point; although I find it wondrous and beautiful beyond a certain level of abstraction,  to me, that place fits more in the realm of philosophy than it does as a useful tool. Calculus sits on this threshold.


 Mathematics goes to places that we should use caution while treading on. We have no reason to suppose that our system, while so perfect in its description of the universe that we see, will work in every possible extension. We assume multiple dimensions as we proceed, and we invent wild physical theory- and such is fascinating- but we must remember that it is all built on the assumption that our mathematics is a valid tool for every approach. The part that scares me is that it can produce apparent contradictions using only algebra, so count me as a skeptic when I hear those wild speculations about string theory on television. Of course, all of this is probably just my own ignorance making this rant, but I still like to see a problem solved with geometry- it just feels so real;)

[gaius_enceladus]

Nice!

This reminds me of the video here on Youtube about the old mechanical gunnery-control systems on battleships that solved gunnery target problems using all kinds of cams, disks and gizmos. Very elegant and cool.

[BrainBlister]

Another nice video. brings back old memories. we used the cut an weigh technique in the lab with gas and liquid chromatographs and chart recorders. the paper was fairly expensive since it needed to be very uniform for weighing. you could get very precise replicates being careful with your cutting. Not a very enjoyable task when you had dozens of samples to run with several peaks to cut out per run.

[JamesNewton]

Excellent video on solving problems by converting them into a different space. Or, as I like to call it, thinking outside the box.

[paulgrosse]

This was the method of choice for finding the area under peaks in chromatography, in the time before mechanical (disc) integrators (which was before it all went electronic).

Using this very method, I was able to demonstrate that a chromatograph of TCPP isomers produced by ICI in Belgium (who were claiming that their chromatogram showed that their product had a superior isomer ratio) was produced by overloading the amplifier which gave the wrong results - they had assumed that they could get the main peaks off scale and it would all still be counted, ie, not clipped by the amplifier.

I photocopied their chromatogram onto some uniform paper, cut it out and weighed the peaks (on a four figure balance (down to 0.1mg)) and came to the same result as they did, demonstrating that their results had the main peak clipped and therefore their real isomer ratio was not as good as they claimed. (Later on, a sample of their product put through our chromatograph showed that it was worse than ours - based on their definition of what was better or what was worse)

[mikeselectricstuff]

How about a completely mechanical automatic integrator - roll of paper, cutting blade (or laser), balance....

[stickyfox]

When I was a kid in Blacksburg VA, my best friend's father, a VT biologist, used an analytical balance to integrate graphed data because it was quicker and simpler than the available electronic means of doing so.

[christheother]

Years ago when designing paper gliders I weighed many sheets of paper to allow calculating a reasonable value for weight per square unit. When calculating wing loading, I would often approximate area of a given wing in calculations too... but here I see you could use a delicate scale to calculate area quickly without all the numerical integration.

[katelikesrectangles]

As a programer, I occasionally find if a concept is difficult to express in one language, sometimes it makes more sense to invent a language where that concept is very natural to express. Then all I need to do is implement that new language. So I've converted the problem from one of expressibility into interpretation.

[Reliquancy]

You could find an approximation of pi by weighing a circle of paper and working backwards from the measured area, dividing out the radius ^2

[litany]

Moving problems into a domain you are expert in really simplifies things when it is possible. I liked the usage for the micro scale. I suspect thinner paper would improve accuracy even higher.

[the_eternal_student]

I am glad you build on history. This reminds me of the numberphile videos about Archimedes and the voulme of a sphere and a parabola.

[NinjaOnANinja]

2:42
My heart sank a little because that was the "OK" you say at the end of your videos.

In my head I instantly was like, WAIT! ITS OVER!?  WHAT!?!?

But you kept going and I checked the remaining time.

All good!  All good!