filmov
tv
Volume of a Pyramid | Animated Visual Representation
Показать описание
Volume of a Pyramid
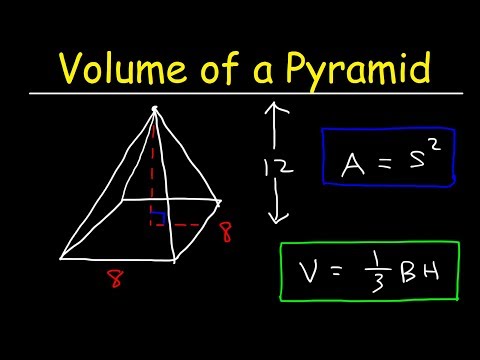
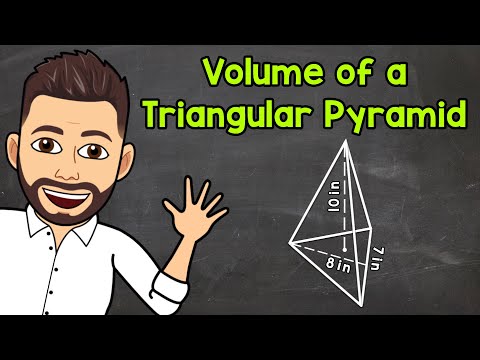
The volume of pyramid is space occupied by it (or) it is defined as the number of unit cubes that can be fit into it. A pyramid is a polyhedron as its faces are made up of polygons. There are different types of pyramids such as a triangular pyramid, square pyramid, rectangular pyramid, pentagonal pyramid, etc that are named after their base. i.e., if the base of a pyramid is a square, it is called a square pyramid. All the side faces of a pyramid are triangles where one side of each triangle merges with a side of the base. Let us explore more about the volume of pyramid along with its formula, its proof, and a few solved examples.
Formula of Volume of Pyramid
Let us consider a pyramid and prism each of which has a base area 'B' and height 'h'. We know that the volume of a prism is obtained by multiplying its base by its height. i.e., the volume of the prism is Bh. In the earlier section, we have seen that the volume of pyramid is one-third of the volume of the corresponding prism (i.e., their bases and heights are congruent).
Thus,
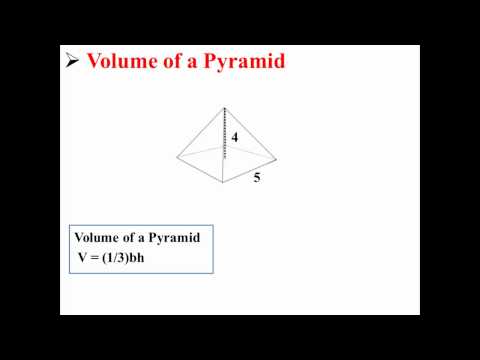
Volume of pyramid = (1/3) (Bh), where
B = Area of the base of the pyramidh = Height of the pyramid (which is also called "altitude")
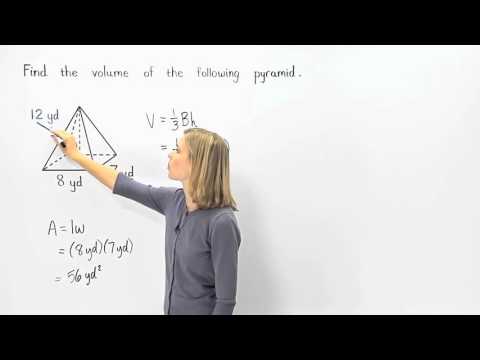
Volume Formulas of Different Types of Pyramids
From the earlier section, we have learned that the volume of a pyramid is (1/3) × (area of the base) × (height of the pyramid). Thus, to calculate the volume of a pyramid, we can use the areas of polygons formulas (as we know that the base of a pyramid is a polygon) to calculate the area of the base, and then by simply applying the above formula, we can calculate the area of the base.
#MathAnimation #ScienceAnimation #VolumeOfPyramid #Pyramid #MathsMagic #MathIsCool #MathTricks #MathArt
#JEEPreparation #JEEExam #JEE2022 #IITJEE #JEEMains #JEEAdvanced
#ChampionsAcademy #AcademicSystem #Coaching #Learning #Online #JEE #NEET #Physics #Chemistry #Biology #CBSE #ICSE #EasyLearning #LearningMadeEasy #OnlineLearning #ScienceCoaching
The volume of pyramid is space occupied by it (or) it is defined as the number of unit cubes that can be fit into it. A pyramid is a polyhedron as its faces are made up of polygons. There are different types of pyramids such as a triangular pyramid, square pyramid, rectangular pyramid, pentagonal pyramid, etc that are named after their base. i.e., if the base of a pyramid is a square, it is called a square pyramid. All the side faces of a pyramid are triangles where one side of each triangle merges with a side of the base. Let us explore more about the volume of pyramid along with its formula, its proof, and a few solved examples.
Formula of Volume of Pyramid
Let us consider a pyramid and prism each of which has a base area 'B' and height 'h'. We know that the volume of a prism is obtained by multiplying its base by its height. i.e., the volume of the prism is Bh. In the earlier section, we have seen that the volume of pyramid is one-third of the volume of the corresponding prism (i.e., their bases and heights are congruent).
Thus,
Volume of pyramid = (1/3) (Bh), where
B = Area of the base of the pyramidh = Height of the pyramid (which is also called "altitude")
Volume Formulas of Different Types of Pyramids
From the earlier section, we have learned that the volume of a pyramid is (1/3) × (area of the base) × (height of the pyramid). Thus, to calculate the volume of a pyramid, we can use the areas of polygons formulas (as we know that the base of a pyramid is a polygon) to calculate the area of the base, and then by simply applying the above formula, we can calculate the area of the base.
#MathAnimation #ScienceAnimation #VolumeOfPyramid #Pyramid #MathsMagic #MathIsCool #MathTricks #MathArt
#JEEPreparation #JEEExam #JEE2022 #IITJEE #JEEMains #JEEAdvanced
#ChampionsAcademy #AcademicSystem #Coaching #Learning #Online #JEE #NEET #Physics #Chemistry #Biology #CBSE #ICSE #EasyLearning #LearningMadeEasy #OnlineLearning #ScienceCoaching
0:01:50
0:07:44
0:01:52
0:01:59
0:01:56
0:02:06
0:02:11
0:04:16
0:02:14
0:08:29
0:02:31
0:29:41
0:00:40
0:04:52
0:04:24
0:00:28
0:03:25
0:39:50
0:09:09
0:08:43
0:05:30
0:12:36
0:00:49
0:04:17