filmov
tv
Bijective Functions and Why They're Important | Bijections, Bijective Proof, Functions and Relations
Показать описание
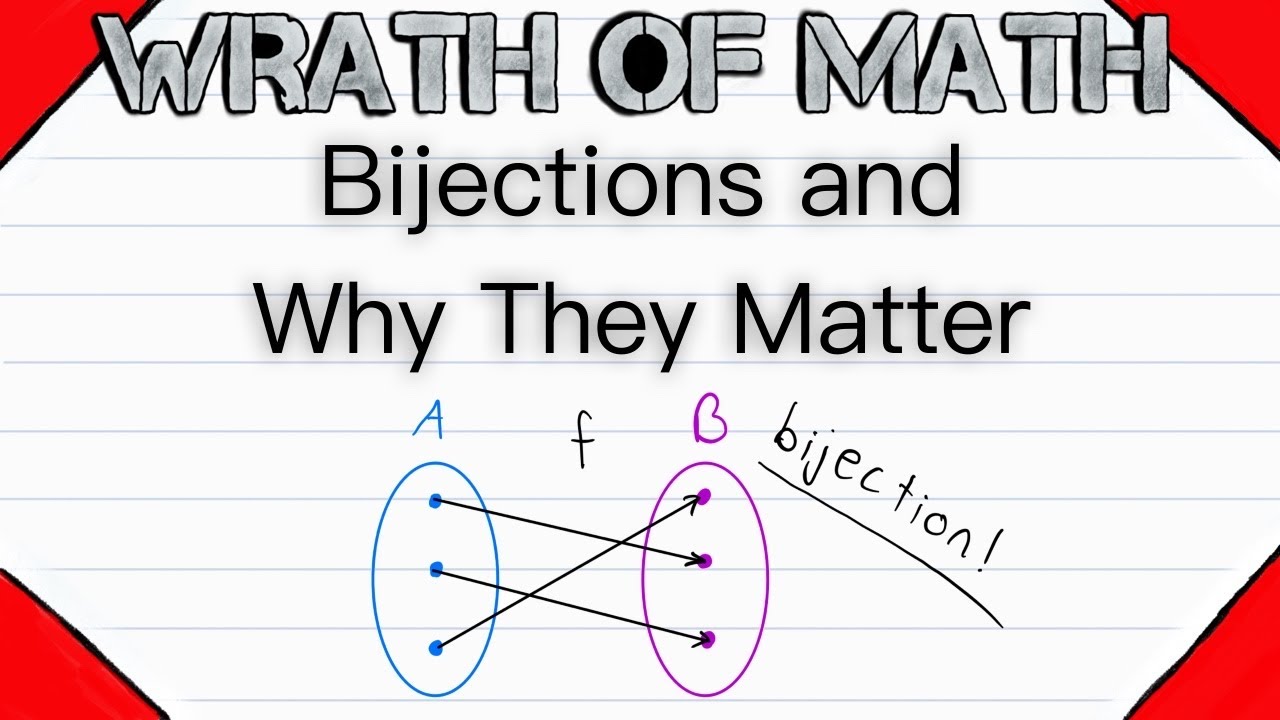
What are bijective functions and why should we care about them? We'll be going over bijections, examples, proofs, and non-examples in today's video math lesson!
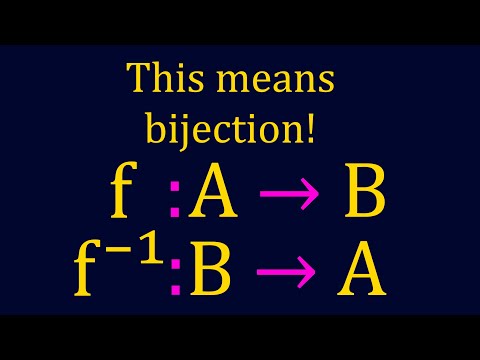
A bijection is a function that is both injective and surjective. We mention two very important things about bijections in the lesson. First, suppose f is a function from A to B and f is injective and surjective. Thus, f is bijective. Then, we can define an inverse function from B to A that takes the images of f and sends them back to their respective pre-images. This can only be done with a bijective function, which is very important!
However, there is a lot of possibility for nuance in the definition of inverse functions (we don't give a formal definition in this lesson), and while only a bijective function can have an inverse like we described, other types of functions can have other types of inverses that follow less strict definitions. This lesson is not meant to be an in-depth presentation of inverse functions, so I hope you'll look forward to learning more about them.
Here is a definition for you if you are interested: Let F be a function from A to B. Then a function, G, that maps B to A, is an inverse function of F if and only if g(f(a)) = a for all a in A and f(g(b)) = b for all b in B.
If you need a recap on injective and surjective functions, check out my lessons on them!
SOLUTION TO PRACTICE PROBLEM:
We want to show that the integers (Z) have the same cardinality as the odd numbers (we'll call the set of odd numbers S). So we want to find a bijection from one to the other; Z to X or X to Z. A good place to start, as we did with the example in the video, is with the definition of an odd number. An odd number is a number equal to 2k + 1 for some integer k. So if we define a function f from Z to X as f(z) = 2z + 1, that should work out! We just need to prove it.
First, we prove f is injective. Take z, w in Z and suppose f(z) = f(w). Then 2z + 1 = 2w + 1. Thus, 2z = 2w and z = w. So f is injective.
Proving surjectivity is even easier. Take x in X. By definition of X, x is odd and therefore there exists an integer k such that 2k + 1 = x. Then, f(k) = 2k + 1 = x. Thus, f is surjective. Therefore, since f is injective and surjective, f is bijective so |Z| = |X|.
I hope you find this video helpful, and be sure to ask any questions down in the comments!
********************************************************************
The outro music is by a favorite musician of mine named Vallow, who, upon my request, kindly gave me permission to use his music in my outros. I usually put my own music in the outros, but I love Vallow's music, and wanted to share it with those of you watching. Please check out all of his wonderful work.
********************************************************************
+WRATH OF MATH+
Follow Wrath of Math on...
A bijection is a function that is both injective and surjective. We mention two very important things about bijections in the lesson. First, suppose f is a function from A to B and f is injective and surjective. Thus, f is bijective. Then, we can define an inverse function from B to A that takes the images of f and sends them back to their respective pre-images. This can only be done with a bijective function, which is very important!
However, there is a lot of possibility for nuance in the definition of inverse functions (we don't give a formal definition in this lesson), and while only a bijective function can have an inverse like we described, other types of functions can have other types of inverses that follow less strict definitions. This lesson is not meant to be an in-depth presentation of inverse functions, so I hope you'll look forward to learning more about them.
Here is a definition for you if you are interested: Let F be a function from A to B. Then a function, G, that maps B to A, is an inverse function of F if and only if g(f(a)) = a for all a in A and f(g(b)) = b for all b in B.
If you need a recap on injective and surjective functions, check out my lessons on them!
SOLUTION TO PRACTICE PROBLEM:
We want to show that the integers (Z) have the same cardinality as the odd numbers (we'll call the set of odd numbers S). So we want to find a bijection from one to the other; Z to X or X to Z. A good place to start, as we did with the example in the video, is with the definition of an odd number. An odd number is a number equal to 2k + 1 for some integer k. So if we define a function f from Z to X as f(z) = 2z + 1, that should work out! We just need to prove it.
First, we prove f is injective. Take z, w in Z and suppose f(z) = f(w). Then 2z + 1 = 2w + 1. Thus, 2z = 2w and z = w. So f is injective.
Proving surjectivity is even easier. Take x in X. By definition of X, x is odd and therefore there exists an integer k such that 2k + 1 = x. Then, f(k) = 2k + 1 = x. Thus, f is surjective. Therefore, since f is injective and surjective, f is bijective so |Z| = |X|.
I hope you find this video helpful, and be sure to ask any questions down in the comments!
********************************************************************
The outro music is by a favorite musician of mine named Vallow, who, upon my request, kindly gave me permission to use his music in my outros. I usually put my own music in the outros, but I love Vallow's music, and wanted to share it with those of you watching. Please check out all of his wonderful work.
********************************************************************
+WRATH OF MATH+
Follow Wrath of Math on...
Комментарии
 0:10:10
0:10:10
 0:17:15
0:17:15
 0:11:18
0:11:18
 0:02:22
0:02:22
 0:09:32
0:09:32
![[Proof] Function is](https://i.ytimg.com/vi/oTub-N4uopw/hqdefault.jpg) 0:08:50
0:08:50
 0:05:25
0:05:25
 0:03:04
0:03:04
 0:08:02
0:08:02
 0:02:05
0:02:05
 0:09:49
0:09:49
 0:17:25
0:17:25
 0:00:51
0:00:51
 0:07:14
0:07:14
 0:03:22
0:03:22
![[Discrete Mathematics] Injective,](https://i.ytimg.com/vi/PpA4LWPh40M/hqdefault.jpg) 0:15:01
0:15:01
 0:10:15
0:10:15
 0:11:44
0:11:44
 0:11:40
0:11:40
 0:01:10
0:01:10
 0:12:38
0:12:38
 0:05:25
0:05:25
 0:08:53
0:08:53
 0:08:06
0:08:06