filmov
tv
Bijective Functions and the Continuum Hypothesis

Показать описание
This video is largely about bijective functions. Specifically why bijective functions have inverses, why bijective functions can be used to show two sets are the same size and how the continuum hypothesis can be written as a statement about bijections.
Chapters:
0:00 - Introduction
01:02 - Definitions
03:32 - Inverses
05:28 - Cardinality
08:29 - Continuum Hypothesis
The product links below are Amazon affiliate links. If you buy certain products on Amazon soon after clicking them, I may receive a commission. The price is the same for you, but it does help to support the channel.
In-video references
1. An Introduction to Set Theory (William A. R. Weiss) - Available online for free.
3. Basic Real Analysis (Anthony W. Knapp) - Available online for free
5. Cohen, P.J., 1964. The independence of the continuum hypothesis, II. Proceedings of the National Academy of Sciences of the United States of America, 51(1), p.105.
FAQ : How do you make these animations?
Animations are made in Apple Keynote which has lots of functionality for animating shapes, lines, curves and text (as well as really good LaTeX). Editing and voiceover work in DaVinci Resolve.
Supporting the Channel.
If you would like to support me in making free mathematics tutorials then you can make a small donation over at
Thank you so much, I hope you find the content useful.
Chapters:
0:00 - Introduction
01:02 - Definitions
03:32 - Inverses
05:28 - Cardinality
08:29 - Continuum Hypothesis
The product links below are Amazon affiliate links. If you buy certain products on Amazon soon after clicking them, I may receive a commission. The price is the same for you, but it does help to support the channel.
In-video references
1. An Introduction to Set Theory (William A. R. Weiss) - Available online for free.
3. Basic Real Analysis (Anthony W. Knapp) - Available online for free
5. Cohen, P.J., 1964. The independence of the continuum hypothesis, II. Proceedings of the National Academy of Sciences of the United States of America, 51(1), p.105.
FAQ : How do you make these animations?
Animations are made in Apple Keynote which has lots of functionality for animating shapes, lines, curves and text (as well as really good LaTeX). Editing and voiceover work in DaVinci Resolve.
Supporting the Channel.
If you would like to support me in making free mathematics tutorials then you can make a small donation over at
Thank you so much, I hope you find the content useful.
Комментарии
 0:09:49
0:09:49
 0:22:48
0:22:48
 0:04:36
0:04:36
 0:01:26
0:01:26
 0:40:53
0:40:53
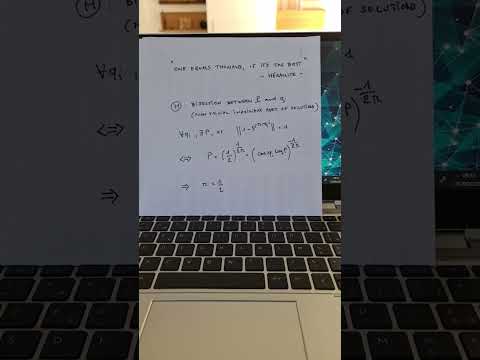 0:00:16
0:00:16
 1:50:36
1:50:36
 0:07:13
0:07:13
 0:14:43
0:14:43
 0:49:54
0:49:54
 0:11:43
0:11:43
 0:01:26
0:01:26
 0:05:58
0:05:58
 1:10:40
1:10:40
 0:00:58
0:00:58
 0:24:44
0:24:44
 0:06:40
0:06:40
 1:04:28
1:04:28
 0:24:28
0:24:28
 0:19:37
0:19:37
 0:11:23
0:11:23
 0:34:38
0:34:38
 0:18:33
0:18:33
 0:01:42
0:01:42