filmov
tv
Complex Numbers as Matrices
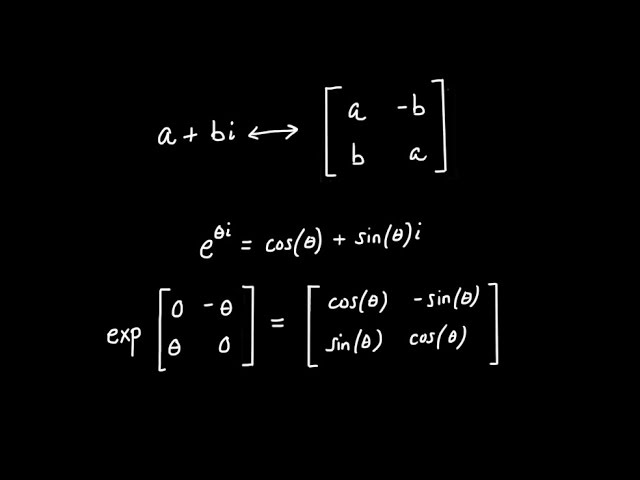
Показать описание
In this video, we'll learn how to view a complex number as a 2x2 matrix with a special form. We'll also see that there is a matrix version for the number 1 and a matrix representation for the imaginary unit, i. Furthermore, the matrix representation for i has the defining feature of the imaginary unit in that it squares to -1. We'll also explore other features of complex numbers that tie into matrix operations, such as the determinant and transpose. Euler's formula even has a sensible interpretation in matrix algebra and we'll see an example of a matrix exponential.
Complex numbers as matrices | Representation theory episode 1
Complex Numbers as Matrices
Complex, Hermitian, and Unitary Matrices
Complex Numbers As Matrices
Complex Matrices
Why Complex Numbers as 2X2 Matrices???
The strange cousin of the complex numbers -- the dual numbers.
Necessity of complex numbers
Types of Matrices - Part 2
Addition Formulae using Matrices?! - Complex Numbers, but Different [ Bonus 1 ]
Matrices 12: Where do Complex Numbers come from?
How (and why) to raise e to the power of a matrix | DE6
Maybe Complex Numbers are Just Matrices | Math, but Extra
Solving 3x3 Complex Matrix using Casio Calculator (Second Method)
Quaternions and 3d rotation, explained interactively
Imaginary Numbers Are Real [Part 1: Introduction]
Matrix Inverse over the Complex Numbers
using calculator to solve complex numbers equations (matrix creamer's rule)
Euler's Formula Beyond Complex Numbers
Complex Numbers as Rotation Matrices
Complex Matrices ( An intuitive visualization )
Intro to Matrices
COMPLEX Eigenvalues, Eigenvectors & Diagonalization **full example**
14C Matrices with Complex Number Elements
Комментарии
 0:19:17
0:19:17
 0:16:25
0:16:25
 0:09:00
0:09:00
 0:09:21
0:09:21
 0:13:02
0:13:02
 0:11:51
0:11:51
 0:19:14
0:19:14
 0:07:39
0:07:39
 0:07:33
0:07:33
 0:19:45
0:19:45
 0:13:12
0:13:12
 0:27:07
0:27:07
 0:05:12
0:05:12
 0:16:50
0:16:50
 0:05:59
0:05:59
 0:05:47
0:05:47
 0:02:43
0:02:43
 0:04:41
0:04:41
 0:29:57
0:29:57
 1:22:20
1:22:20
 0:16:59
0:16:59
 0:11:23
0:11:23
 0:14:10
0:14:10
 0:07:15
0:07:15