Derivative of log x with any Base Explained | Calculus 1

Показать описание

We show the derivative of loga(x) is 1/(xlna) using implicit differentiation, our formula for the derivative of an exponential function a^x, and the chain rule! Then we plug in a=e to see the derivative of lnx is 1/x. We also find the derivative of log_3x using our new formula. #calculus1 #calculus

Join Wrath of Math to get exclusive videos, lecture notes, and more:

Guide to Implicit Differentiation: (coming soon)

◉Textbooks I Like◉

★DONATE★

Thanks to Matt Venia, Micheline, Doug Walker, Odd Hultberg, Marc, Roslyn Goddard, Shlome Ashkenazi, Barbora Sharrock, Mohamad Nossier, Rolf Waefler, and Shadow Master for their generous support on Patreon!

Outro music is mine. You cannot find it anywhere, for now.

Follow Wrath of Math on...

Рекомендации по теме

Комментарии

Another way to find the derivative of y=log_a(x) is to use the change of base formula in terms of ln(x). y=log_a(x)=ln(x)/ln(a). Therefore, we know that the derivative of ln(x) is 1/x. Multiply that by 1/ln(a), which is 1/(xln(a)). Therefore, y'=1/(xln(a)).

justabunga

Thank you so much, great explanation!

bradocksolo

awesome. very good explanation. thank you.

vil

Derivative of log x with any Base Explained | Calculus 1

Derivative of log x with any Base Explained | Calculus 1

Derivative of Logarithmic Functions

Derivatives of Logarithmic and Exponential Functions

Take the derivative of the natural log function

Differentiating Logarithmic Functions

Proof of the Formula for the Derivative of Log Base a of x

Derivative of log with arbitrary base

Proof: Derivative of log(x)

How to find the derivative of logx

differentiation of log x | differentiation of logarithmic functions | derivative of log x

How to differentiate y equals log x (log base 10)

The Derivative of the Logarithm Base a of x

Derivative Of logx with base 'a'| Differentiation Of log x with base a | Differentiation C...

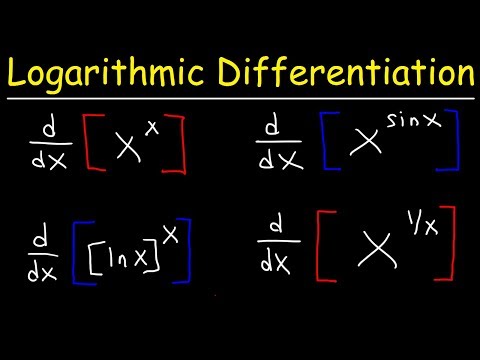
Introduction to Logarithmic Differentiation

Logarithmic Function Differentiation

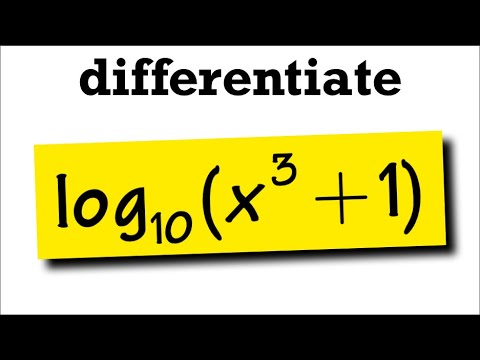
derivative of log base 10 of (x^3+1), with the box

Derivative of ln(1/x), calculus 1 tutorial

Find second derivative of log x Calculus

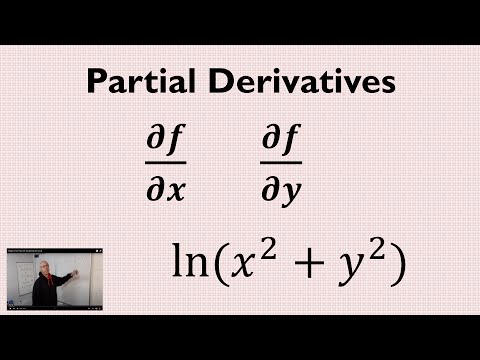
How to do Partial Derivatives with a Log function

Derivative of log x #Shorts

how do we know the derivative of ln(x) is 1/x (the definition & implicit differentiation)

How to find the Derivative of logarithmic function.

Derivative of log(x) by method of first principle

Find the second order derivative of the function log (log x) Ncert continuity and Differentiation