filmov
tv
how do we know the derivative of ln(x) is 1/x (the definition & implicit differentiation)
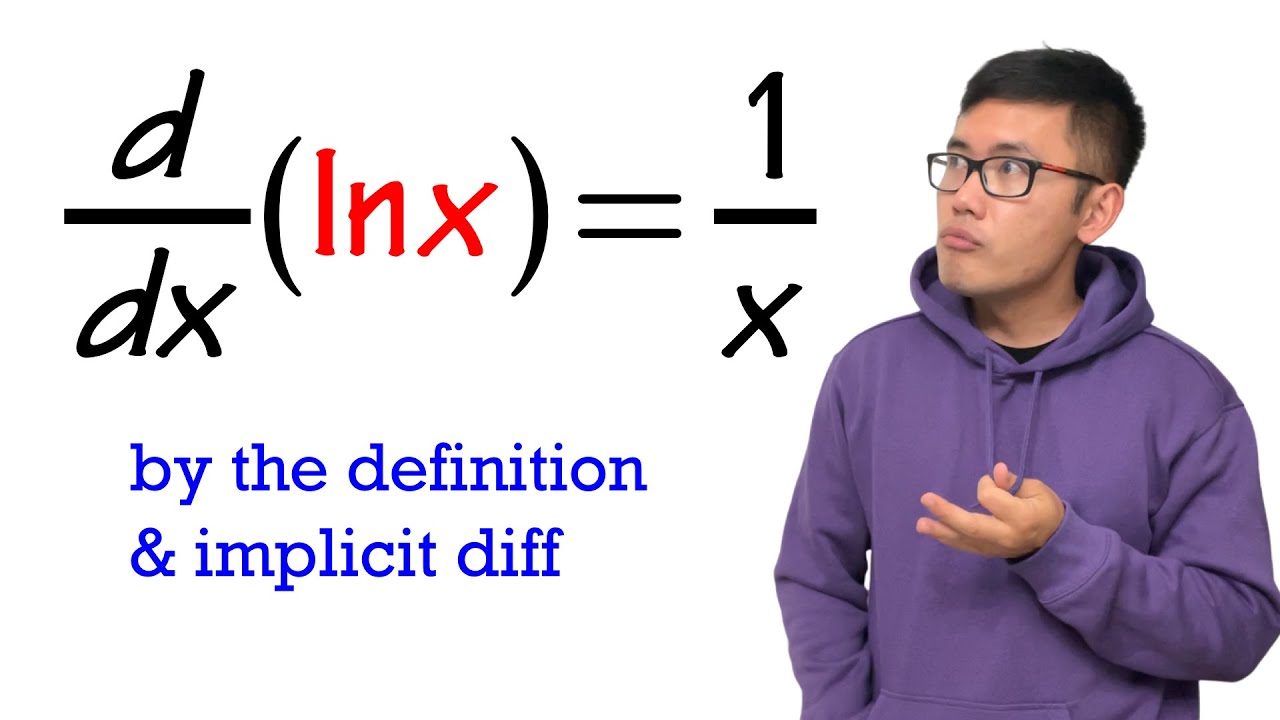
Показать описание
We will show that the derivative of ln(x), namely the natural logarithmic function, is 1/x. We will use the definition of the derivative and also implicit differentiation.
Subscribe to @bprpcalculusbasics for new calculus tutorials
Subscribe to @bprpcalculusbasics for new calculus tutorials
How Do We Know The Universe Is ACCELERATING?
How Do We Know the Age of the Universe?
How do we know the Universe is expanding?
How do you know you exist? - James Zucker
How Do We Know What Air is Like on Other Planets?
What Is An Atom And How Do We Know?
How do we know the Earth is round?
How Do We Know What Dinosaurs Looked Like?
HOW WELL DO YOU KNOW THE HOLYSPIRIT || PART 18 || BY APOSTLE LAWRENCE AMOFAH
How Do We Know What the Milky Way Really Looks Like?
Epistemology: How Do I Know? | Episode 1807 | Closer To Truth
How Do We Know What Stars Are Made Of?
How do we know we're not at the centre of the universe? 🪐 💫 - BBC
How Do We Know We’re Tired?
How do you know what's true? - Sheila Marie Orfano
How Do We Know What the Milky Way Looks Like?
How do we know what color dinosaurs were? - Len Bloch
How do you know you’re not dreaming? - Daniel Gregory
How do we know how long the Sun has left to live? | 7 things we need to know
How Do We Know Earth Is Changing?
How Do You Know If You’re A Genius?
How do we know there's a black hole in every galaxy centre? | History of Supermassive Black Hol...
How do we study the stars? - Yuan-Sen Ting
How do you know whom to trust? - Ram Neta
Комментарии
 0:03:27
0:03:27
 0:05:43
0:05:43
 0:05:33
0:05:33
 0:03:03
0:03:03
 0:02:43
0:02:43
 0:12:15
0:12:15
 0:07:22
0:07:22
 0:03:19
0:03:19
 1:13:42
1:13:42
 0:11:55
0:11:55
 0:26:48
0:26:48
 0:12:44
0:12:44
 0:03:41
0:03:41
 0:03:57
0:03:57
 0:05:12
0:05:12
 0:03:52
0:03:52
 0:04:24
0:04:24
 0:04:31
0:04:31
 0:23:47
0:23:47
 0:05:12
0:05:12
 0:03:46
0:03:46
 0:25:49
0:25:49
 0:04:45
0:04:45
 0:04:35
0:04:35