Ever heard of Quantum Operators and Commutators? (Explained for Beginners)!

What is a quantum operator? And just how useful are quantum commutators? Find out how they help us understand the Ehrenfest Theorem!

Hi everyone, I'm back with a new video! This time it's the first in a two-part mini-series on one of the coolest theorems in quantum mechanics - Ehrenfest's Theorem. With a good grasp of high school level mathematics, I believe it is possible to understand this theorem on a basic level. However, we need to do some groundwork first.

A quantum operator is a mathematical object / entity that corresponds to taking a measurement on a quantum system in real life. Examples of quantum operators include the position operator, the momentum operator, and the Hamiltonian operator. The Hamiltonian is an extremely important operator in quantum mechanics, consisting of kinetic energy and potential energy terms. It's also worth knowing that the operators we will deal with in this video are known as "observables". Entities that respond to things we can observe in the real world (such as position and momentum).

But one of the most interesting takeaways here is that in quantum mechanics, making a measurement is a complicated process. Unlike in classical physics, making a measurement doesn't necessarily affect or alter the system in some way, quantum mechanics tells us that a measurement fundamentally changes the wave function of the system. This process (of taking a measurement, causing the wave function to change) is known as the "collapse of the wave function". And mathematically, this process of making a measurement is represented by "applying" a quantum operator to the wave function ket of a system. The wave function, remember, is just a mathematical representation of all the information we have about our system. It is directly related to the probability distribution of the system, meaning it tells us the likelihood of finding particles that part of our system, in different locations in space (and much more).

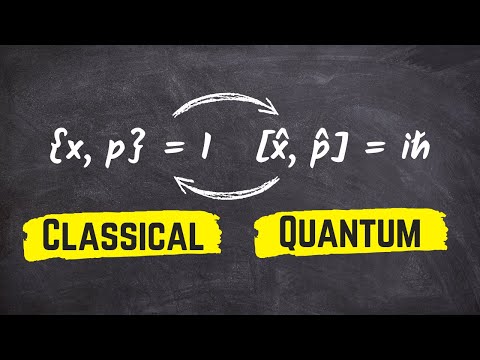
Now, because measurements are fundamentally system-altering in quantum mechanics, this means that the order in which we make two measurements can actually give us different results. For example, if we were to measure a quantum system's position, and then its momentum, we might get different results to if we measured its momentum first, and then its position. For this reason, it becomes useful to define a mathematical quantity known as the "commutator" between two measurement operators.

A commutator is just a measure of the difference in results when we measure one quantity (e.g. position) and then the other (e.g. momentum), as compared to when we measure them the other way round (i.e. momentum first, and then position). If switching the order gives us different results, then the commutator is not zero, and the order in which we make measurements needs to be considered carefully. However, in some cases the order does not matter. For such cases (and such pairs of operators), the commutator is zero. This is similar to saying 2 x 5 = 5 x 2. Multiplication is a commutative operation, meaning the order in which we multiply the two numbers does not matter.

Once we learn enough about operators and commutators, we can start to notice that they show up in Ehrenfest's theorem! In the finale of this two-part mini-series, we will be learning about expectation values (very important to QM) and then finally putting it all together to understand what Ehrenfest's Theorem is trying to tell us.

If you enjoyed this video, please do hit the thumbs up and subscribe for more fun physics content. Feel free to hit the bell button to be notified every time I upload. Check out my second channel, "Parth G's Shenanigans" for some music, and follow me on Instagram @parthvlogs to see what I get up to on a more day-to-day basis. Thanks for watching, I'll see you soon!

[ParthGChannel]

Hi friends! Part 2 of this series is out now! Check it out on my channel, or down below!

[anant_singh]

I was really willing to learn quantum operators. Thanks Parth for making this 😁😁

[subhampoddar]

Just love the way you explain difficult things. Your work is really appreciable. Thank you so much.

[exponentmantissa]

These videos are really well done. I am a retired engineer that always loved physics so I have spent a lot of time reading and studying since I wrapped up work.

[amjadalhindi]

Oh my god!!!!
1- your voice is calm and relaxing
2- you explain things smoothly
3- i JUST realized the physical meaning of commutation and the operator order things from YOU and i screamed! Mind blow!!
4- you sum up the video at the end, this shows you really *care* that viewers really *understand*!

I subscribed at one point when i was watching the video, then THREE times during it i thought of subscribing before realizing that i already did.

Sir, thank you!

[FabiFisica]

I'm amazed at this subject and the facility with which you explain it! I would also like you to give a physical interpretation of what it means for operators to commute. Keep it up and greetings from Colombia!

[clemenstombozichama]

Wish I knew about your channel earlier... Love how you explain QM. I'm officially a fan.

[robertschlesinger]

Very interesting, informative and worthwhile video. To say this video is excellent is an understatement; this video, and others by Parth are absolutely superb and a must see for anyone interested in the sciences.

[georgearrington]

Very nice presentation on commutators, Parth. You explained the value of knowing how this applies in QM problems.

[DKonigsbach]

A hundred "Likes". You are the first I've seen to give an intuitive conceptual explanation of commutators before giving the definition. Perfect!

[DM-mxpd]

I used laugh being indian when foreign people used say that everything becomes easy when an indian teaches you. After watching your videos I believe they were right.

[lisal]

Hi, I just wanted to say thank you so much! I am an undergraduate physics major and have my quantum physics exam in two weeks. This helped me so much! I was really confused after the lecture, but now I think I start to understand the topic. I wish you were my profesor...

[drover]

Watching this as a graduate doing a masters in physics. This is a really good description and explanation for approaching this topic of learning. Good work mate

[asdfafafdasfasdfs]

Wow I've been watching some of your videos and it's basically impossible NOT to understand.. best QM videos on YT to me, thank you!

[fikirbirru]

I have a hard time understanding this operators, but when you explain it, I now have a better understanding of it.

[lewisleslie]

Great video, my QM lecturer sucked at explaining commutators so this was very much appreciated!

[mnada]

You are doing a great job bringing these advanced notations to common people interested to explore the front shores of science. 👍

[sriranjit]

My High Sch Math Knowledge + My interest towards Quantum Mechanics = This video is awsm

[Higgsinophysics]

Really well explained <3 good to see you back :D

[quitecomplex]

This video is a pretty good video, and in general this channel provides something I think is missing from a lot of book, an explanation of what the physics is. I’ve read about commutators, but never understood why they were important until now.