filmov
tv
Unitary operators in quantum mechanics

Показать описание
📚 Unitary operators in quantum mechanics are used to describe physical processes such as spatial translations and time evolution. In this video, we discuss the basic properties of unitary operators and how we can transform quantum states and observables under the action of unitary operators.
⏮️ BACKGROUND
⏭️ WHAT NEXT?
~
Director and writer: BM
Producer and designer: MC
Ch 11: What are unitary operators? | Maths of Quantum Mechanics
Unitary operators in quantum mechanics
L5.2 Unitary Transforms (Quantum Mechanics and Computation)
Quantum Operators
Quantum Mechanics: Examples of Operators | Hermitian, Unitary etc.
Ch 9: What are Hermitian operators? | Maths of Quantum Mechanics
Ch 13: Where does the Schrödinger equation come from? | Maths of Quantum Mechanics
mod03lec29 - Unitary operators
Reverse Physics (Live): planning Quantum Mechanics
10 Unitary Operator | Projection Operator
2-2 Unitary operations
Unitary Time Evolution In Quantum Mechanics
Unitary Operator and Transformation in Quantum Mechanics (part 1)
Lecture 6: Unitary transformations in Quantum Computing
Operators in quantum mechanics
Unitary Transformation (Frame Change) for Hamiltonians and States, Quantum System Time Evolution
Lecture 4. Unitary Operators
Introduction to Operators in Quantum Mechanics
The time evolution operator in quantum mechanics
3. Symmetries : Unitary and Anti-unitary Operators | Weinberg’s Lectures on Quantum Mechanics
Lecture-2.3.2 Unitary Operator for Space Translation
Why are unitary transformations used in quantum mechanics?
Quantum Mechanics Lec 37 : Unitary Operator | GATE | IITJAM | CSIR-NET
Unitary Transformation
Комментарии
 0:06:35
0:06:35
 0:19:51
0:19:51
 0:10:45
0:10:45
 0:21:46
0:21:46
 0:07:43
0:07:43
 0:11:10
0:11:10
 0:14:58
0:14:58
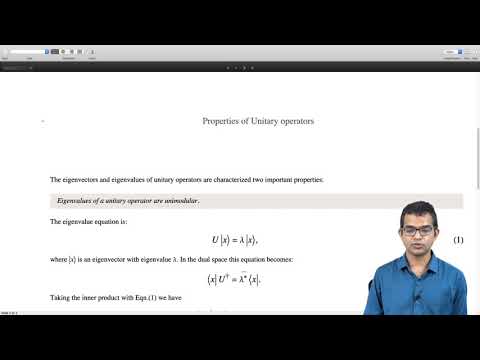 0:07:32
0:07:32
 1:38:46
1:38:46
 0:16:37
0:16:37
 0:12:57
0:12:57
 0:09:20
0:09:20
 0:09:11
0:09:11
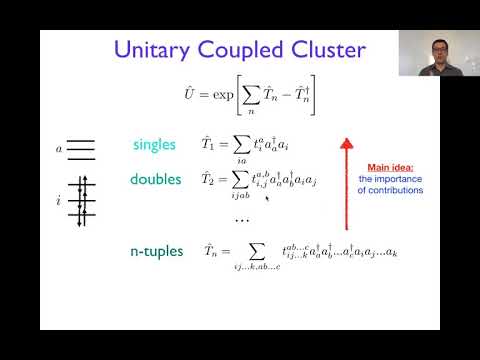 0:19:52
0:19:52
 0:13:07
0:13:07
 0:11:35
0:11:35
 0:34:38
0:34:38
 0:03:35
0:03:35
 0:17:50
0:17:50
 0:50:08
0:50:08
 0:09:02
0:09:02
 0:17:42
0:17:42
 0:09:10
0:09:10
 0:06:58
0:06:58