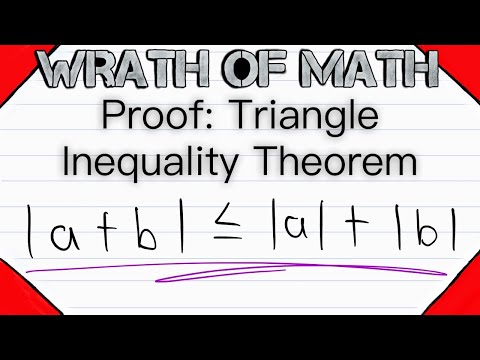
Theorem || Real Analysis || A continuous function is integrable

#riemannintegral #realanalysis #bsc #gate #msc

Riemann Integral Real Analysis Bsc Non Medical, MSc Mathematics

Theorem of Riemann Integral
Real Analysis
Finite number of points of Discontinuity
A Bounded function having a finite number of points of discontinuity on [a,b] is Integrable on [a,b]
Riemann Integral complete chapter
Real Analysis for CSIR NET

Descriptive video lectures taught by Karan Sir. Here you can watch all video lectures of Higher Mathematics for B.Sc , M.Sc, B.Tech, M.Tech, Csir Net, Gate, and Ph.D Mathematics.

Real Analysis Syllabus

• Course Description: The Real Analysis section will cover some basic analysis topics with a focus
on preparing you for first year doctoral-level courses. Topics covered will include sequences and
series, functions, and elemental measure theory.

1. Real Mathematical Analysis, Second Edition; Charles Chapman Pugh, 2015
2. Theory of Point Estimation, Second Edition, Lehmann, E. and Casella, G., 2015
.
• Main Topics:
1. Basic topology: compactness, metric spaces, open, closed, and bounded sets.
2. Sequences, series, and convergence: Cauchy sequences, bounded sequences, monotone sequences, power series, limsup, liminf, definition of big O and little o notation.
3. Functions: continuity, uniform continuity, differentiability, Taylor expansion, convex functions, sequences of functions.
4. Introduction to measure theory: definition and properties of measure, Lesbesgue measure,
measurable sets, measurable functions.
1
5. Integration: Riemann integral, Newton-Libniz integral, integration by parts, Lesbesgue integral, Fatou’s lemma, Fubini theorem
6. Distribution theory, complex numbers, Fourier transformation (if time allows)
• Quizzes: At the beginning of some classes there may be a short quiz. These will generally take
10 minutes and cover material covered in previous classes.

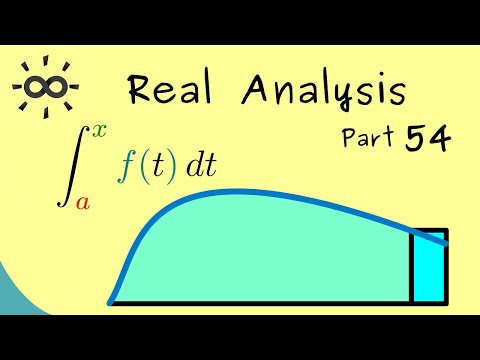
It defines a function to be Riemann integrable if the supremum over all dissections of the lower Riemann sums is the same as the infimum over all dissections of the upper Riemann sums. This value is then the value of the integral.

We explain and motivate this definition in the video and also give an example of proving a function is Riemann integrable over an interval and o different example of proving a function isn’t Riemann integrable over an interval.

Contents:
Part 1 - Definition of a dissection/partition
Parts 2 to 4 - Riemann Sums
Part 5 - Definition of Riemann Integration
Part 6 - Example of proving a function is Riemann Integrable