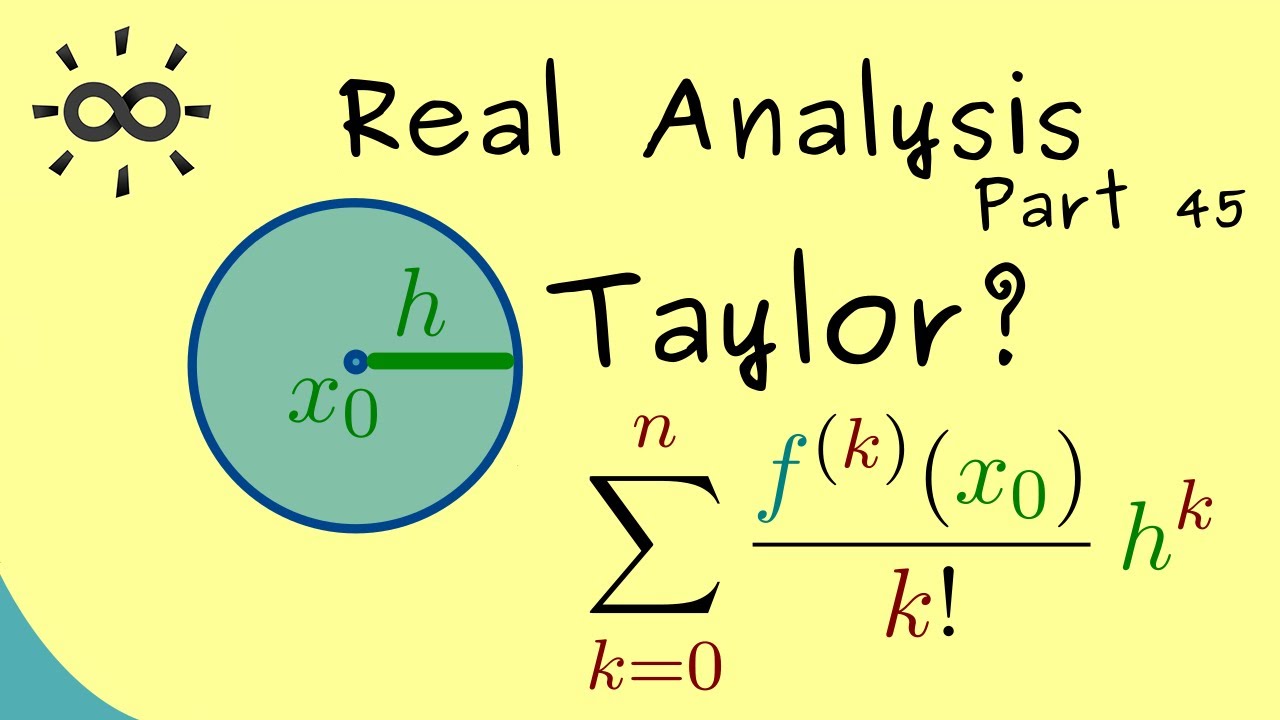
Real Analysis 45 | Taylor's Theorem

Please consider to support me if this video was helpful such that I can continue to produce them :)

🙏 Thanks to all supporters! They are mentioned in the credits of the video :)

This is my video series about Real Analysis. We talk about sequences, series, continuous functions, differentiable functions, and integral. I hope that it will help everyone who wants to learn about it.

x

#RealAnalysis
#Mathematics
#Calculus
#LearnMath
#Integrals
#Derivatives

I hope that this helps students, pupils and others. Have fun!

(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)

[lucaug]

As a CS major, I used Landau symbols all the time to describe the complexity of algorithms, but never knew the symbol actually had this name! The more you know hahahah
Great video as always! :)

[thomasjefferson]

Gotta say this whole video series is an amazing public service

[fastundercoverkitgoogle]

Damn. Writing (x - x_0) as h makes the formula so much easier to remember! Great work as always

[jamestanny]

I lost track at 2:11, when you introduced the equation for f(x0 + h). Could you please explain exactly how you came up with this equation, why is it this equation and how this relates to the pictured graph. Thank you!

[jamespashley]

I'm confused about just one thing; what do you mean by the remainder of h, or r(h)? 
what does it represent and how do you work it out

[jan]

Why do we need h^2 for the quadratic approximation ?

[xyz-fqlv]

How to derive that is not the part of video, what a mystery !

[mobbiblue]

gibts die Videoreihe auch auf deutsch?