filmov
tv
Verifying trig identities by adding rational terms

Показать описание
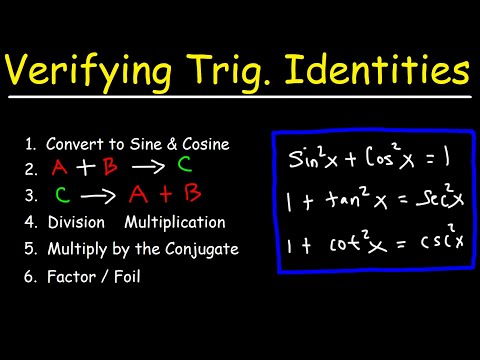
👉 Learn how to verify rational trigonometric identities involving the addition and subtraction of terms. To verify trigonometric expression means to verify that the term on the left-hand side of the equality sign is equal to the term on the right-hand side. To verify rational trigonometric identities, it is usually more convenient to start with getting rid of the denominator(s) of the rational term(s). This can be done by multiplying both the numerator and the denominator by the conjugate if the denominator involves addition/subtraction or by the reciprocal if the denominator involves product.
After evaluating the rational terms, we can then further reduce the trigonometric identity using any of the common trigonometric identities like the quotient, reciprocal, even, odd, co-function, and Pythagoras trigonometric identities or all the trigonometric functions can be converted to sine and cosine functions.
Organized Videos:
✅ Verify Trigonometric Identities
✅ Verify Simple Trigonometric Identities
✅ Verify Trigonometric Identities with Rational Expressions
✅ Verify Trigonometric Identities by Multiplying
✅ Verify Trigonometric Identities by Adding and Subtracting
✅ Verify Trigonometric Identities with Fractions
✅ Verify Trigonometric Identities by Pythagorean Identities
Connect with me:
#analytictrig #brianmlogan
After evaluating the rational terms, we can then further reduce the trigonometric identity using any of the common trigonometric identities like the quotient, reciprocal, even, odd, co-function, and Pythagoras trigonometric identities or all the trigonometric functions can be converted to sine and cosine functions.
Organized Videos:
✅ Verify Trigonometric Identities
✅ Verify Simple Trigonometric Identities
✅ Verify Trigonometric Identities with Rational Expressions
✅ Verify Trigonometric Identities by Multiplying
✅ Verify Trigonometric Identities by Adding and Subtracting
✅ Verify Trigonometric Identities with Fractions
✅ Verify Trigonometric Identities by Pythagorean Identities
Connect with me:
#analytictrig #brianmlogan
Комментарии
 0:04:52
0:04:52
 0:24:51
0:24:51
 0:06:53
0:06:53
 0:25:08
0:25:08
 0:05:12
0:05:12
 0:09:14
0:09:14
 0:07:37
0:07:37
 0:01:45
0:01:45
 1:12:58
1:12:58
 0:09:20
0:09:20
 0:06:58
0:06:58
 0:15:19
0:15:19
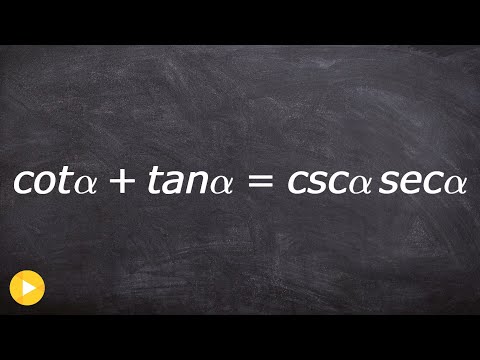 0:04:17
0:04:17
 0:04:36
0:04:36
 0:03:41
0:03:41
 0:06:35
0:06:35
 0:05:16
0:05:16
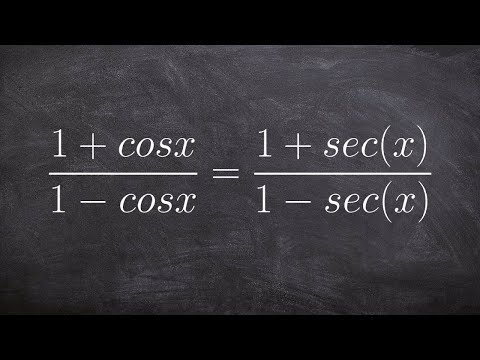 0:07:26
0:07:26
 0:08:26
0:08:26
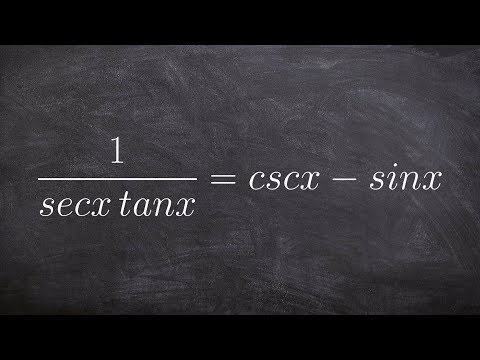 0:03:51
0:03:51
 0:55:39
0:55:39
 0:27:57
0:27:57
 0:04:30
0:04:30
 0:10:51
0:10:51