filmov
tv
How to Prove Trigonometric Identities (and how not to)

Показать описание
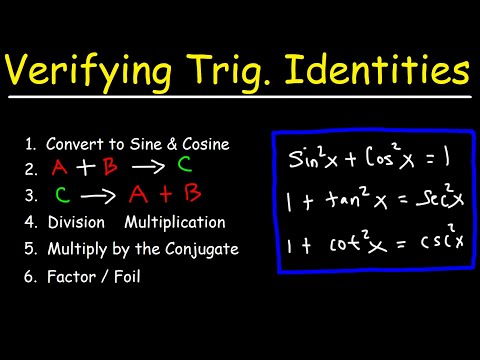
Verifying Trigonometric Identities
How to Prove Trigonometric Identities (and how not to)
Verifying Trigonometric Identities
Learn How To Prove A Trigonometry Question & Apply Trig Identities Effectively
Verifying Trigonometric Identities Easily - Strategy Explained (14 Examples)
The Best Way to Master Trigonometric Identities
Verifying trigonometric identities, hard with multiple steps
How to Prove Trigonometric Identities (Precalculus - Trigonometry 24)
How to Prove Trigonometric Identities | Example 6
Verifying a trigonometric Identities
Trig Identities
Proving Trigonometric Identities Grade 12
Mathematics Trigonometric Proofs: Solving Trigonometric Identities Part 1
Trig Visualized: One Diagram to Rule them All (six trig functions in one diagram)
Prove Trigonometric Identities Getting Started MCR3U
Intro to Trigonometric Identities - part 1
2 Useful Tips to Help You Prove Trig Identities
Strategy to Prove Trigonometric Identity
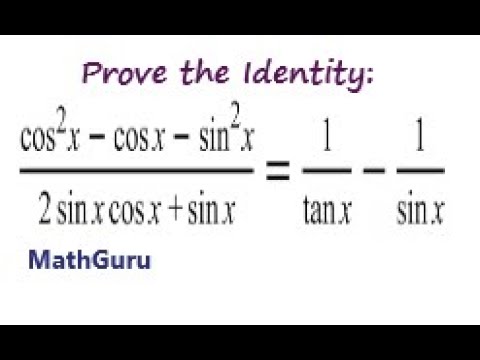
Prove this Trigonometric Identity | Step-by-Step Explanation
Trigonometric Identity Proofs (1 of 2: Overall strategies)
Trigonometric Identities
Proving Trig Identities | Past Exam | Mlungisi Nkosi
Verifying Trig Identities (Pre Calc)
Proving Trig Identities but they keep getting HARDER | jensenmath.ca
Комментарии
 0:24:51
0:24:51
 0:07:37
0:07:37
 0:09:14
0:09:14
 0:13:17
0:13:17
 0:25:08
0:25:08
 0:03:36
0:03:36
 0:06:53
0:06:53
 0:55:39
0:55:39
 0:05:15
0:05:15
 0:05:12
0:05:12
 0:27:57
0:27:57
 0:05:19
0:05:19
 0:11:45
0:11:45
 0:04:15
0:04:15
 0:07:57
0:07:57
 0:14:36
0:14:36
 0:10:00
0:10:00
 0:02:39
0:02:39
 0:07:23
0:07:23
 0:04:20
0:04:20
 0:11:20
0:11:20
 0:05:45
0:05:45
 0:09:20
0:09:20
 0:19:28
0:19:28