filmov
tv
There's more to 3B1B's absurd circle division pattern video | Hyperplane Arrangements

Показать описание
Original Title of this video: "3B1B There's a problem with your absurd circle division pattern video"
There's a tiny detail in the statement of Moser's Circle Problem that Grant gives that is a bit off if you think about it too much, and if you decide to address it like I do in this video we run into a different circle division problem, the lazy caterer problem, which gives rise to the lazy caterer's sequence and yet another connection to Pascal's Triangle. Continuing we also wander into the field of Hyperplane Arrangements. If you like thinking about the combinatorial nature of Moser's Circle problem presented in the 3Blue1Brown's video, you may be pleasantly surprised that problems like it are not just some piece of popular mathematics, but a whole mathematical industry in the research world in their own right. Hopefully this video finds you well!
Books on Hyperplane Arrangements:
_____________________
_____________________
WHAT GEAR I USED FOR THIS VIDEO:
DISCLAIMER: Links included in this description may be affiliate links. If you purchase a product or service with the links that I include here, I may receive a small commission. There is no additional charge to you! Thank you for supporting my channel and for helping me create these free videos!
#CHALK #3B1B #Hyperplanes
Комментарии
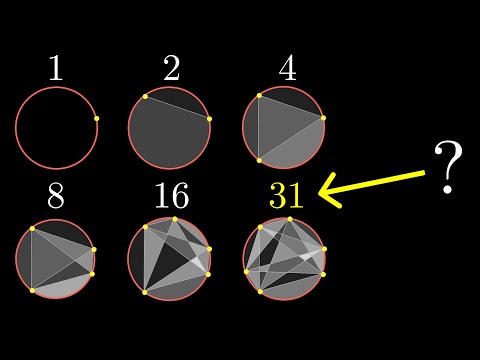 0:16:13
0:16:13
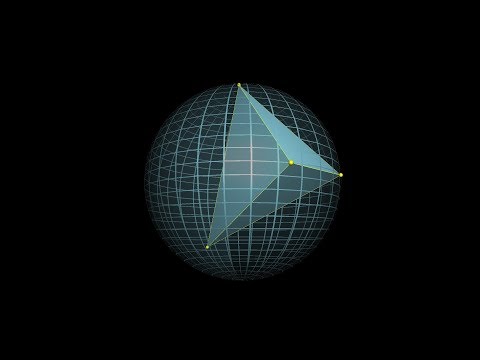 0:11:15
0:11:15
 0:29:42
0:29:42
 0:16:03
0:16:03
 0:21:58
0:21:58
 0:40:06
0:40:06
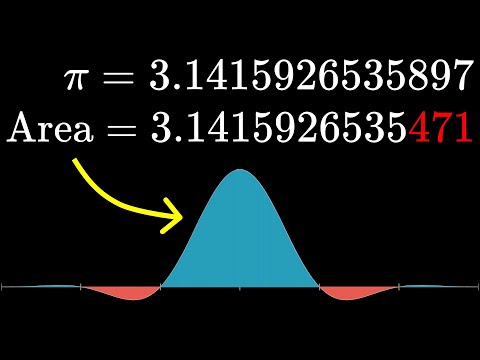 0:17:26
0:17:26
 0:24:46
0:24:46
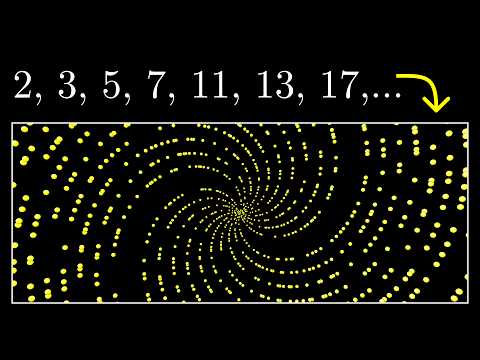 0:22:21
0:22:21
 0:00:44
0:00:44
 0:07:50
0:07:50
 0:24:14
0:24:14
 0:06:07
0:06:07
 0:22:09
0:22:09
 0:10:19
0:10:19
 0:00:22
0:00:22
 0:24:47
0:24:47
 0:01:19
0:01:19
 0:15:08
0:15:08
 1:22:11
1:22:11
 1:03:07
1:03:07
 0:10:21
0:10:21
 0:12:59
0:12:59
 0:00:44
0:00:44