filmov
tv
Complex Analysis 01: Inequalities

Показать описание
The two basic inequalities, and an application
Complex Analysis 01: Inequalities
Complex Analysis | Unit 1 | Lecture 11 | Inequality
The ML inequality for complex integrals | Complex Analysis | LetThereBeMath |
The Triangle Inequalities (1 of 3: Sum of Complex Numbers)
Complex Analysis: The Integral Inequality
Complex Analysis | Unit 1 | Lecture 10 | Triangle Inequality
How to Prove the Triangle Inequality for Complex Numbers
Complex Analysis (Lesson 1)
Complex Analysis | Unit 2 | Lecture 5 | ML Inequality
This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation
Complex Numbers 1(Definition, Addition, Subtraction, Multiplication and Division of Complex Numbers)
How REAL Men Integrate Functions
When mathematicians get bored (ep1)
Prove the Triangle Inequality for Complex Numbers
IIT Bombay CSE 😍 #shorts #iit #iitbombay
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
🔥 POV: Integration - Look at me! 👀 💪 | JEE 2024 | Math | Bhoomika Ma'am
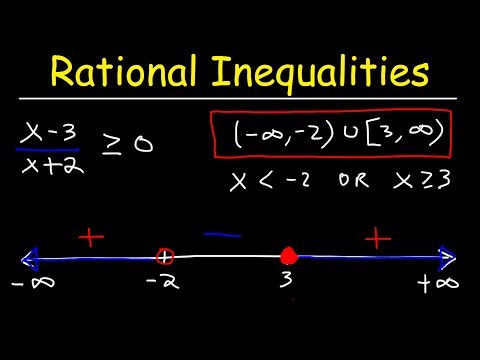
Rational Inequalities
Triangle inequality
Complex Analysis: Estimation Lemma (ML Inequality)
What is 'above' the complex numbers??
Intro Complex Analysis, Lec 2, Geometric Interpretations of Complex Arithmetic, Triangle Inequality
Trying transition video for the first time 💙😂 || #transformation #transition #shorts #viralvideo...
Complex Integration: The ML Inequality Proof and Example
Комментарии
 0:10:19
0:10:19
 0:04:35
0:04:35
 0:10:18
0:10:18
 0:10:46
0:10:46
 0:09:06
0:09:06
 0:05:52
0:05:52
 0:09:24
0:09:24
 0:07:01
0:07:01
 0:03:50
0:03:50
 0:00:16
0:00:16
 0:40:02
0:40:02
 0:00:35
0:00:35
 0:00:37
0:00:37
 0:08:45
0:08:45
 0:00:11
0:00:11
 0:00:38
0:00:38
 0:00:48
0:00:48
 0:10:18
0:10:18
 0:00:05
0:00:05
 0:08:56
0:08:56
 0:00:59
0:00:59
 0:59:21
0:59:21
 0:00:15
0:00:15
 0:10:51
0:10:51