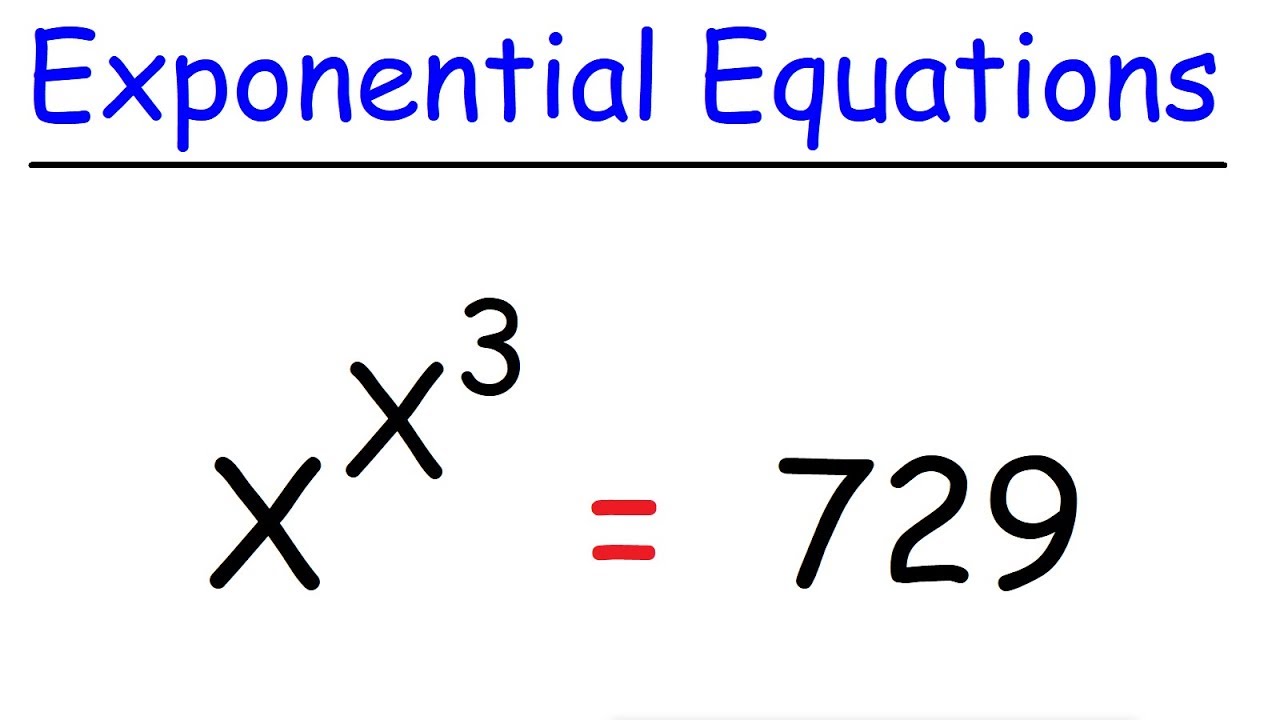
Exponential Equations With Powers of X

This math video tutorial explains how to solve exponential equations with powers of x.

_________________________________
Logarithms - The Easy Way!

Log to Exponential Form:

Change of Base Formula:

Change of Base Log Problem:

Properties of Logarithms:

____________________________________
Expanding Logarithmic Expressions:

Condensing Logarithmic Expressions:

Natural Logarithms:

Solving Exponential Equations:

Exponential Equations - Quadratic Form:

_______________________________________
Solving Logarithmic Equations:

Graphing Logarithmic Functions:

Graphing Exponential Functions:

Compound Interest Word Problems:

Logarithms Practice Problems:

_______________________________________
Final Exams and Video Playlists:

Full-Length Videos and Worksheets:

[rickyjames]

I think that this way to solve is for who already know the solution. You should decompose 729 before and then you play with x^x^3, comparing both. If you do like the video it's like throwing randomly hoping that it works

[marcellom]

This case in simple because have 729, but how do you do if you have another number for example 5?

[sabalsneh]

Moral of the story:- don't always take log . Wait and use other alternatives too .😁😁

[georget]

We could transform the problem in the form u^u=729, and apply the Lambert W function to solve it. Couldn't we?

[petrit]

Hello. Can you check again a to the power of two parentheses power of three?!

[georgesadler]

MR. Organic Chemistry Tutor, thank you for the video.

[josephmuriuki]

Your explanation is very clear, thank you very much.I was stuck with a problem of this nature thanks J.G.😅

[Andrew-dhws]

Khan academy should hire you, no joke

[aritrajb]

Huh! I expected some integer and this turned out to be an irrational number.
Moral: Never be the die hard fan of Pythagoras!

[TheLordrain]

can we find all x^x^n = k equalities with only using algebra logarithm and stuff? (of course not with this technique)

[haha-orsz]

Bu iyiydi. Yeni bir bakış açısı kazandım. Teşekkürler

[Goku_is_my_idol]

So i tried to solve this ques from thumbnail
Now right side is a power of 3
Take x= 3^(k/3)
We get 3^(k*3^(k-1))= 729= 3^6
==> k*3^(k-1)= 6
==> 3^(k-2)= 2/k
Now we shall compare their graphs to see how many solutions are possible
Since in the negative k --- 3^(k-2) lies in the second quadrant and 2/k lies in the third quadrant they never intersect
One of them is increasing and the other one is decreasing. So if they intersect that particular point will be the only point of intersection
Its obvious that k=2 satisfies
x= 3^(2/3)

[AkashKumar-czgm]

x^(4*x^2) = 1/sqrt(2) How do you solve this equation?

[buildandmakewithnila]

On step no. 3, how did you bring it out, am confused

[rcnayak_]

This is an alternative solution to the problem, maybe bit easier. The order of evaluation of any exponential number such as 2^3^2 is 2^(3^2) so that the answer is 2^9 which is 512. And not 64 as some calculate. Now let us proceed. Our question is x^x^3 = 729. Let x^3 = k. So that x = (k)^(1/3). Our left hand side term now can be expressed as x^k which is {(k)^(1/3))^k}. This equals to k^(k/3) which can be expressed as (k^k)^(1/3) which is equal to 729 (9^3). So that k^k becomes ((9^3)^3) = 9^9. Therefore k^k = 9^9 i.e, k = 9. In other words, x^3 = 9 which makes x = cube root of 9.

[BinaHejazi]

actually (x^x^3)=729 can be written as (x^2)^3=9^3. taking cube root of both sides we have: x^2=9 and hence x=3 and thats the answer!!
isnt that right?

[saktishvikmend]

hi.. i need your help pls. 3x + 3^(x+1) + 3^(x-1) = 13. Whats x?

[nataliexelena]

What app/website/thing do you use to write with?

[eliaselieza]

Nimeelewa sana, let apply your logic concepts,

[kamranshadkhast]

(x^x)^3 is different with x^(x^3) which one do you choose to go ahead and why?? when it is not specified by prantesis.