Number Sequences in the Mandelbrot Set

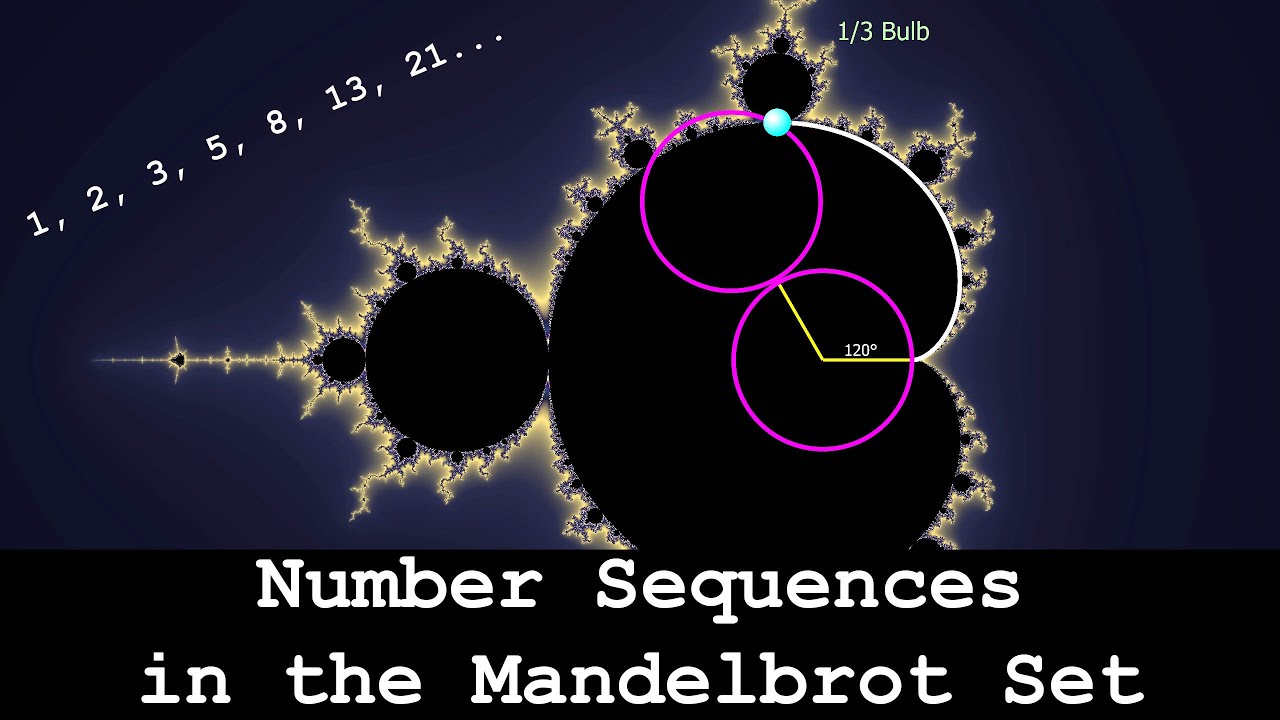
Welcome to part 4 of our little Mandelbrot Explained series. In this video we explore the bulbs around the main cardioid, and find that they contain number sequences such as the natural numbers, Fibonacci sequence, and the rational numbers. We then investigate them in terms of their Julia Sets to try and understand visually why they are there. Finally, we look at precisely where the bulbs are attached to the cardioid.

In this video:
00:00 Introduction
00:30 Period of the bulbs
01:45 Signposts in the Mandelbrot Set
02:42 Number Sequences
04:24 Rational Numbers
07:06 Julia Sets of the bulbs
11:36 Location of the bulbs
13:15 Why signposts?
14:30 Building a Mandelbrot Set

In this series:

Extra Visuals (No commentary):

Mentioned in video:

[EvilSandwich]

Could you do the internet favor and just post images of that mandelbrot set made out of a circle with degree marks to as many social media sites as you can?

That is probably one of the best and most important visuals I got out of this entire fantastic series.

[vladimirarnost]

My jaw has dropped when watching this video and I can't find it. It's probably somewhere in the complex plane, in a dark place behind one of the Mandelbrot bulbs.

Absolutely mindblowing stuff. 🤯 Thank you!

[denelson]

4:57 - I never knew you could get the numerator that way!

You should really put together a fifth video in this series, dealing with other concepts connected to the Mandelbrot set, such as finding other number sequences in the bulbs like the powers of two, external rays, equipotential curves, Misiurewicz points, Siegel discs, how you can derive a bifurcation diagram from the set, the Buddhabrot variation, and even how you can calculate π just by using the set.

[asherwilkins]

oh man I got into fractals with the discovery of the fibbonocci sequence, and how I kind of discovered it myself butlater in life learning its incredibly implications in life and physics, and I've watched that numberphule video probably 10 times trying to best understand what she is getting at, but this is just what I needed. Thanks you good sir, and just know I lovetthese videos so much.

[MarshallBrandt]

Thank you for your intuitive visual explanations of these beautiful patterns. Can't wait for your future videos!

[RobCozzens]

Thank you for these videos! I've been interested in the Mandelbrot set for years and these are some of the most informative videos for giving a taste of "why" it looks the way it does.

[wallywutsizface]

The thing about the bulbs matching with the internal angle of the cardioid is insane

[zfloyd]

Jeez, I never knew that the period 2 bulb was the only perfect circle.

[ronancoyle]

Wow! thanks for the clear insights. I was always happy to just wonder at the the sets, knowing natural sequences were echoed in them. This video has just bent my head enough to kinnnd of understand a little more.

[SmokeyDope]

Youre awesome dude i wish this series got more views. I finished all 4 and keep coming back. Hope you make some more videos about the Mbrot, if not its understandable as im sure this takes a huge chunk of time to produce with animations and all. Thanks for your work!

[mnada]

That is beyond imagination. Thank you.

[SmokeyDope]

Hey Mr. Mathemagicians Guild, when you get back into making mandelbrot set videos, can you please talk about Mandelbrots of different exponents (Zn=Z^X+C), what happens as the exponent N approaches infinity, and what a mandelbrot set looks like with a imaginary/complex exponent? I tried making a imaginary mandelbrot using the fractal imaging software Xaos and posted my findings on my channel, however i think a proper hand coded imaging method is needed to properly view them in good quality. Finally i would love to hear your take on how the mandelbrot set and bifuration diagram/logistic map are connected. Thank you!

[johnadriandodge]

Shalom and evening howdy how.
Very nicely done and thank you for sharing!

[utilizator]

6:22 in other words, the rational number sequence in Mandelbrot set is the form m/n, where n is a natural number greater than 1, and m is a number which it's congruent modulo n admits inverse in mod n.

[galaxygur]

00:06:34 - The Mandelbrot set reveals an infinite number of fractions between 0 and 1, each with its own unique bulb.

00:12:01 - Only rational numbers can find a periodic equilibrium in the Julia set, forming the bulbs in the Mandelbrot set.

[danielvieira]

my theory is that the size of the circle needed to build the main cardioid is the same size as the period 2 circle.

[ivymike]

Imagine a VR Mandelbrot in 3D where you can also see the values for each point you follow.
Although math is conceptual, we clearly see the corresponding mirror in tangible nature such as crystal formation, plant formation, and interstellar body formations. Is this by accident or design? 🤔

[abacussssss]

Love this series! Any chance external rays will be covered at some point? The fact that _any interesting point at all_ is representable by a rational number seems even more incredible than the rational numbers of the bulbs.

[zfloyd]

Also, can you make a video on why minibrots are distorted? I would really like to know.

[Szabolcs]

All this is incredible. Btw, does that mean all the "mini mandelbrots" are distorted as well?