filmov
tv
Find Area Of Circle Inscribed in An Equilateral Triangle with side length 10 | 2 methods

Показать описание
Today I will teach you tips and tricks to solve the given olympiad math question in a simple and easy way. Learn how to prepare for Math Olympiad fast!
Step-by-step tutorial.
Need help with solving this Math Olympiad Question? You're in the right place!
Subscribe my Channel:
@BlacksmithTWD
@prafulherode463
@prafulherode7819
@oliverderks8928
@pianobar1956
@globalvoice.5603
@cicerochen313
@bricepaya4865
@jaumesues
@aishwarynigam605
@affan4183
@dashinggamerz9874
@dashinggamerz9236
@devilhorrorgamer1142
@iophop9266
@sohomsen2922
@magicalblacksmithplays9765
@kennethstevenson6925
@kennethstevenson9237
@ronaldleeman6441
@indiathenextsuperpower7256
#FindAreaOfTheCircle #AreaOfCircle #GeometryMath #MathOlympiad #IsoscelesTriangle
#OlympiadMathematicalQuestion #HowToSolveOlympiadQuestion #MathOlympiadQuestion #MathOlympiadQuestions #OlympiadQuestion #Olympiad #AlgebraReview #Algebra #Mathematics #Math #Maths #MathOlympiad #HarvardAdmissionQuestion
#MathOlympiadPreparation #LearntipstosolveOlympiadMathQuestionfast #OlympiadMathematicsCompetition #MathOlympics #CollegeEntranceExam
#blackpenredpen #MathOlympiadTraining #Olympiad Question #GeometrySkills #GeometryFormulas #CircumscribedCircle #PythagoreanTheorem #TwoTangentTheorem #CircleTheorem #PerpendicularBisectorTheorem
#MathematicalOlympiad #OlympiadMathematics #CompetitiveExams #CompetitiveExam
How to solve Olympiad Mathematical Question
How to prepare for Math Olympiad
How to Solve Olympiad Question
How to Solve international math olympiad questions
international math olympiad questions and solutions
international math olympiad questions and answers
olympiad mathematics competition
blackpenredpen
math olympics
olympiad exam
olympiad exam sample papers
math olympiad sample questions
math olympiada
British Math Olympiad
olympics math
olympics mathematics
olympics math activities
olympics math competition
Math Olympiad Training
How to win the International Math Olympiad | Po-Shen Loh and Lex Fridman
Po-Shen Loh and Lex Fridman
Number Theory
There is a ridiculously easy way to solve this Olympiad qualifier problem
This U.S. Olympiad Coach Has a Unique Approach to Math
The Map of Mathematics
mathcounts
math at work
Pre Math
Olympiad Mathematics
Olympiad Question
Geometry
Geometry math
Geometry skills
Right triangles
imo
Competitive Exams
Competitive Exam
Inscribed Circle
Circumscribed Circle
Pythagorean Theorem
Two Tangent Theorem
Circle Theorem
Tangents
Isosceles Triangle
Perpendicular Bisector Theorem
Area of the circle formula
Area of the circle
Subscribe Now as the ultimate shots of Math doses are on their way to fill your minds with the knowledge and wisdom once again.
Комментарии
 0:07:41
0:07:41
 0:04:33
0:04:33
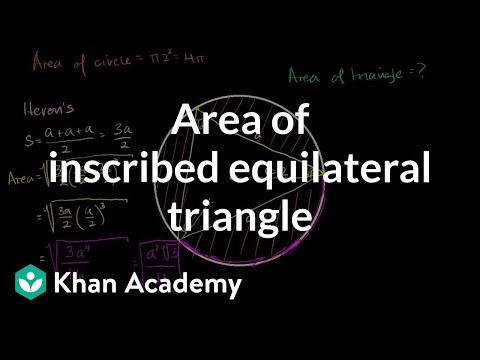 0:11:39
0:11:39
 0:06:59
0:06:59
 0:00:28
0:00:28
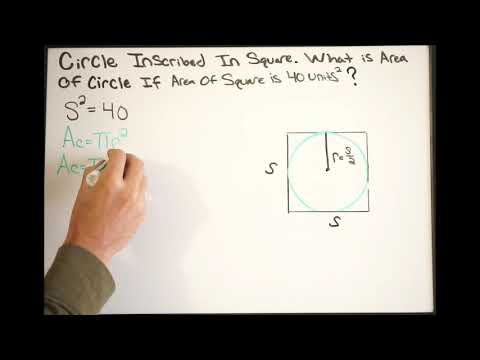 0:02:30
0:02:30
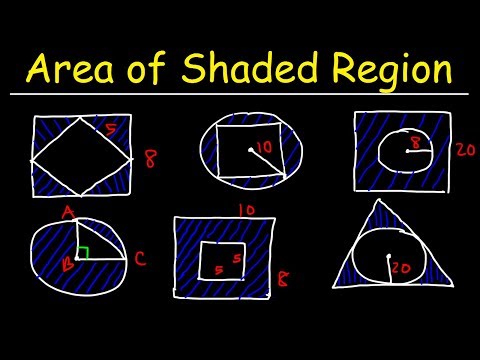 0:13:36
0:13:36
 0:05:53
0:05:53
 4:49:17
4:49:17
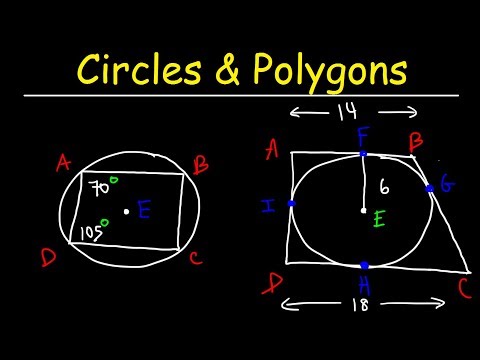 0:12:50
0:12:50
 0:04:31
0:04:31
 0:03:42
0:03:42
 0:08:08
0:08:08
 0:02:38
0:02:38
 0:01:47
0:01:47
 0:09:27
0:09:27
 0:02:03
0:02:03
 0:08:27
0:08:27
 0:05:15
0:05:15
 0:15:31
0:15:31
 0:04:06
0:04:06
 0:08:49
0:08:49
 0:08:47
0:08:47
 0:03:28
0:03:28