Periodic billiard paths on regular polygons (Diana Davis)

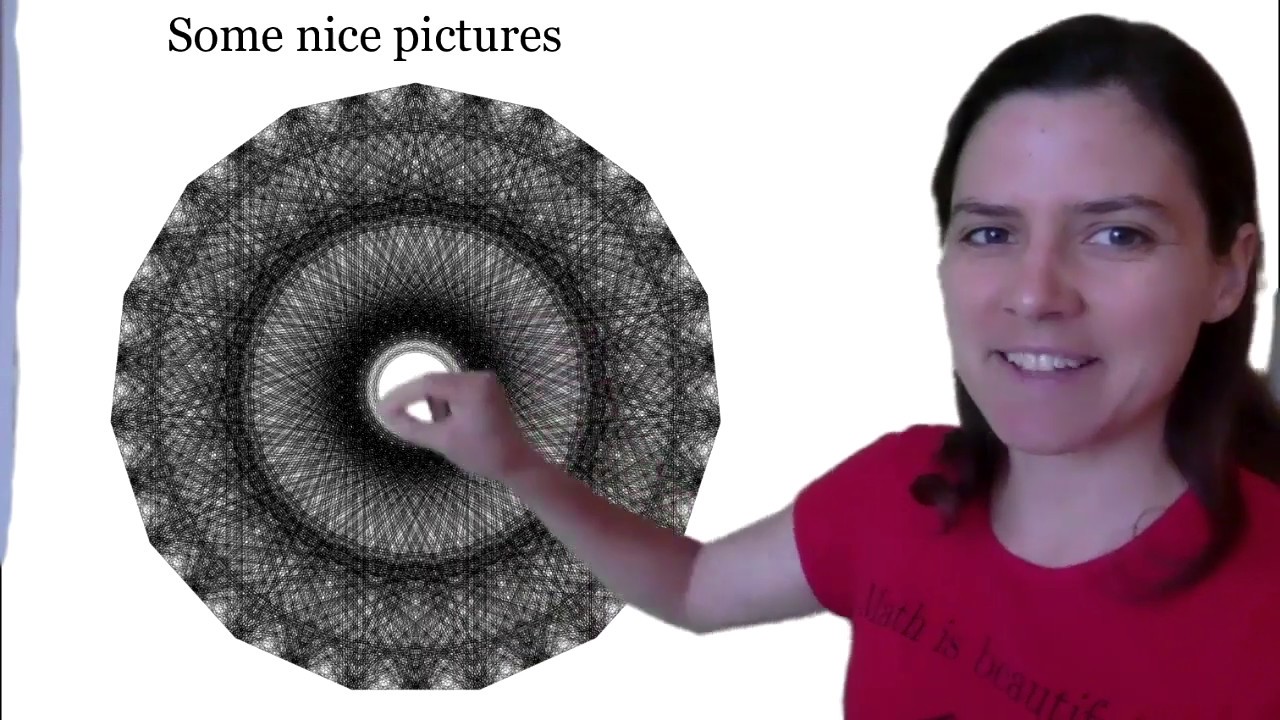
preview_player
Показать описание
Abstract: Mathematicians have understood periodic billiards on the square for hundreds of years, and my collaborator Samuel Lelièvre and I have understood them on the regular pentagon for about five years now. During the COVID-19 pandemic, I have been in France, working with Samuel to extend our understanding to all regular polygons with an odd number of sides. In this talk, I’ll briefly explain results and techniques for the square and pentagon, and then show lots of nice pictures of billiards on polygons with more than 5 sides, that we have created recently.

I made this with a green screen (a.k.a. a tablecloth hanging from an Ethernet cord) and 85 consecutive virtual backgrounds.

A talk for the Nearly Carbon Neutral Geometric Topology conference, June 2020.
  1. Diana Davis
Рекомендации по теме
Periodic billiard paths 0:20:12

Periodic billiard paths on regular polygons (Diana Davis)

Does every triangle 0:04:49

Does every triangle have a periodic billiard path?

Diana Davis, Periodic 0:47:30

Diana Davis, Periodic billiards on regular polygons

Periodic Paths on 1:02:42

Periodic Paths on Regular Polygons

Periodic paths on 0:59:08

Periodic paths on regular polygons

Periodic paths on 1:02:05

Periodic paths on regular polygons -- at University Nebraska

Families of periodic 0:02:15

Families of periodic trajectories on the regular pentagon

D. Davis - 0:53:10

D. Davis - Periodic paths on the pentagon

Tutorial: Periodic trajectories 1:12:35

Tutorial: Periodic trajectories in billiards - and where to find them

Periodic paths on 1:14:45

Periodic paths on the pentagon and friends -- at Trinity University

Periodic paths on 1:02:45

Periodic paths on the pentagon: Swarthmore College Math/Stat Colloquium

[LoopMe] Periodic Orbit 0:01:17

[LoopMe] Periodic Orbit Families in a random Right Triangle

Periodic trajectories on 0:23:40

Periodic trajectories on the regular pentagon - faculty lunch talk 1/31/18

Periodic paths on 1:09:41

Periodic paths on the pentagon -- at LG&TBQ conference at U of M

Periodic paths on 1:26:29

Periodic paths on the pentagon -- joint Brazil-France meeting at IMPA

Problems with Periodic 0:06:02

Problems with Periodic Orbits - Numberphile

Trying transition video 0:00:15

Trying transition video for the first time 💙😂 || #transformation #transition #shorts #viralvideo...

Boyle’s Law 0:00:15

Boyle’s Law

Simple Harmonic Motion 0:00:25

Simple Harmonic Motion

Leonardo Macarini - 1:00:26

Leonardo Macarini - Periodic orbits on symmetric spheres and dynamical convexity

11 years later 0:00:11

11 years later ❤️ @shrads

Period on the 0:00:41

Period on the road 😱 | Omg..

Billiard dynamics and 0:06:35

Billiard dynamics and the Birkhoff-Poritzky Conjecture

Bromine is scary 0:00:49

Bromine is scary

Комментарии
Автор

With a huge respect this is a strong concept, , one request for you that please make a video on tensor maybe short video...

puneetkumar
Автор

This channel will be boost very soon we r here for ur support 🙏

puneetkumar
Автор

I request you to upload your teaching lectures on real analysis and theory of numbers, it's humble request madam

MANISHKUMAR-tcbs
Автор

Hi Diana, apart from drawing pretty pictures what world applications are there? What return on investment did you offer tax payers who funded your research?

ChauTu