filmov
tv
Example of Basis for a Null Space

Показать описание
Linear Algebra: Find a basis for the null space of the matrix A = [ 1 0 3 2 1 \ 0 2 2 4 4 \ 0 0 0 2 6 ]. We use reduced row echelon form to assign dependent and independent variables.
Example of Basis for a Span
Basis and Dimension
Check something is a basis
Change of Basis
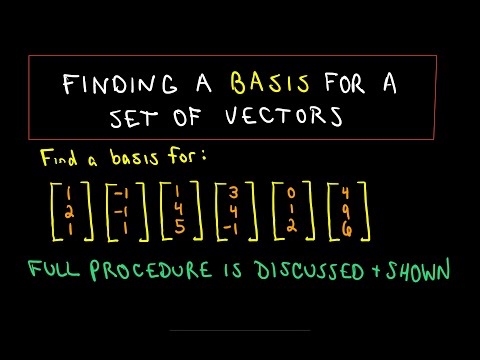
Find a Basis for a Set of Vectors
Finding Basis for the Column Space of a Matrix | Linear Algebra
Change of basis | Chapter 13, Essence of linear algebra
Example of Basis for a Null Space
🔵 Imago Meaning - Imago Examples - Imago Definition - Psychology - Imago
Linear Algebra Example Problems - Vector Space Basis Example #1
Example of Change of Basis
Linear Algebra Example Problems - Basis for an Eigenspace
Basis and dimension
Basis for a Set of Vectors
Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra
Basis and dimension example
Example: Writing a vector in a new basis
Finding Basis for Column Space, Row Space, and Null Space - Linear Algebra
Change of Basis (Example 1)
Dimension and Basis of Vectors | How to find Basis and Dimension of a Vector Space (with example)
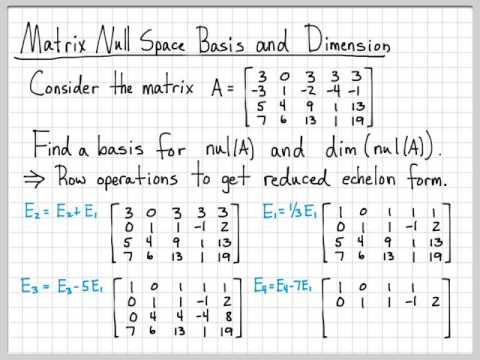
Linear Algebra Example Problems - Matrix Null Space Basis and Dimension
Linear Algebra Example Problems - Matrix Row Space Basis and Dimension
Examples of Basis and standard basis of a vector space
The Vector Space of Polynomials: Span, Linear Independence, and Basis
Комментарии
 0:06:37
0:06:37
 0:10:06
0:10:06
 0:03:53
0:03:53
 0:09:34
0:09:34
 0:07:17
0:07:17
 0:03:59
0:03:59
 0:12:51
0:12:51
 0:04:35
0:04:35
 0:05:24
0:05:24
 0:04:47
0:04:47
 0:07:11
0:07:11
 0:07:48
0:07:48
 0:06:20
0:06:20
 0:11:43
0:11:43
 0:09:59
0:09:59
 0:04:25
0:04:25
 0:08:55
0:08:55
 0:18:31
0:18:31
 0:10:27
0:10:27
 0:04:02
0:04:02
 0:09:16
0:09:16
 0:03:45
0:03:45
 0:33:03
0:33:03
 0:12:50
0:12:50