filmov
tv
Derivative of y = x^x^x #jeemains #derivatives #jee

Показать описание
Derivative of y=x^x^x | logarithmic Differentiation #derivatives #jonahemmanuel #excellenceacademy
the most fun derivative of x^x^x^...
Derivative of x^x^x - Logarithmic Differentiation of Exponential Functions
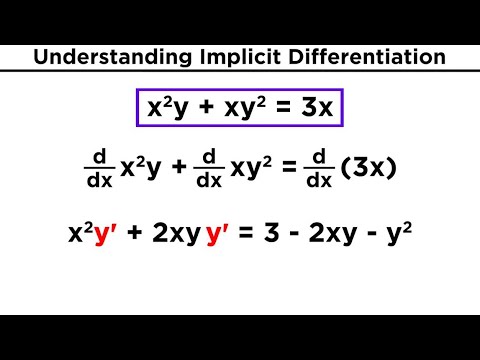
Implicit Differentiation
Derivative of x^x
find the derivative of y = x*sqrt(x)
Derivative of xy
derivative of x^y=y^x, implicit differentiation , calculus 1 tutorial, AP calculus
x^2+y^2=(z-c)^2Tan^2α forming PDE by eliminating arbitrary constants #PDE L1k,133
derivative of x^x
How to find the derivative dy/dx for y^x=x^y (calculus 1 tutorial logarithmic differentiation)
Implicit Differentiation
Derivative of y = xlnx - x
Difference Between Partial and Total Derivative
derivative for e^(x/y) = x - y, calculus 1 tutorial
derivative of x^(1/x), calculus 1 tutorial
find the derivative of y = (4x-1)^3
Derivative of e^xy (Implicit Differentiation) | Calculus 1 Exercises
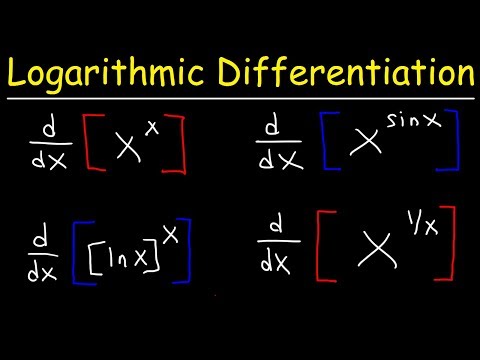
Introduction to Logarithmic Differentiation
Derivative of y = x^x^x #jeemains #derivatives #jee
y = x^3e^x, find the derivative
Find the Derivative of y = 2x*sqrt(x^2 + 1)
Differentiation by First Principle Method | Derivative #jonahemmanuel #excellenceacademy
How to find the derivative of square root of x (steps)
Комментарии
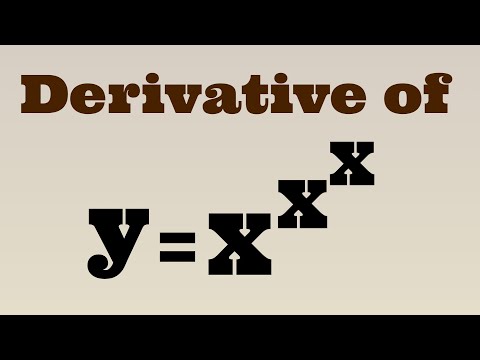 0:06:26
0:06:26
 0:05:28
0:05:28
 0:11:46
0:11:46
 0:11:45
0:11:45
 0:03:02
0:03:02
 0:00:44
0:00:44
 0:01:46
0:01:46
 0:05:02
0:05:02
 0:15:54
0:15:54
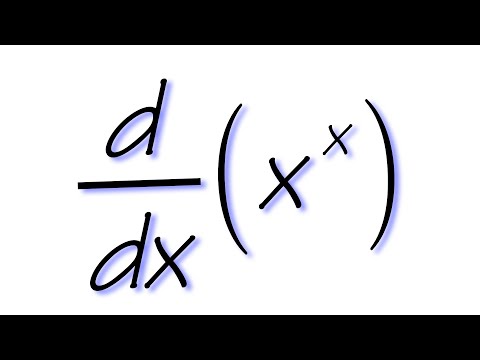 0:07:54
0:07:54
 0:05:06
0:05:06
 0:14:34
0:14:34
 0:00:44
0:00:44
 0:01:44
0:01:44
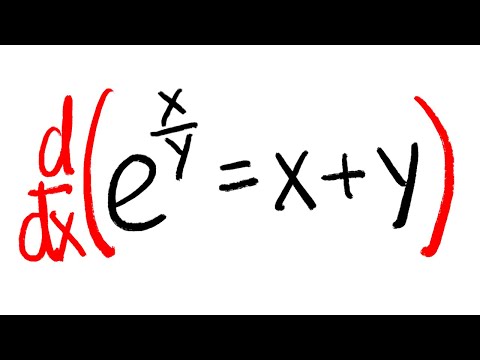 0:05:24
0:05:24
 0:09:51
0:09:51
 0:00:34
0:00:34
 0:03:37
0:03:37
 0:13:31
0:13:31
 0:00:47
0:00:47
 0:00:40
0:00:40
 0:05:01
0:05:01
 0:10:58
0:10:58
 0:01:24
0:01:24