filmov
tv
Integral of sqrt((1-x)/(1+x))

Показать описание
here's how to integrate x/sqrt(1-x^2)
Integral of sqrt((1-x)/(1+x))
Subscribe to @bprpcalculusbasics for more calculus tutorials.
Integral of sqrt((1-x)/(1+x))
Subscribe to @bprpcalculusbasics for more calculus tutorials.
Integral of sqrt((1-x)/(1+x))
Integral of Sqrt (1-x)/(1+x) ❖ Calculus 1
Evaluate : `int sqrt((1+x)/(1-x))dx`
integrate sqrt((1+x)/(1-x)) dx
integral of sqrt(1+1/x), by u-substitution
Integral of sqrt(1 - 1/x)
Integral of sqrt((1+x)/(1-x)) - Integral example
integral of sqrt(1+sqrt(x))
( No Audio) Calculus-Integration: Integral of (x^2- 3x+1) / sqrt(1- x^2) by trigonometric substituti
Trigonometric Substitution the Integral of sqrt(1 - x)/sqrt(x)
Integral of x*sqrt(1-x^2) vs. integral of sqrt(1-x^2), u-substitution vs. integration by parts
Evaluate the Integral dx/(sqrt(x) (1+x)). Inverse Trig Functions
integral of sqrt(1+x^2)/x vs integral of x/sqrt(1+x^2)
Steps to Understand Substitution Method to Integrate sqrt(1 + x^2) x^5
Integral of 1/sqrt(x)
İntegrating x/sqrt(x+1) | Calculus
Q6, integral of 1/sqrt(1-x^2) vs. integral of x/sqrt(1-x^2)
Integral of sqrt(x)/sqrt(1-x^3) (substitution)
The Integral of sqrt(1-x^2) from 0 to 1 Using U-Substitution
How to Integrate x/sqrt(x-1) using a Substitution
integral of sqrt(1-x^2), trig substitution, calculus 2 tutorial
Integral of 1/x
Integral x*sqrt(x-1). Apply integration by parts, square root example. Choose u=x!
Int{dx/sqrt(x(1-x)) dx} from 0 to 1
Комментарии
 0:02:59
0:02:59
 0:04:33
0:04:33
 0:03:24
0:03:24
 0:02:43
0:02:43
 0:04:42
0:04:42
 0:09:26
0:09:26
 0:03:40
0:03:40
 0:02:15
0:02:15
 0:04:50
0:04:50
 0:06:52
0:06:52
 0:09:15
0:09:15
 0:02:58
0:02:58
 0:11:51
0:11:51
 0:06:26
0:06:26
 0:02:15
0:02:15
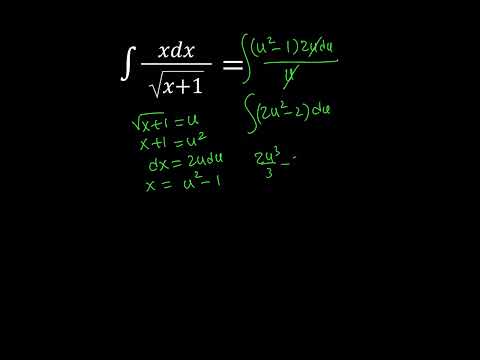 0:00:21
0:00:21
 0:03:40
0:03:40
 0:02:55
0:02:55
 0:03:50
0:03:50
 0:03:38
0:03:38
 0:03:41
0:03:41
 0:00:50
0:00:50
 0:03:04
0:03:04
 0:08:39
0:08:39