Linear Independence of Vectors Example 4

Показать описание

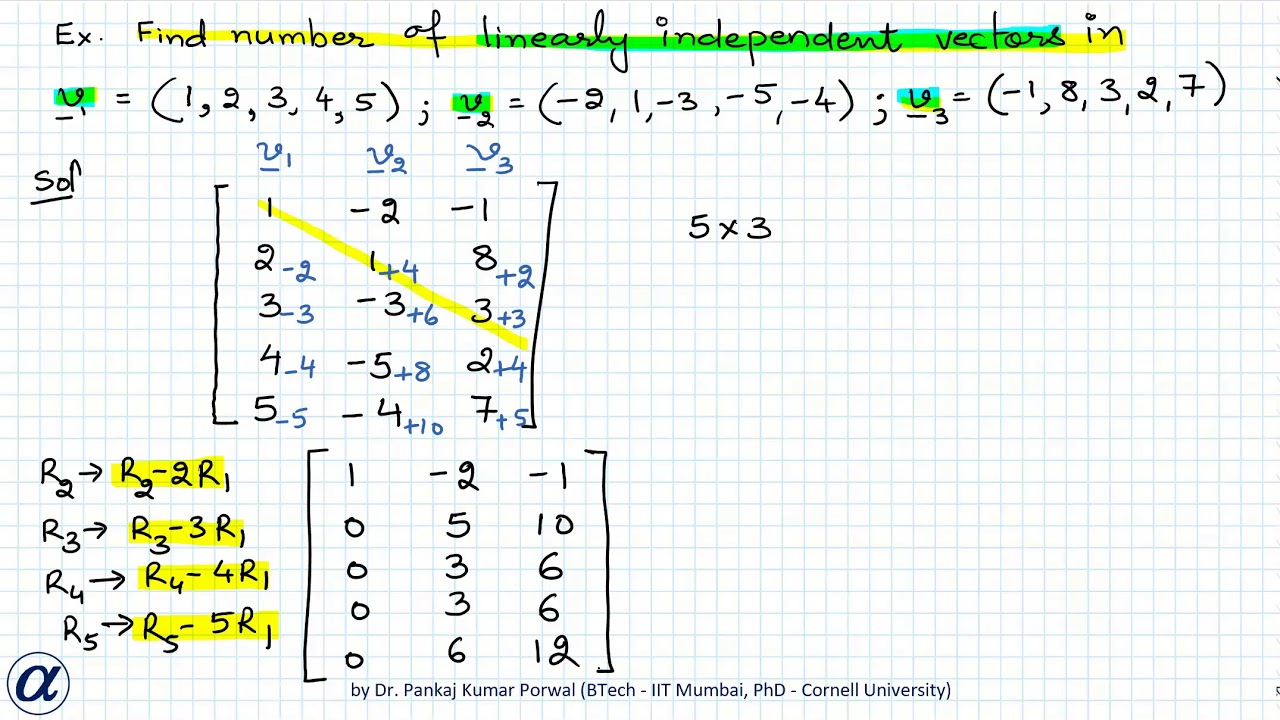
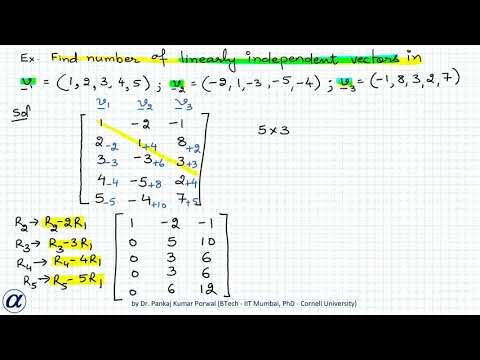
Q . Find number of linearly independent vectors in v1 = ( 1 , 2 , 3 , 4 , 5 ) ; v2 = ( -2 , 1 , -3 , -5 , -4 ) ; v3 = ( -1 , 8 , 3 , 2 , 7 )

In this video you will learn about linear independence of vectors. If any vector from given collection of vectors can be written as linear combination of remaining vectors then the vectors are said to be linearly dependent. If vectors are not linearly dependent then they are said to be linearly dependent.

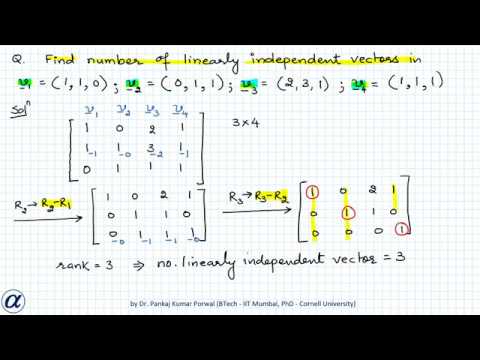
To find if given vectors are linearly independent (or to find number on linearly independent vectors in given vectors ) we are arrange all the given vectors in matrix form such that each vector forms a column of the matrix. Now rank of this matrix will be equal to number of linearly independent vectors in given vectors.

How to find if vectors are linearly independent?
Are these vectors linearly independent?
Are these vectors linearly dependent?
How to determine if the given vectors are linearly dependent or independent?
How to determine number on linearly independent vectors in given vectors?
What is matrix rank method to find number of linearly independent vectors in given vectors?
What is difference between linear dependence and linear independence of vectors?
What is linear combination of vectors?
What happens if all the scalar coefficients in linear combination are zero?
What happens if all the scalar coefficients in linear combination are not zero?

This video is uploaded by
Alpha Academy, Udaipur
Minakshi Porwal (9460189461)