filmov
tv
Linearly Dependent Vectors | Example of Linearly Dependent Vectors

Показать описание
Linearly Independent Vectors Test (Shortcut!!)
Subscribe
How to test the given vectors are linearly independent or not? The vectors v1,v2,v3,...vn in a vector space V are said to be linearly dependent if there exist constants c1,c2,c3,....cn not all zero such that:
c1v1+c2v2+c3v3+......+cnvn=0 -------------------------(i)
otherwise v1,v2,v3,.....vn are called linearly independent, that is v1,v2,v3,....vn are linearly independent if whenever c1v1+c2v2+c3v3+......+cnvn=0 , we must have c1=c2=c3=0.
That is the linear combination of v1,v2,v3,....vn yields the zero vector.
How to determine either the vectors are linearly independent or not?
There are two ways to check either the vectors are linearly independent or not.
1-Graphically
2-Linear Combination Equation
Graphical Approach:
This approach is helpful for all those vectors that lie in 2D: means those vectors that have two components. Another point to consider is there should be nor more than three vectors for better understanding.
Lets consider an example:
V1=[1 2]
v2=[2 4]
click here for reading the complete post:
Subscribe
How to test the given vectors are linearly independent or not? The vectors v1,v2,v3,...vn in a vector space V are said to be linearly dependent if there exist constants c1,c2,c3,....cn not all zero such that:
c1v1+c2v2+c3v3+......+cnvn=0 -------------------------(i)
otherwise v1,v2,v3,.....vn are called linearly independent, that is v1,v2,v3,....vn are linearly independent if whenever c1v1+c2v2+c3v3+......+cnvn=0 , we must have c1=c2=c3=0.
That is the linear combination of v1,v2,v3,....vn yields the zero vector.
How to determine either the vectors are linearly independent or not?
There are two ways to check either the vectors are linearly independent or not.
1-Graphically
2-Linear Combination Equation
Graphical Approach:
This approach is helpful for all those vectors that lie in 2D: means those vectors that have two components. Another point to consider is there should be nor more than three vectors for better understanding.
Lets consider an example:
V1=[1 2]
v2=[2 4]
click here for reading the complete post:
Linearly Dependent Vectors | Example of Linearly Dependent Vectors
Linear Independence
How to find out if a set of vectors are linearly independent? An example.
❖ Linear Independence and Linear Dependence, Ex 1 ❖
Introduction to linear independence | Vectors and spaces | Linear Algebra | Khan Academy
Linearly Dependent Vectors Example in Linear Algebra-Step by Step Explanation (2024)
06 Linearly Dependent Vectors Exercise
Linear Algebra Example Problems - Linearly Independent Vectors #1
Linear Algebra Example Problems - Linearly Independent Vectors #2
Linearly Independent Set
Span and linear independence example | Vectors and spaces | Linear Algebra | Khan Academy
Vector Subspace | Linearly Independent | Linearly Dependents Vectors | Linear Algebra
Vector Subspace | Linearly Independent | Linearly Dependents Vectors | Linear Algebra | Engineering|
More on linear independence | Vectors and spaces | Linear Algebra | Khan Academy
Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra
Example of Linear Independence Using Determinant
Determining Linear Independence vs Linear Dependence
7 easy tricks to check linearly dependent and independent vectors| definition and examples
Linear Algebra: check if three vectors are linearly independent
Part 2/3: Linearly Independent and Linearly Dependent Set of Vectors
Linear Algebra 6e: Linear Dependence Example 1 - Geometric Vectors
ShortTrick For Linear Dependent & Independent | Phaipedia #shorts #csirnet #gate #mathstricks
Vector Space | Linearly Independent & Dependent Vectors , Concept With Trick | Linear Algebra
Linear Algebra - 10 - EXAMPLE Find the Linearly Independent Set
Комментарии
 0:09:08
0:09:08
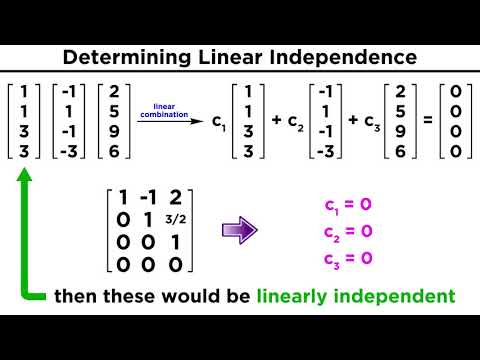 0:12:56
0:12:56
 0:04:41
0:04:41
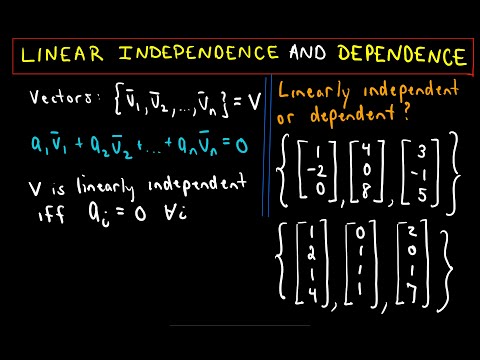 0:08:05
0:08:05
 0:15:46
0:15:46
 0:12:03
0:12:03
 0:04:04
0:04:04
 0:04:58
0:04:58
 0:05:38
0:05:38
 0:12:17
0:12:17
 0:16:53
0:16:53
 0:17:10
0:17:10
 0:09:19
0:09:19
 0:17:38
0:17:38
 0:09:59
0:09:59
 0:03:05
0:03:05
 0:06:39
0:06:39
 0:11:47
0:11:47
 0:03:44
0:03:44
 0:20:34
0:20:34
 0:06:27
0:06:27
 0:01:00
0:01:00
 0:12:56
0:12:56
 0:01:55
0:01:55