filmov
tv
Example of Linear Independence Using Determinant
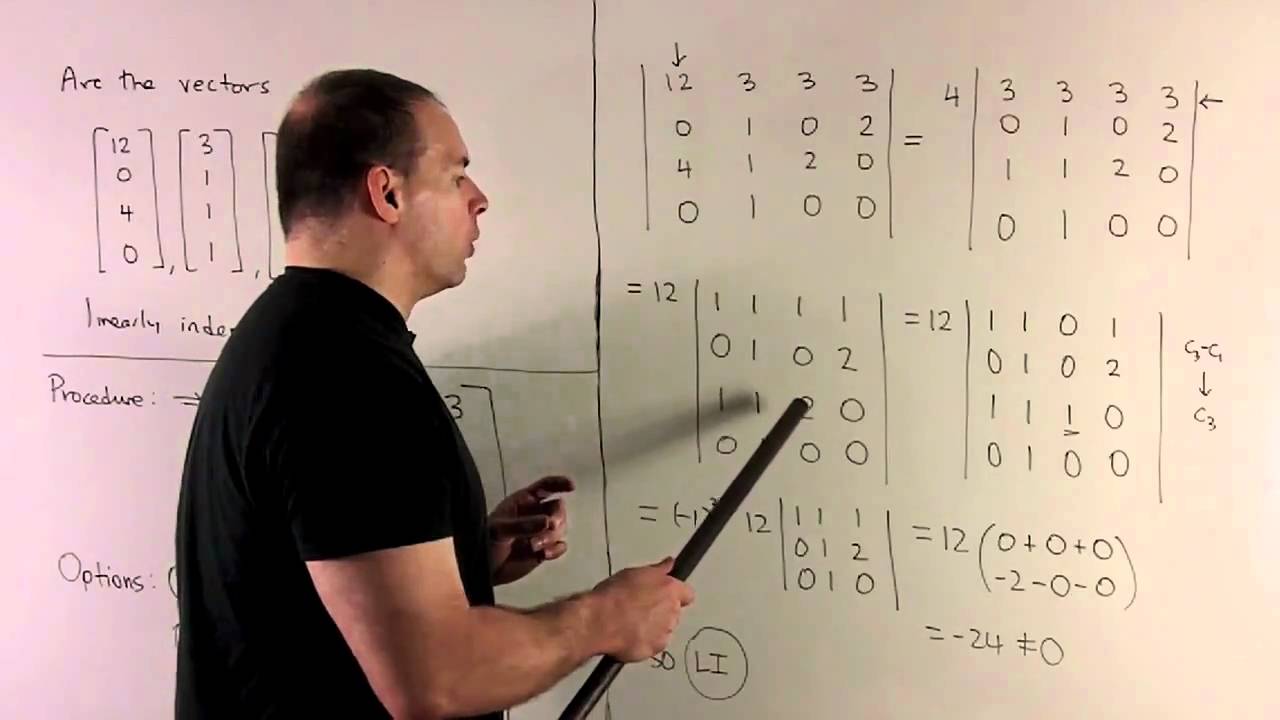
Показать описание
Linear Algebra: Let S = {[12, 0, 4, 0], [3,1 , 1, 1], [3, 0, 2, 0], [3, 2, 0, 0]}. Show that S is a linearly independent set by computing the determinant of the matrix whose columns are the vectors in S.
Example of Linear Independence Using Determinant
Linear Independence
How to find out if a set of vectors are linearly independent? An example.
❖ Linear Independence and Linear Dependence, Ex 1 ❖
Span and linear independence example | Vectors and spaces | Linear Algebra | Khan Academy
Introduction to linear independence | Vectors and spaces | Linear Algebra | Khan Academy
Linear independence proof example
Linear Independence of Functions & The Wronskian
Linearly Dependent Vectors | Example of Linearly Dependent Vectors
Linear Algebra - Lecture 15 - Linear Independence
Linear Algebra - 10 - EXAMPLE Find the Linearly Independent Set
Oxford Linear Algebra: Basis, Spanning and Linear Independence
Linear Algebra Example Problems - Linearly Independent Vectors #1
Linear Independence of Matrix Columns (Example)
The Vector Space of Polynomials: Span, Linear Independence, and Basis
Determine if the Functions are Linearly Independent or Linearly Dependent
More on linear independence | Vectors and spaces | Linear Algebra | Khan Academy
ShortTrick For Linear Dependent & Independent | Phaipedia #shorts #csirnet #gate #mathstricks
Wronskian Test for Linear Independence | Math with Janine
Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra
Chapter 04.02: Lesson: Are These Vectors Linearly Independent: Example 2
Linear Independence and Linear Dependence, Part 2 of 2
Ch6Pr43: Linear Independence of Polynomials
Linearly Independent Set
Комментарии
 0:03:05
0:03:05
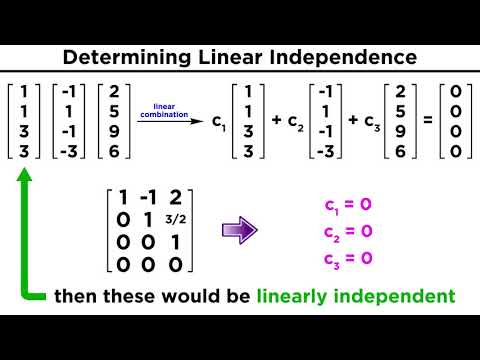 0:12:56
0:12:56
 0:04:41
0:04:41
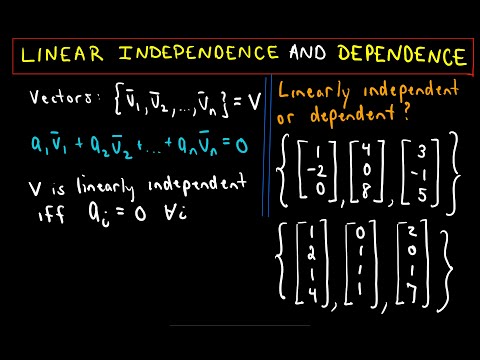 0:08:05
0:08:05
 0:16:53
0:16:53
 0:15:46
0:15:46
 0:03:33
0:03:33
 0:09:59
0:09:59
 0:09:08
0:09:08
 0:13:46
0:13:46
 0:01:55
0:01:55
 0:41:44
0:41:44
 0:04:58
0:04:58
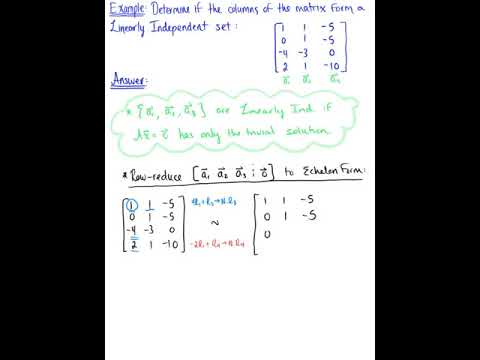 0:07:37
0:07:37
 0:12:50
0:12:50
 0:04:29
0:04:29
 0:17:38
0:17:38
 0:01:00
0:01:00
 0:06:46
0:06:46
 0:09:59
0:09:59
 0:09:13
0:09:13
 0:06:46
0:06:46
 0:08:31
0:08:31
 0:12:17
0:12:17